URL:https://pintia.cn/problem-sets/91827364500/exam/problems/91827370530
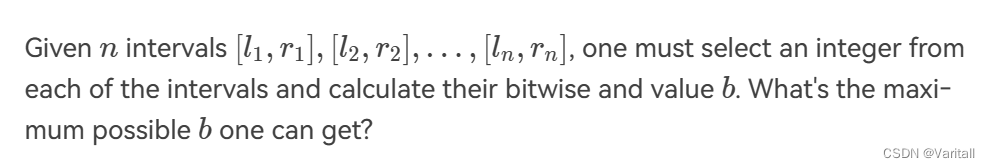
目录
Problem/题意
Thought/思路
Code/代码
Problem/题意
给出 N 个区间,可以从每个区间中选择一个数,问选出的 N 个数的按位与的值最大是多少?
Thought/思路
因为 100...00 一定比 011...11 大,所以我们可以从高位开始往低位考虑这个问题:
假设现在是第 i 位,那么是否能在所有区间中都找到一个数 Xi,它的第 i 位为 1
只要能找到这个数 Xi,那么就一定比 i 后面的低位全为带来的收益都要大。
那么我们如何找到这个数,并判断是否符合条件呢?
- (1)对于每个区间,找出从 Li 开始的,第一个在第 i 位为 1 的数 Xi;
- (2)若某个 Xi > Ri,则不合法,直接考虑下一个 i;
- (3)若所有的 Xi ≤ Ri,则将所有的 Li = Xi,这代表着,选中了高位 i 为 1 的情况下,低位 i 只能从更小的范围去选中 1。
Code/代码
#include "bits/stdc++.h"
#define int long long
int l[1000007], r[1000007];
int upperbound(int x, int i) {
if (((x >> i) & 1) == 0) {
x = (((x >> i) | 1) << i); // 往右移,把低位都置0,再移回来
}
return x;
}
void solve() {
int n; std::cin >> n;
for (int i = 1; i <= n; ++ i) std::cin >> l[i] >> r[i];
int ans = 0;
for (int i = 31; i >= 0; -- i) {
int flag = 1;
for (int j = 1; j <= n; ++ j) {
if (upperbound(l[j], i) > r[j]) {
flag = 0;
}
}
if (flag == 0) continue;
for (int j = 1; j <= n; ++ j) {
l[j] = upperbound(l[j], i);
}
ans = ans | (1 << i);
}
std::cout << ans << "\n";
}
signed main() {
std::ios::sync_with_stdio(false);
std::cin.tie(0); std::cout.tie(0);
int t; std::cin >> t;
while (t --) solve();
return 0;
}