主要记录算法和数据结构学习笔记，新的一年更上一层楼！

初级算法-栈与队列

一、栈实现队列
二、队列实现栈
三、有效的括号
四、删除字符串中的所有相邻重复项
五、逆波兰表达式求值
六、滑动窗口最大值
七、前K个高频元素

栈先进后出，不提供走访功能和迭代器
递归、表达式求值、括号匹配、进制转换一般利用栈

队列先进先出
满队列：front==(rear+1)%m
循环队列出队操作 front = (front+1) % (m+1)
循环队列，f 队头，r 队尾，队列中的元素个数为 (r-f+MAX+1) % MAX
循环队列队空：rear==front；队满：front == (rear+1) % MAX
链接方式存储队列，进行插入运算时常修改尾指针，插入前为空，头尾都需修改。
循环队列：尾指针-头指针+容量，front小于rear时N-front+rear

一、栈实现队列

1.题目：使用栈实现队列的下列操作：

push(x) – 将一个元素放入队列的尾部。
pop() – 从队列首部移除元素。
peek() – 返回队列首部的元素。
empty() – 返回队列是否为空。
示例

MyQueue queue = new MyQueue();
queue.push(1);
queue.push(2);
queue.peek();  // 返回 1
queue.pop();   // 返回 1
queue.empty(); // 返回 false

说明:
你只能使用标准的栈操作 – 也就是只有 push to top, peek/pop from top, size, 和 is empty 操作是合法的。
你所使用的语言也许不支持栈。你可以使用 list 或者 deque（双端队列）来模拟一个栈，只要是标准的栈操作即可。
假设所有操作都是有效的（例如，一个空的队列不会调用 pop 或者 peek 操作）。
2.解题思路：

// 使用两个数组的栈方法（push, pop） 实现队列
/**
* Initialize your data structure here.
*/
var MyQueue = function() {
   this.stackIn = [];
   this.stackOut = [];
};

/**
* Push element x to the back of queue. 
* @param {number} x
* @return {void}
*/
MyQueue.prototype.push = function(x) {
   this.stackIn.push(x);
};

/**
* Removes the element from in front of queue and returns that element.
* @return {number}
*/
MyQueue.prototype.pop = function() {
   const size = this.stackOut.length;
   if(size) {
       return this.stackOut.pop();
   }
   while(this.stackIn.length) {
       this.stackOut.push(this.stackIn.pop());
   }
   return this.stackOut.pop();
};

/**
* Get the front element.
* @return {number}
*/
MyQueue.prototype.peek = function() {
   const x = this.pop();
   this.stackOut.push(x);
   return x;
};

/**
* Returns whether the queue is empty.
* @return {boolean}
*/
MyQueue.prototype.empty = function() {
   return !this.stackIn.length && !this.stackOut.length
};
// 运行时间：60ms
// 内存消耗：40.8MB

二、队列实现栈

1.题目：使用队列实现栈的下列操作：

push(x) – 元素 x 入栈
pop() – 移除栈顶元素
top() – 获取栈顶元素
empty() – 返回栈是否为空

注意:
你只能使用队列的基本操作-- 也就是 push to back, peek/pop from front, size, 和 is empty 这些操作是合法的。
你所使用的语言也许不支持队列。你可以使用 list 或者 deque（双端队列）来模拟一个队列 , 只要是标准的队列操作即可。
你可以假设所有操作都是有效的（例如, 对一个空的栈不会调用 pop 或者 top 操作）。

2.解题思路：

// 使用两个队列实现
/**
 * Initialize your data structure here.
 */
var MyStack = function() {
    this.queue1 = [];
    this.queue2 = [];
};

/**
 * Push element x onto stack. 
 * @param {number} x
 * @return {void}
 */
MyStack.prototype.push = function(x) {
    this.queue1.push(x);
};

/**
 * Removes the element on top of the stack and returns that element.
 * @return {number}
 */
MyStack.prototype.pop = function() {
    // 减少两个队列交换的次数， 只有当queue1为空时，交换两个队列
    if(!this.queue1.length) {
        [this.queue1, this.queue2] = [this.queue2, this.queue1];
    }
    while(this.queue1.length > 1) {
        this.queue2.push(this.queue1.shift());
    }
    return this.queue1.shift();
};

/**
 * Get the top element.
 * @return {number}
 */
MyStack.prototype.top = function() {
    const x = this.pop();
    this.queue1.push(x);
    return x;
};

/**
 * Returns whether the stack is empty.
 * @return {boolean}
 */
MyStack.prototype.empty = function() {
    return !this.queue1.length && !this.queue2.length;
};

// 运行时间：64ms
// 内存消耗：40.9MB

// 使用一个队列实现
/**
 * Initialize your data structure here.
 */
var MyStack = function() {
    this.queue = [];
};

/**
 * Push element x onto stack. 
 * @param {number} x
 * @return {void}
 */
MyStack.prototype.push = function(x) {
    this.queue.push(x);
};

/**
 * Removes the element on top of the stack and returns that element.
 * @return {number}
 */
MyStack.prototype.pop = function() {
    let size = this.queue.length;
    while(size-- > 1) {
        this.queue.push(this.queue.shift());
    }
    return this.queue.shift();
};

/**
 * Get the top element.
 * @return {number}
 */
MyStack.prototype.top = function() {
    const x = this.pop();
    this.queue.push(x);
    return x;
};

/**
 * Returns whether the stack is empty.
 * @return {boolean}
 */
MyStack.prototype.empty = function() {
    return !this.queue.length;
};

// 运行时间：76ms
// 内存消耗：41.1MB

三、有效的括号

1.题目：
给定一个只包括 ‘(’，‘)’，‘{’，‘}’，‘[’，‘]’ 的字符串，判断字符串是否有效。

有效字符串需满足：

左括号必须用相同类型的右括号闭合。
左括号必须以正确的顺序闭合。
注意空字符串可被认为是有效字符串。

示例

示例 1:

输入: "()"
输出: true
示例 2:

输入: "()[]{}"
输出: true
示例 3:

输入: "(]"
输出: false
示例 4:

输入: "([)]"
输出: false
示例 5:

输入: "{[]}"
输出: true

2.解题思路：

var isValid = function (s) {
  const stack = [];
  for (let i = 0; i < s.length; i++) {
    let c = s[i];
    switch (c) {
      case '(':
        stack.push(')');
        break;
      case '[':
        stack.push(']');
        break;
      case '{':
        stack.push('}');
        break;
      default:
        if (c !== stack.pop()) {
          return false;
        }
    }
  }
  return stack.length === 0;
};
// 简化版本
var isValid = function(s) {
    const stack = [], 
        map = {
            "(":")",
            "{":"}",
            "[":"]"
        };
    for(const x of s) {
        if(x in map) {
            stack.push(x);
            continue;
        };
        if(map[stack.pop()] !== x) return false;
    }
    return !stack.length;
};
// 运行时间：52ms
// 内存消耗：41.6MB

四、删除字符串中的所有相邻重复项

1.题目：
给出由小写字母组成的字符串 S，重复项删除操作会选择两个相邻且相同的字母，并删除它们。

在 S 上反复执行重复项删除操作，直到无法继续删除。

在完成所有重复项删除操作后返回最终的字符串。答案保证唯一。

示例

输入："abbaca"
输出："ca"
解释：例如，在 "abbaca" 中，我们可以删除 "bb" 由于两字母相邻且相同，这是此时唯一可以执行删除操作的重复项。之后我们得到字符串 "aaca"，其中又只有 "aa" 可以执行重复项删除操作，所以最后的字符串为 "ca"。

提示：
1 <= S.length <= 20000
S 仅由小写英文字母组成。

2.解题思路：

// 使用栈
var removeDuplicates = function(s) {
    const stack = [];
    for(const x of s) {
        let c = null;
        if(stack.length && x === (c = stack.pop())) continue;
        c && stack.push(c);
        stack.push(x);
    }
    return stack.join("");
};
// 运行时间：76ms
// 内存消耗：51.1MB

// 原地解法（双指针模拟栈）
var removeDuplicates = function(s) {
    s = [...s];
    let top = -1; // 指向栈顶元素的下标
    for(let i = 0; i < s.length; i++) {
        if(top === -1 || s[top] !== s[i]) { // top === -1 即空栈
            s[++top] = s[i]; // 入栈
        } else {
            top--; // 推出栈
        }
    }
    s.length = top + 1; // 栈顶元素下标 + 1 为栈的长度
    return s.join('');
};
// 运行时间：64ms
// 内存消耗：46.8MB

五、逆波兰表达式求值

1.题目
根据逆波兰表示法，求表达式的值。

有效的运算符包括 + , - , * , / 。每个运算对象可以是整数，也可以是另一个逆波兰表达式。

说明：
整数除法只保留整数部分。给定逆波兰表达式总是有效的。换句话说，表达式总会得出有效数值且不存在除数为 0 的情况。

示例

示例 1：

输入: ["2", "1", "+", "3", " * "]
输出: 9
解释: 该算式转化为常见的中缀算术表达式为：((2 + 1) * 3) = 9
示例 2：

输入: ["4", "13", "5", "/", "+"]
输出: 6
解释: 该算式转化为常见的中缀算术表达式为：(4 + (13 / 5)) = 6
示例 3：

输入: ["10", "6", "9", "3", "+", "-11", " * ", "/", " * ", "17", "+", "5", "+"]

输出: 22

解释:该算式转化为常见的中缀算术表达式为：

((10 * (6 / ((9 + 3) * -11))) + 17) + 5       
= ((10 * (6 / (12 * -11))) + 17) + 5       
= ((10 * (6 / -132)) + 17) + 5     
= ((10 * 0) + 17) + 5     
= (0 + 17) + 5    
= 17 + 5    
= 22    
逆波兰表达式：是一种后缀表达式，所谓后缀就是指运算符写在后面。

平常使用的算式则是一种中缀表达式，如 ( 1 + 2 ) * ( 3 + 4 ) 。

该算式的逆波兰表达式写法为 ( ( 1 2 + ) ( 3 4 + ) * ) 。

逆波兰表达式主要有以下两个优点：

-去掉括号后表达式无歧义，上式即便写成 1 2 + 3 4 + * 也可以依据次序计算出正确结果。

-适合用栈操作运算：遇到数字则入栈；遇到运算符则取出栈顶两个数字进行计算，并将结果压入栈中。

2.解题思路：

var evalRPN = function (tokens) {
    const stack = [];
    for (const token of tokens) {
        if (isNaN(Number(token))) { // 非数字
            const n2 = stack.pop(); // 出栈两个数字
            const n1 = stack.pop();
            switch (token) { // 判断运算符类型，算出新数入栈
                case "+":
                    stack.push(n1 + n2);
                    break;
                case "-":
                    stack.push(n1 - n2);
                    break;
                case "*":
                    stack.push(n1 * n2);
                    break;
                case "/":
                    stack.push(n1 / n2 | 0);
                    break;
            }
        } else { // 数字
            stack.push(Number(token));
        }
    }
    return stack[0]; // 因没有遇到运算符而待在栈中的结果
};
// 运行时间：64ms
// 内存消耗：43.8MB

六、滑动窗口最大值

1.题目：给定一个数组 nums，有一个大小为 k 的滑动窗口从数组的最左侧移动到数组的最右侧。你只可以看到在滑动窗口内的 k 个数字。滑动窗口每次只向右移动一位。

返回滑动窗口中的最大值。

进阶：

你能在线性时间复杂度内解决此题吗？

示例
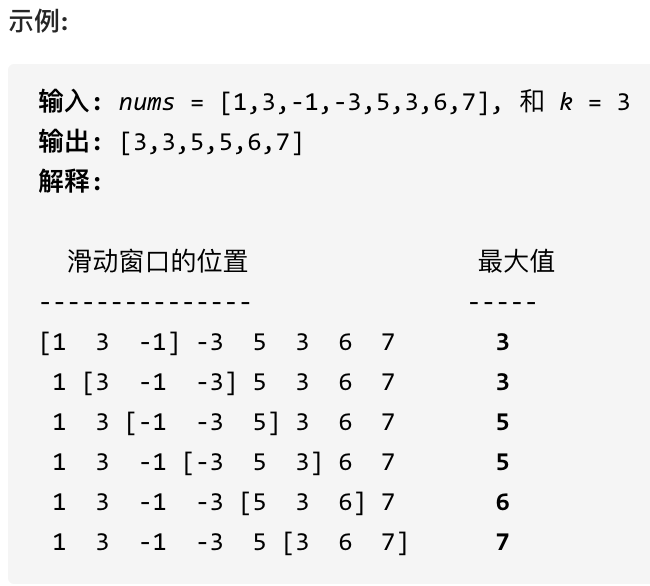
在这里插入图片描述
提示：
1 <= nums.length <= 10⁵
-10⁴ <= nums[i] <= 10⁴
1 <= k <= nums.length

2.解题思路：

/**
 * @param {number[]} nums
 * @param {number} k
 * @return {number[]}
 */
var maxSlidingWindow = function (nums, k) {
    class MonoQueue {
        queue;
        constructor() {
            this.queue = [];
        }
        enqueue(value) {
            let back = this.queue[this.queue.length - 1];
            while (back !== undefined && back < value) {
                this.queue.pop();
                back = this.queue[this.queue.length - 1];
            }
            this.queue.push(value);
        }
        dequeue(value) {
            let front = this.front();
            if (front === value) {
                this.queue.shift();
            }
        }
        front() {
            return this.queue[0];
        }
    }
    let helperQueue = new MonoQueue();
    let i = 0, j = 0;
    let resArr = [];
    while (j < k) {
        helperQueue.enqueue(nums[j++]);
    }
    resArr.push(helperQueue.front());
    while (j < nums.length) {
        helperQueue.enqueue(nums[j]);
        helperQueue.dequeue(nums[i]);
        resArr.push(helperQueue.front());
        i++, j++;
    }
    return resArr;
};
#运行时间：8528ms
#内存消耗：75.3MB

七、前K个高频元素

1.题目：
给定一个非空的整数数组，返回其中出现频率前 k 高的元素。
示例

示例 1:

输入: nums = [1,1,1,2,2,3], k = 2
输出: [1,2]
示例 2:

输入: nums = [1], k = 1
输出: [1]

提示：
你可以假设给定的 k 总是合理的，且 1 ≤ k ≤ 数组中不相同的元素的个数。
你的算法的时间复杂度必须优于 $\log n)$ , n 是数组的大小。
题目数据保证答案唯一，换句话说，数组中前 k 个高频元素的集合是唯一的。
你可以按任意顺序返回答案。

// js 没有堆 需要自己构造
class Heap {
    constructor(compareFn) {
        this.compareFn = compareFn;
        this.queue = [];
    }

    // 添加
    push(item) {
        // 推入元素
        this.queue.push(item);

        // 上浮
        let index = this.size() - 1; // 记录推入元素下标
        let parent = Math.floor((index - 1) / 2); // 记录父节点下标

        while (parent >= 0 && this.compare(parent, index) > 0) { // 注意compare参数顺序
            [this.queue[index], this.queue[parent]] = [this.queue[parent], this.queue[index]];

            // 更新下标
            index = parent;
            parent = Math.floor((index - 1) / 2);
        }
    }

    // 获取堆顶元素并移除
    pop() {
        // 堆顶元素
        const out = this.queue[0];

        // 移除堆顶元素 填入最后一个元素
        this.queue[0] = this.queue.pop();

        // 下沉
        let index = 0; // 记录下沉元素下标
        let left = 1; // left 是左子节点下标 left + 1 则是右子节点下标
        let searchChild = this.compare(left, left + 1) > 0 ? left + 1 : left;

        while (searchChild !== undefined && this.compare(index, searchChild) > 0) { // 注意compare参数顺序
            [this.queue[index], this.queue[searchChild]] = [this.queue[searchChild], this.queue[index]];

            // 更新下标
            index = searchChild;
            left = 2 * index + 1;
            searchChild = this.compare(left, left + 1) > 0 ? left + 1 : left;
        }

        return out;
    }

    size() {
        return this.queue.length;
    }

    // 使用传入的 compareFn 比较两个位置的元素
    compare(index1, index2) {
        // 处理下标越界问题
        if (this.queue[index1] === undefined) return 1;
        if (this.queue[index2] === undefined) return -1;

        return this.compareFn(this.queue[index1], this.queue[index2]);
    }

}

const topKFrequent = function (nums, k) {
    const map = new Map();

    for (const num of nums) {
        map.set(num, (map.get(num) || 0) + 1);
    }

    // 创建小顶堆
    const heap= new Heap((a, b) => a[1] - b[1]);

    // entry 是一个长度为2的数组，0位置存储key，1位置存储value
    for (const entry of map.entries()) {
        heap.push(entry);

        if (heap.size() > k) {
            heap.pop();
        }
    }

    // return heap.queue.map(e => e[0]);

    const res = [];

    for (let i = heap.size() - 1; i >= 0; i--) {
        res[i] = heap.pop()[0];
    }

    return res;
};
#运行时间：88ms
#内存消耗：44.4MB