解法：

1.先序遍历创建二叉树链表形式

2.求二叉树的深度

用后序遍历实现：

1.后序遍历求节点A左右子树高度

2.对节点A：

        1.取左右子树较大高度

        2.返回高度+1（即以节点A为根节点的子树的最大深度）

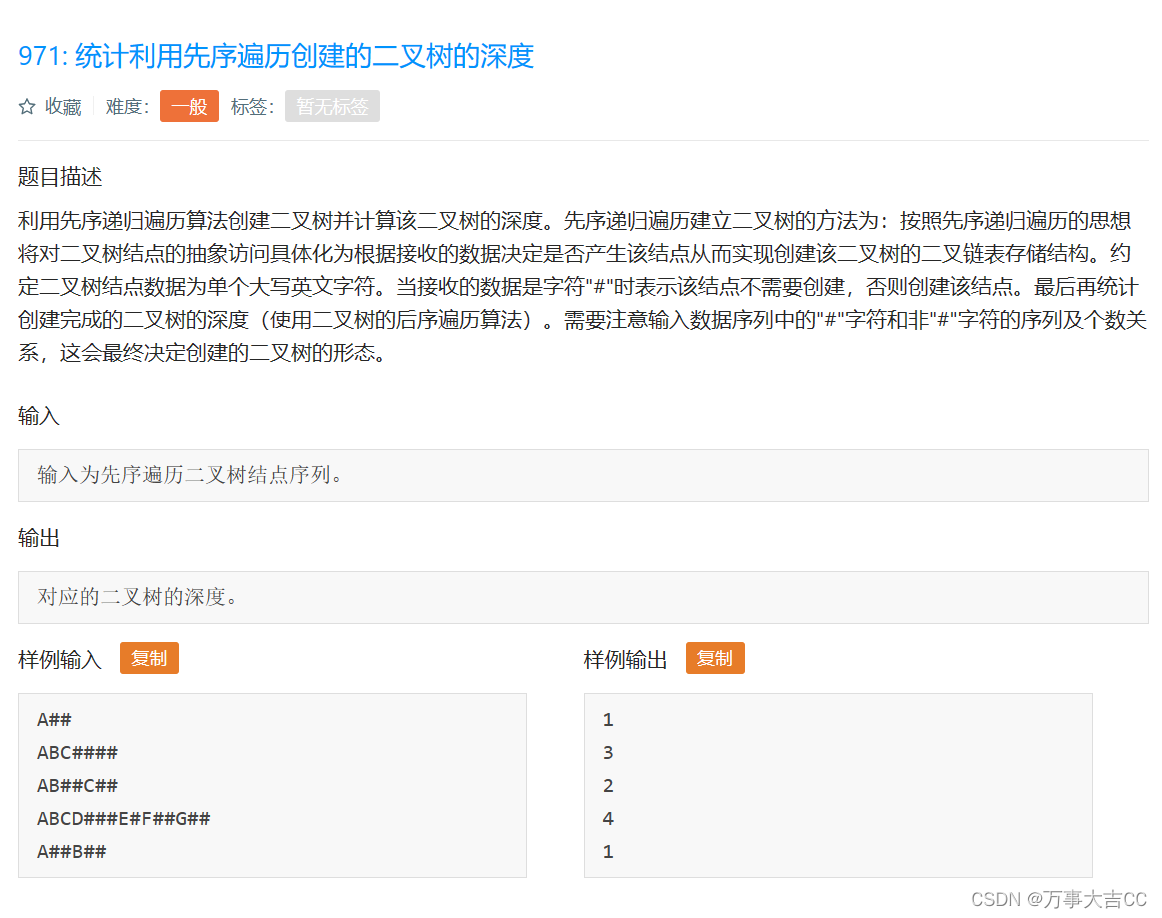
例如

#include <iostream>
using namespace std;
// 定义二叉树结点
struct TreeNode {
    char val;
    TreeNode* left;
    TreeNode* right;
    TreeNode(char x) :val(x), left(NULL), right(NULL) {};
};
// 先序递归遍历建立二叉树
TreeNode* buildTree() {
    char c;
    cin >> c;
    if (c == '#') {
        return NULL;
    }
    TreeNode* root = new TreeNode(c);
    root->left = buildTree();
    root->right = buildTree();
    return root;
}
// 后序遍历计算二叉树深度
int getDepth(TreeNode* root) {
    if (root == NULL) {
        return 0;
    }
    else {
        int leftDepth = getDepth(root->left);
        int rightDepth = getDepth(root->right);
        return max(leftDepth, rightDepth) + 1;
    }
}
int main() {
    TreeNode* root= buildTree();
    cout<<getDepth(root);
    return 0;
}

由于树的最大深度=树的高度=层数

可以用层序遍历

#include<iostream>
#include<queue>
using namespace std;
// 定义二叉树结点
struct TreeNode {
    char val;
    TreeNode* left;
    TreeNode* right;
    TreeNode(char x) :val(x), left(NULL), right(NULL) {};
};
// 先序递归遍历建立二叉树
TreeNode* buildTree() {
    char c;
    cin >> c;
    if (c == '#') {
        return NULL;
    }
    TreeNode* root = new TreeNode(c);
    root->left = buildTree();
    root->right = buildTree();
    return root;
}
// 计算二叉树深度
int getDepth(TreeNode* root) {
    int result = 0;
    queue<TreeNode*> q;
    q.push(root);
    while (!q.empty()) {
        result++;
        for (int i = 0; i < q.size(); i++) {
            TreeNode* cur = q.front();
            q.pop();
            if (cur->left) q.push(cur->left);
            if (cur->right) q.push(cur->right);
        }
    }
    return result;
}
int main() {
    TreeNode* root= buildTree();
    cout<<getDepth(root);
    return 0;
}