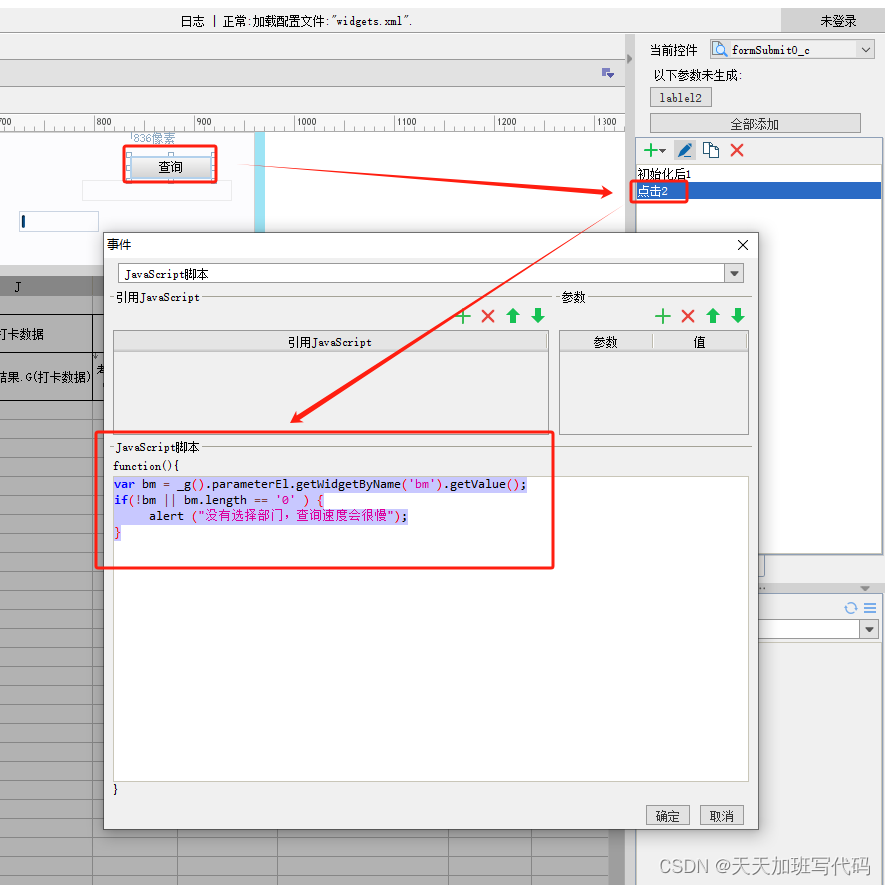
图的性质
聚类系数
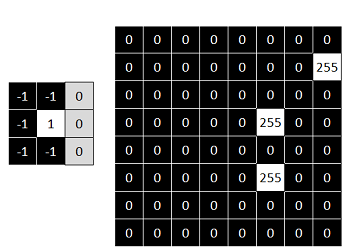
C i = E i T i C_i = \frac{E_i}{T_i} Ci=TiEi
E i E_i Ei表示节点 i i i的邻居实际存在的边的数量, T i T_i Ti表示节点 i i i的邻居可能(最多)存在的边的数量
理论溯源
聚类系数这一概念首先源于论文“Collective dynamics of ‘small-world’ networks”,主要介绍了“小世界网络”现象以及它在各种自组织系统中的应用。作者通过对网络结构的调整,发现这样的网络可以同时具有高度聚集性和短特征路径长度的特征,而这种特点在传统的规则网络或随机网络中不易出现。
小世界网络:通常将网络拓扑连接假定为完全规则或完全随机,但现实世界中的很多网络连接介于这两个极端之间,作者探索了调整这个中间度,得到的简单网络模型,这些网络具有高度聚类特征、也具有特征路径长度较小的特征。
以下是原文中关于聚类系数的定义
Suppose that a vertex v has k v k_v kv neighbours;then at most k v ( k v − 1 ) k_v(k_v-1) kv(kv−1) edges can exist between them (this occurs when every neighbour of v is connected to every other neighbour of v).
Let C v C_v Cv denote the fraction of these allowable edges that actually exist
C v C_v Cv reflects the extent to which friends of v are also friends of each other; and thus C measures the cliquishness(小圈子) of a typical friendship circle
其含义是,在某一个节点一跳领域内封闭三角形的比例【图片来源-参考文章1】

参考文章
图论中的聚类系数(Clustering coefficient)简单介绍