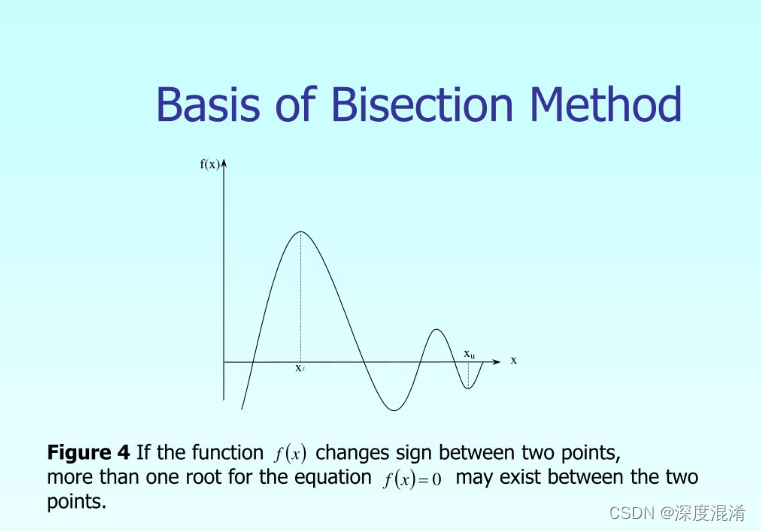
1 二分法

二分法是一种分治算法，是一种数学思维。

对于区间[a，b]上连续不断且f（a）·f（b）<0的函数y=f（x），通过不断地把函数f（x）的零点所在的区间一分为二，使区间的两个端点逐步逼近零点，进而得到零点近似值的方法叫二分法。
 

基本思路：

给定精确度ξ,用二分法求函数f(x)零点近似值的步骤如下:
1 确定区间[a,b],验证f(a)·f(b)<0,给定精确度ξ.
2 求区间(a,b)的中点c.
3 计算f(c).
(1) 若f(c)=0,则c就是函数的零点;
(2) 若f(a)·f(c)<0,则令b=c;
(3) 若f(c)·f(b)<0,则令a=c.
(4) 判断是否达到精确度ξ:即若|a-b|<ξ,则得到零点近似值a(或b),否则重复2-4.
 

2 代码

调用之前，请用委托方法给定需要求解的方程。

这样就可以求解任意的方程而无需修改核心代码。

using System;
using System.Text;
using System.Collections;
using System.Collections.Generic;

namespace Legalsoft.Truffer.Algorithm
{
	public delegate double delegateFunctionX(double x);

	public static partial class Algorithm_Gallery
	{
		public static delegateFunctionX funx = null;

		/// <summary>
		/// 二分法解方程的算法
		/// </summary>
		/// <param name="a"></param>
		/// <param name="b"></param>
		/// <param name="epsilon"></param>
		/// <returns></returns>
		public static double Bisection(double a, double b, double epsilon = 0.01)
		{
			if (funx(a) * funx(b) >= 0)
			{
				return 0.0;
			}

			double c = a;
			while ((b - a) >= epsilon)
			{
				c = (a + b) / 2;

				if (funx(c) == 0.0)
				{
					break;
				}
				else if (funx(c) * funx(a) < 0)
				{
					b = c;
				}
				else
				{
					a = c;
				}
			}
			return c;
		}
	}
}

POWER BY TRUFFER.CN
BY 315SOFT.COM