目录

F - Hop Sugoroku

题目大意：

思路解析：

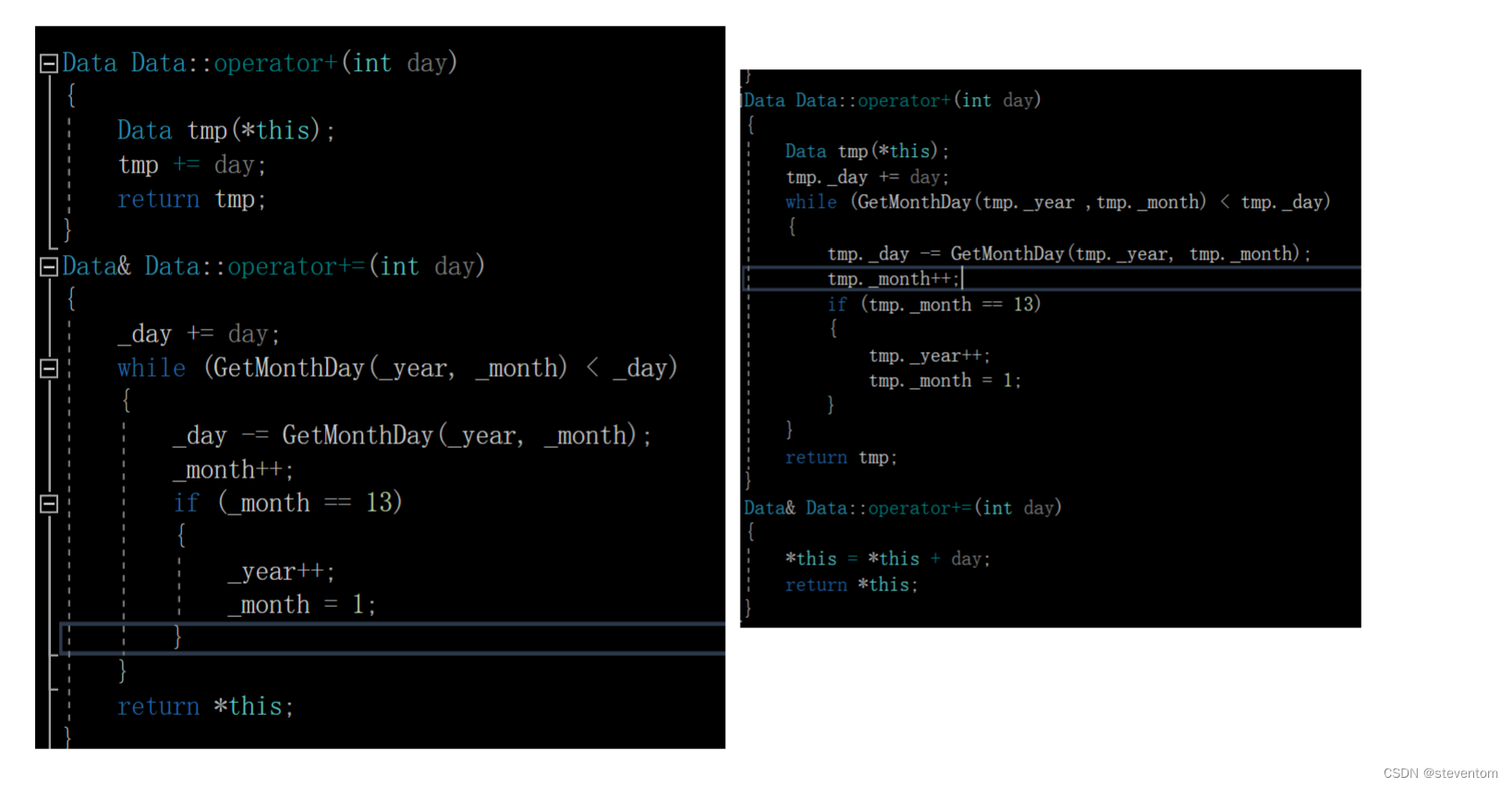
代码实现：

        

---

F - Hop Sugoroku

题目大意：

        

思路解析：

        容易想到这是一个dp题，然后初始转移方程为：

         如果当a[i] 较大时，时间复杂度为 O(N*N/a[i]) 接近于N，但是当a[i]为1时，时间复杂度解决O(N^2)，这是不能接受的。

观察dp转移方程可以发现 （j*a[i]+i）% a[i] == i % a[i]，即只有当 j % a == i % a时才能转移，这衍生出另一个dp方程 dp2[i][j]，表示当前格子在t，a[t] == i, t % a[t] == j。

根据上面分析可以发现dp[i] += dp2[j][i%j]，如果dp2开很大的话会爆内存空间，所以我们应该想到，当a[i] 较小时( 我取的是500) 采用dp2进行转移，如果a[i]较大则采用第一种方式进行转移。

这样的会时间开销就变为 O(N*N/500) 约等于 O(N*400)  

代码实现：

        

import java.io.*;
import java.math.BigInteger;
import java.util.*;


public class Main {
    static int mod1 = (int) 1e9 + 7;
    static int mod2 = 998244353;
    static int BD = 500;

    public static void main(String[] args) throws IOException {
        int n = input.nextInt();
        int[] dp = new int[n];
        dp[0] = 1;
        int ans = 0;
        int[][] dp2 = new int[505][505];
        for (int i = 0; i < n; i++) {
            int a = input.nextInt();
            for (int j = 1; j <= BD; j++) {
                dp[i] = (dp[i] + dp2[j][i % j]) % mod2;
            }
            if (a <= BD){
                dp2[a][i % a] = (dp2[a][i%a] + dp[i]) % mod2;
            }else {
                for (int j = 1; j * a + i < n; j++) {
                    dp[j * a + i] = (dp[j * a + i] + dp[i]) % mod2;
                }
            }
        }
        for (int i = 0; i < n; i++) {
            ans = (ans + dp[i]) % mod2;
        }
        out.println(ans);
        out.flush();
        out.close();
        br.close();
    }

    // -------------------------------- 模板 ---------------------------
    static boolean nextPermutation(int[] arr) {  // 排列数循环模板    记得使用 do while 循环
        int len = arr.length;
        int left = len - 2;
        while (left >= 0 && arr[left] >= arr[left + 1]) left--; // 从升序 一直往降序排列。
        if (left < 0) return false;
        int right = len - 1;
        // 找到第一个升序的位置，将其改为降序。
        while (arr[left] >= arr[right]) right--;
        {
            int t = arr[left];
            arr[left] = arr[right];
            arr[right] = t;
        }
        // 修改后它的前面仍然为降序，将前面全部修改为升序，这样能保证不会漏算，也不会算重
        left++;
        right = len - 1;
        while (left < right) {
            {
                int t = arr[left];
                arr[left] = arr[right];
                arr[right] = t;
            }
            left++;
            right--;
        }
//        System.out.println(Arrays.toString(arr));
        return true;
    }

    public static long qkm(long a, long b, long mod) { // 快速幂模板
        long res = 1;
        while (b > 0) {
            if ((b & 1) == 1) res = (res * a) % mod;
            a = (a * a) % mod;
            b >>= 1;
        }
        return res;
    }

//    // 线段树模板
//    public static int lowbit(int x){
//        return x & (-x);
//    }
//
//    public static void bulid(int n){
//        for (int i = 1; i <= n; i++) {
//            t[i] = a[i];
//            int j = i + lowbit(i);
//            if (j<=n) t[j] += t[i];
//        }
//    }
//
//    public static void updata(int x, int val){
//        while (x <= n){
//            t[x] += val;
//            x += lowbit(x);
//        }
//    }
//
//    public static int query(int l, int r){
//        int res = 0;
//        while (l <= r){
//            res += a[r];
//            r--;
//            while (r - lowbit(r) >= l){
//                res += t[r];
//                r -= lowbit(r);
//            }
//        }
//        return res;
//    }


    static PrintWriter out = new PrintWriter(new OutputStreamWriter(System.out));
    static Input input = new Input(System.in);
    static BufferedReader br = new BufferedReader(new InputStreamReader(System.in));

    static class Input {
        public BufferedReader reader;
        public StringTokenizer tokenizer;

        public Input(InputStream stream) {
            reader = new BufferedReader(new InputStreamReader(stream), 32768);
            tokenizer = null;
        }

        public String next() {
            while (tokenizer == null || !tokenizer.hasMoreTokens()) {
                try {
                    tokenizer = new StringTokenizer(reader.readLine());
                } catch (IOException e) {
                    throw new RuntimeException(e);
                }
            }
            return tokenizer.nextToken();
        }

        public String nextLine() {
            String str = null;
            try {
                str = reader.readLine();
            } catch (IOException e) {
                // TODO 自动生成的 catch 块
                e.printStackTrace();
            }
            return str;
        }

        public int nextInt() {
            return Integer.parseInt(next());
        }

        public long nextLong() {
            return Long.parseLong(next());
        }

        public Double nextDouble() {
            return Double.parseDouble(next());
        }

        public BigInteger nextBigInteger() {
            return new BigInteger(next());
        }
    }

}