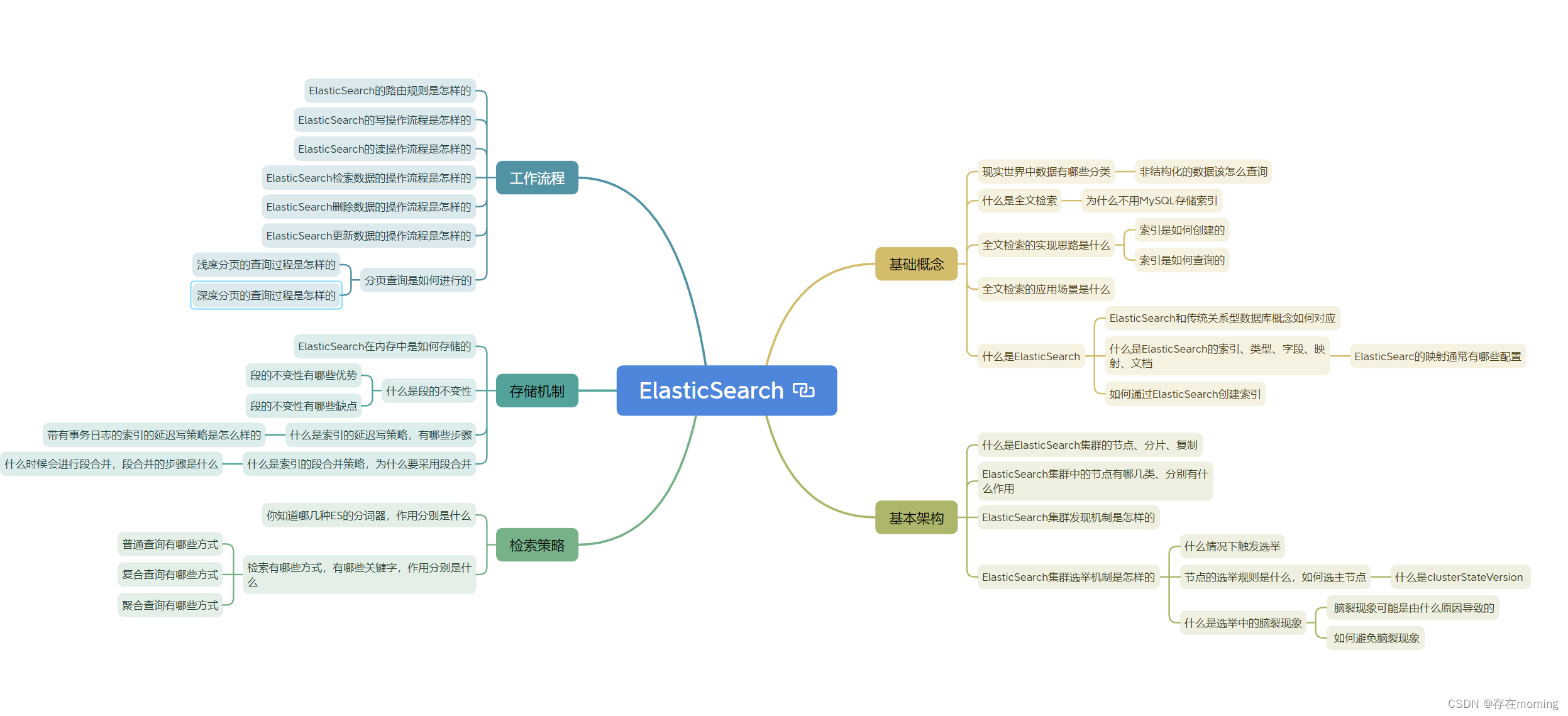
目录

1.单调栈

例题：【模板】单调栈

例题:求和  

2.单调队列

 例题：滑动窗口

---

1.单调栈

例题：【模板】单调栈

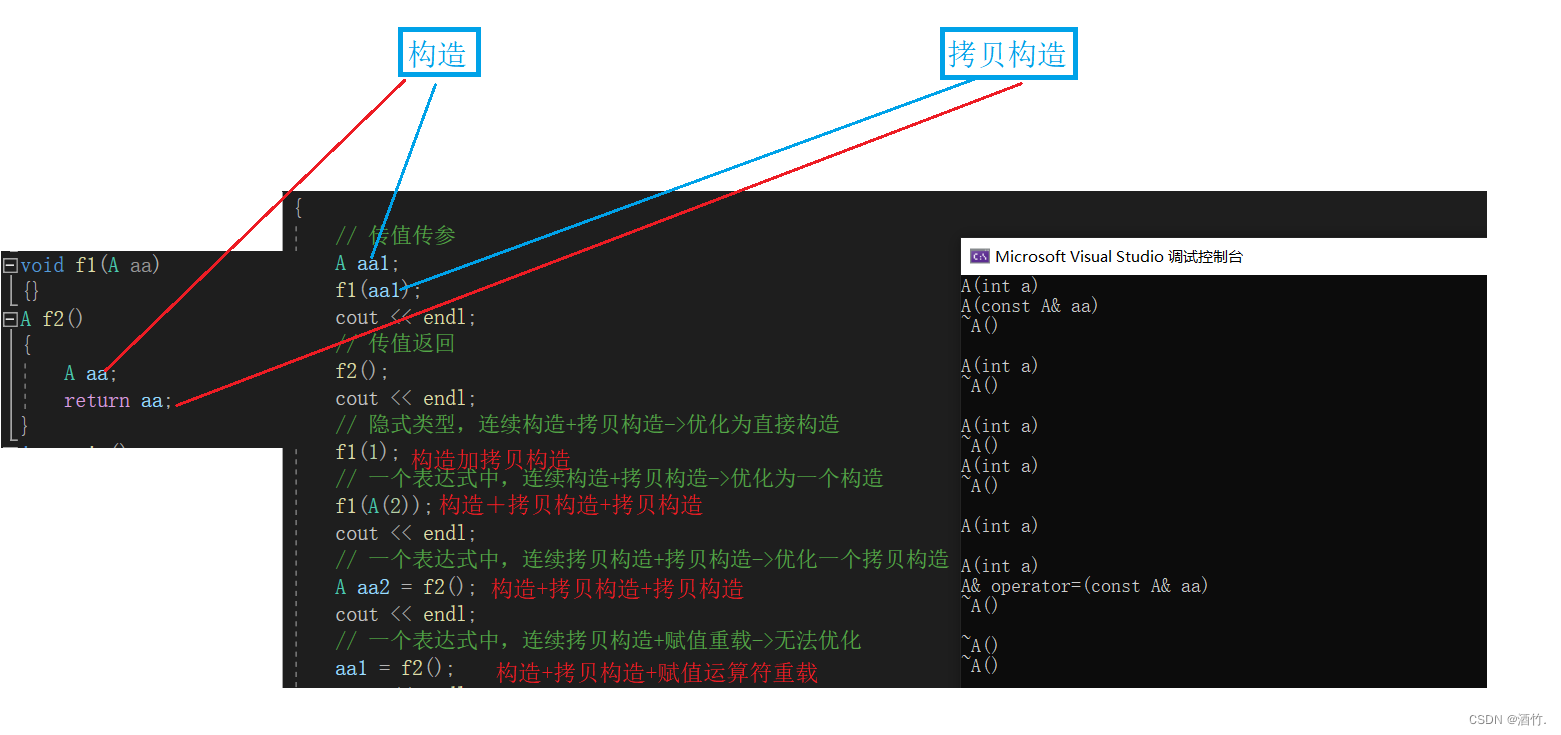
可以想象出一个柱状图，值越大，这个柱子越高

以此题的样例为例：

第一个数为7，想象一个高度为7的柱子，它的左边没有任何数，所以直接输出-1

然后第二个数为8，想象高度为8的柱子从右往左靠近7，因为7比8小，所以输出7

第三个数为5，5从右往左靠近8，而5比8小，所以8被删除，7大于5，所以7也被删除

此时5左边就没数了，输出-1

第四个数为6大于5，所以输出5

第五个数为7大于6，所以输出6

用栈的方法 

#include<bits/stdc++.h>
using namespace std;
using ll = long long;
const int N = 2e5 + 10;

int a[N],l[N];

int main(){
	ios::sync_with_stdio(0),cin.tie(0),cout.tie(0);
	stack<int> stk;
	int n;
	cin >> n;
	
	for(int i = 1; i <= n; i++) cin >> a[i];
	
	for(int i = 1; i <= n; i++)
	{
		while(stk.size() && stk.top() >= a[i]) stk.pop();
		
		if(stk.empty()) l[i] = -1;
		else l[i] = stk.top();
		
		stk.push(a[i]);
	}
	
	for(int i = 1; i <= n; i++)
		cout << l[i] << ' ';
	return 0;
}

例题:求和  

这题要对一个数的左边和右边都进行单调栈求比它小的值

#include<bits/stdc++.h>
using namespace std;
using ll = long long;
const int N = 1e5 + 10;

ll a[N],l[N],r[N];
int stk[N];
int top;//表示栈中元素个数

int main(){
	ios::sync_with_stdio(0),cin.tie(0),cout.tie(0);
	int n;
	cin >> n;
	for(int i = 1; i <= n; i++) cin >> a[i];
	
	for(int i = 1; i <= n; i++)
	{
		while(top && a[i] <= a[stk[top]]) top--;
		
		if(top != 0) l[i] = stk[top];
		else l[i] = 0;
		
		stk[++top] = i;
	}
	
	top = 0;//清空栈
	
	for(int i = n; i >= 1; i--)
	{
		while(top && a[i] < a[stk[top]]) top--;
		
		if(top != 0) r[i] = stk[top];
		else r[i] = n + 1;
		
		stk[++top] = i;
	}
	
	ll ans = 0;
	for(int i = 1; i <= n; i++) ans += a[i] * (r[i] - i) * (i - l[i]);
	cout << ans;
	return 0;
}

2.单调队列

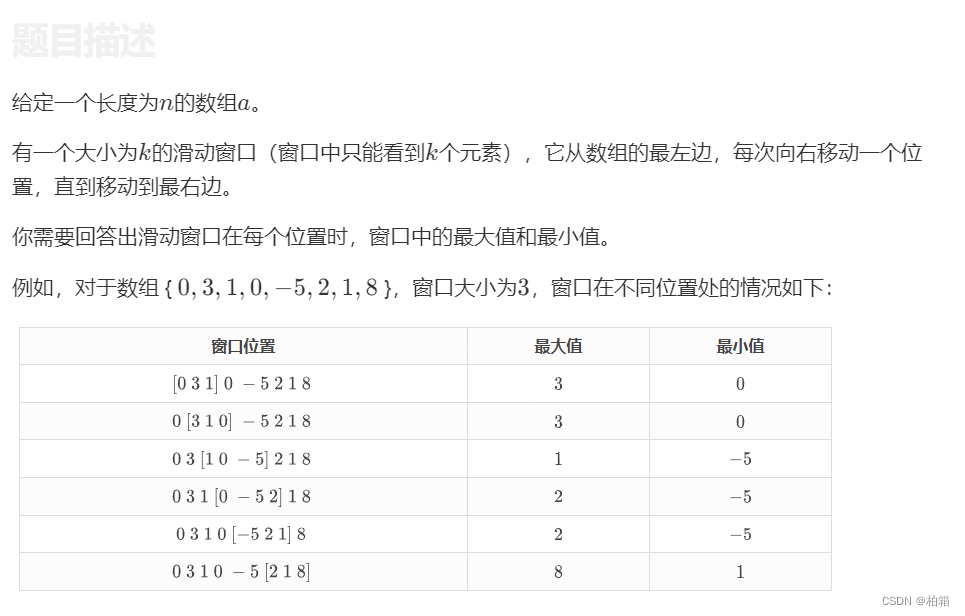
 例题：滑动窗口

以求窗口内最大值为例，想象一个双端队列，从右边入队，每次入队与原来的队列中的最右边的元素比较大小，如果它的数值更大，那么就从右边pop掉原来队列中最右端的那个数，直到最左边的元素最大为止

#include<bits/stdc++.h>
using namespace std;
using ll = long long;
const int N = 2e5 + 10;

int a[N];

int main(){
	ios::sync_with_stdio(0),cin.tie(0),cout.tie(0);
	int n,k;
	cin >> n >> k;
	
	for(int i = 1; i <= n; i++) cin >> a[i];
	deque<int> dq;
	
	//求最大值
	for(int i = 1; i <= n; i++)
	{
		while(dq.size() && k <= i - dq.front()) dq.pop_front();
		
		while(dq.size() && a[dq.back()] <= a[i]) dq.pop_back();
		
		dq.push_back(i);
		
		if(i >= k) cout << a[dq.front()] << ' ';
	}
	
	dq.clear();
	cout << endl;
	
	//求最小值
	for(int i = 1; i <= n; i++)
	{
		//区间合法
		while(dq.size() && k <= i - dq.front()) dq.pop_front();
		
		while(dq.size() && a[dq.back()] >= a[i]) dq.pop_back();
		
		dq.push_back(i);
		
		if(i >= k)cout << a[dq.front()] << ' ';
	}
	return 0;
}