文章目录
- 1、算法复杂度
- 1.1 时间复杂度
- 1.2 空间复杂度
- 2、数组
- 3、leetcode485:最大连续1的个数
- 4、leetcode283:移动0
- 5、leetcode27:移除元素
1、算法复杂度
1.1 时间复杂度
算法的执行时间与输入值之间的关系(看代码实际总行数的变化) ⇒ 常对幂指阶
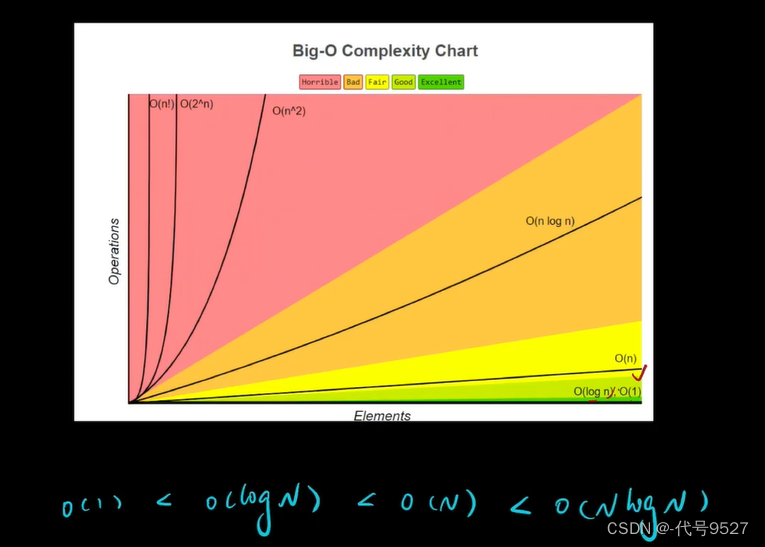
1.2 空间复杂度
算法存储空间与输入值之间的关系(看变量的数量或者对象的数量)

2、数组
数组特点:
- 所占的内存空间连续
- 存储的元素类型相同
访问、搜索、插入、删除的时间复杂度:
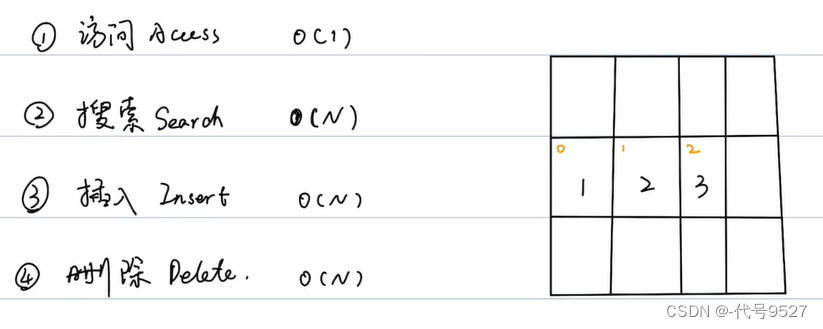
访问时,时间复杂度为O(1)
a[i] = a[0]地址 + i * 元素类型所占字节
搜索时,需要遍历,直到找到a[i]的值等于所查的value,复杂度为O(n),插入和删除时,最坏的情况在数组的开头进行增删,后面的元素都得后退或者前移一格,或者说插入引起了数组的copy到扩容后的新数组,则也是O(n)

3、leetcode485:最大连续1的个数

思路:遍历数组,出现一个1就把计数 +1,出现一个0则计数置为0,置为0之前存一下当前的计数值
public class P485 {
public static void main(String[] args) {
int[] array = {1, 0, 1, 1, 0, 1, 1, 1, 0, 1};
System.out.println(column(array));
}
public static int column(int[] array) {
if (null == array || array.length == 0) {
return 0;
}
int result = 0;
// 被出现的0打断计数后,存下目前的最大计数值
int resultTemp = 0;
for (int i : array) {
if (i == 1) {
result = result + 1;
}
if (i == 0) {
resultTemp = Math.max(result, resultTemp);
// 置为0,重新计数
result = 0;
}
}
return Math.max(result, resultTemp);
}
}
测试:
4、leetcode283:移动0

一开始想的是:遍历找为0的元素,找到一个就将其后面的所有元素往前移动一位,然后将这个为0的元素自身扔在数组末尾。操作次数太多。
转向考虑找非0的,找到第一个,就将其放到index = 0 的位置,并且index计数加一,准备等下一个非0的元素,依次往后放非0元素,遍历完后,index后面剩下的空位都补0即可。
public class P283 {
public static void main(String[] args) {
int[] array = {1, 0, 9, 0, 6, 8, 0};
for (int i : move(array)) {
System.out.print(i + ",");
}
}
public static int[] move(int[] array) {
// 记录非0元素下标位置(像一个指针,告诉我目前非0元素放到几号空位了)
int index = 0;
for (int i = 0; i < array.length; i++) {
if (array[i] != 0) {
// 非0元素前移
array[index] = array[i];
index++;
}
}
// 剩余空位全部为0
for (; index < array.length ; index++) {
array[index] = 0;
}
return array;
}
}
5、leetcode27:移除元素

思路:双指针算法 + 置换

左右两个指针,L指针向右找等于要移除的val的位置停下来,R指针向左找不等于val的位置停下来,然后两个位置的元素置换。如此,右边的就全是要移除的值,左边的数量即为该题的返回值。
当左指针L >= 右指针R时,说明已经全部遍历了一遍了,此时,看下,如果L所在的值为val,说明其前面全都是不等于val的值,比如L为4,说明当前位置下标为4,前面为0、1、2、3这四个元素,返回的数量就是4,也即L的值,反之,L所在的值不等于val,说明当前位置的值及其前面的值,都是不等于val的值,数量 = 索引 + 1,返回L + 1
public class P27 {
public static void main(String[] args) {
int[] array = {1, 0, 9, 0, 6};
System.out.println(remove(array, 0));
}
public static int remove(int[] array, int val) {
if (array == null || array.length == 0) {
return 0;
}
int left = 0;
int right = array.length - 1;
while (left < right) {
// 没找到左边等于val的值前,一直找
while (left < right && array[left] != val) {
left++;
}
// 没找到右边不等于val的值前,一直找
while (left < right && array[right] == val) {
right--;
}
// 置换,把数组中等于value的元素扔右边
int temp = array[left];
array[left] = array[right];
array[right] = temp;
}
if (array[left] == val) {
return left;
} else {
return left + 1;
}
}
}
测试: