前言
本次博客来讲解一下堆的数组实现,好吧还是会结合图例,让大家理解
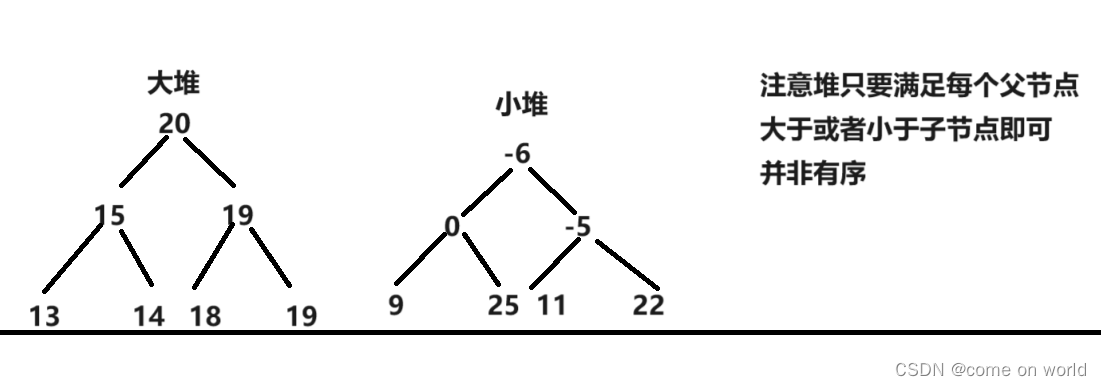
堆的定义
什么是堆?
堆是一颗完全二叉树。它的性质是父节点一定大于或者一定小于子节点
每一个结点都要满足这个性质就是堆
堆的特性是堆顶的数据一定是最大或最小,最大为大堆,最小为小堆
看图
那我们如何实现堆呢?
我们可以注意堆是一个完全二叉树,我们可以使用一个数组来模拟完全二叉树
那么如何使用数组实现完全二叉树
使用数组实现完全二叉树
OK,首先我们可以通过数组下标,来确定节点,只要能够得到父子关系就可以遍历整个完全二叉树,看图吧

OK,那么咱么可不可以实现一下前序遍历呢
使用递归和非递归
#define _CRT_SECURE_NO_WARNINGS 1
#include<stdio.h>
#include<stdlib.h>
#define N 10
//适用于满二叉树和完全二叉树
typedef struct BianryTree {
int a[N];
}BT;
void InitBinaryTree(BT* bt,int cursize)
{
if (cursize >= N)
return;
bt->a[cursize] = cursize;
InitBinaryTree(bt,cursize*2+1);
InitBinaryTree(bt,cursize*2+2);
}
void TraversalTree(BT* bt, int cursize)
{
if (cursize >= N)
return;
printf("%d ", bt->a[cursize]);
TraversalTree(bt, cursize * 2 + 1);
TraversalTree(bt, cursize * 2 + 2);
}
int main()
{
BT* bt = (BT*)malloc(sizeof(BT));
InitBinaryTree(bt,0);
TraversalTree(bt, 0);
return 0;
}上面是前序遍历属于递归遍历
接下来看非递归遍历,其实可以
void TraversalTree(BT* bt, int cursize)
{
while (cursize < N)
printf("%d ", bt[cursize++]);
}只需改一改就好,这里相当于层序遍历
那么使用数组去实现完全二叉树是可行的
数组实现堆
我们先看看堆有哪些接口,然后一一实现
看一看头文件吧
#pragma once
#include<stdio.h>
#include<stdlib.h>
#include<stdbool.h>
#include<assert.h>
typedef int HpDatatype;
typedef struct Heap {
HpDatatype* a;
int capacity;
int size;
}HP;
void IintHeap(HP* hp);
void PushHeap(HP* hp, HpDatatype x);
void PopHeap(HP* hp);
HpDatatype TopHeap(HP* hp);
void DestroyHeap(HP* hp);
bool EmptyHeap(HP* hp);
int SizeHeap(HP* hp);OK
先实现几个简单的 初始化,没什么好说的
void IintHeap(HP* hp)
{
hp->a = NULL;
hp->capacity = 0;
hp->size = 0;
}返回堆大小,也没有什么好说的
int SizeHeap(HP* hp)
{
assert(hp);
return hp->size;
}返回堆顶
HpDatatype TopHeap(HP* hp)
{
assert(hp);
assert(hp->size > 0);
return hp->a[0];
}判空
bool EmptyHeap(HP* hp)
{
assert(hp);
return hp->size == 0;
}销毁堆
void DestroyHeap(HP* hp)
{
assert(hp);
free(hp->a);
hp->capacity = 0;
hp->size = 0;
}插入数据
void PushHeap(HP* hp, HpDatatype x)
{
assert(hp);
//检查容量
if (hp->size == hp->capacity)
{
int newcapacity = hp->capacity == 0 ? 4 : hp->capacity * 2;
HpDatatype* temp = (HpDatatype*)realloc(hp->a, sizeof(HpDatatype) * newcapacity);
if (temp == NULL)
{
printf("realloc fail\n");
return;
}
else
{
hp->a=temp;
hp->capacity = newcapacity;
}
}
//向上调整法
hp->a[hp->size++] = x;
AdjustUp(hp->a,hp->size-1);
}这个代码的逻辑是,每插入一个数据,对该数据进行向上调整法
那么向上调整法可以确保,每插入一个数据,该数组保持为堆
那么现在我们画图来理解什么是向上调整法
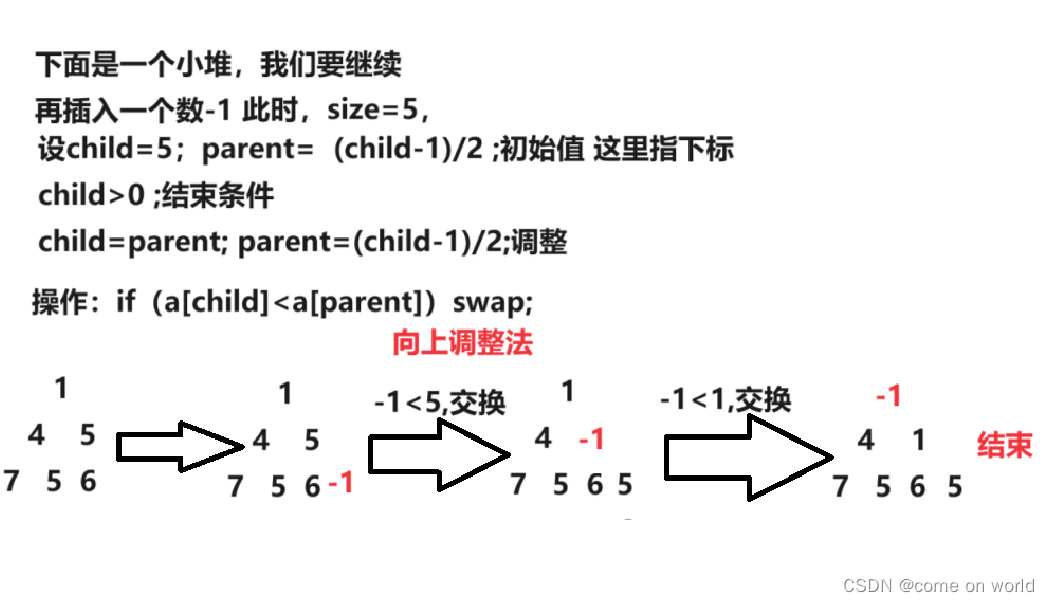
OK,下面是向上调整法
void AdjustUp(HpDatatype* a, int child)
{
//这里的向上调整法是调整它的祖先
while (child > 0)
{
int parent = (child - 1) / 2;
if (a[child] < a[parent])
{
int temp = a[child];
a[child] = a[parent];
a[parent] = temp;
child = parent;
}
else
{
break;
}
}
}
删除一个数据
void PopHeap(HP* hp)
{
assert(hp);
assert(hp->a > 0);
swap(&hp->a[0], &hp->a[hp->size - 1]);
//向下调整法
AdjustDown(hp->a, 0, hp->size);
hp->size--;
}我们要删除的是堆顶的数据
所以让第一个数据与最后一个数据交换,size--
此时,第一个节点的左子树和右子树都是堆,我们只要再调整一下根节点即可
这种调整叫做向下调整法
这里就不画图了,有点麻烦
直接看代码吧
void AdjustDown(HpDatatype* a, int start, int end)
{
int parent = start;
//假设法,假设左孩子更接近目的
int child = parent * 2 + 1;
end = end - 1;
while (child <end)
{
if (child + 1 < end && a[child + 1] < a[child])
child++;
if (a[parent] > a[child])
{
swap(&a[child],&a[parent]);
parent = child;
child = parent * 2 + 1;
}
else
break;
}
}这里的逻辑就是,先使用假设法让child唯一
然后,比对a[child]和a[parent]的大小 满足条件则交换
大堆 a[child]>a[parent] 小堆 a[child]<a[parent]
如果不满足条件,直接结束.
测试堆
看图吧
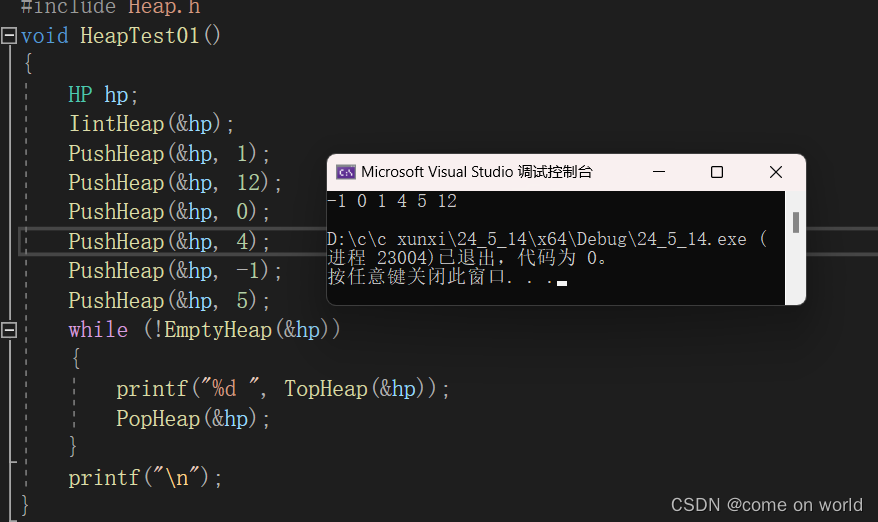
这是小堆,所以是升序,也算是完成了