一 、哈希表
1.1 哈希表原理精讲
哈希表-散列表,它是基于快速存取的角度设计的,也是一种典型的“空间换时间”的做法
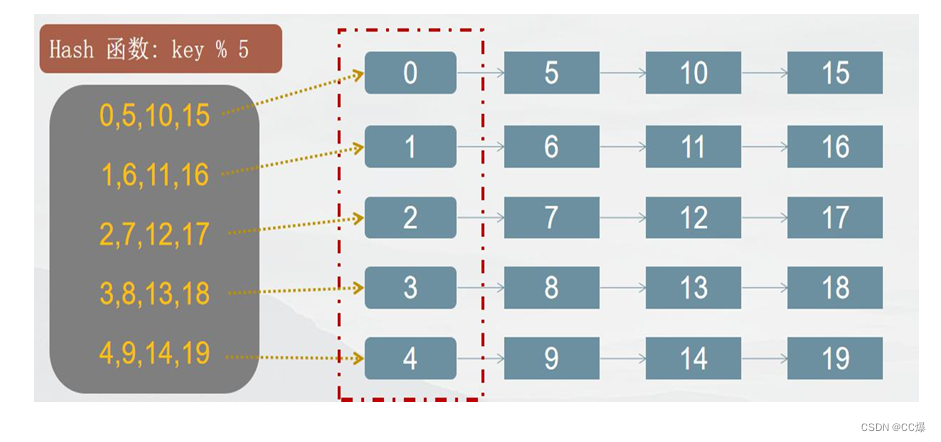
键(key): 组员的编号如,1、5、19。。。
值(value): 组员的其它信息(包含性别、年龄和战斗力等)
索引: 数组的下标(0,1,2,3,4),用以快速定位和检索数据
哈希桶: 保存索引的数组(链表或数组),数组成员为每一个索引值相同的多个元素
哈希函数: 将组员编号映射到索引上,采用求余法,如:组员编号 19
1.2 哈希链表算法实现
1.2.1 结构体定义
#define DEFAULT_SIZE 16
/*哈希表元素定义*/
typedef struct _ListNode
{
struct _ListNode* next;
int key;
void* data;
}ListNode;
typedef ListNode* List;
typedef ListNode* Element;
/*哈希表结构定义*/
typedef struct _HashTable
{
int TableSize;
List* Thelists;
}HashTable;
1.2.2 哈希函数
/*根据key计算索引,定位Hash桶的位置*/
int Hash(int key, int TableSize)
{
return(key % TableSize);
}
1.2.3 链表初始化
/*初始化哈希表*/
HashTable* InitHash(int TableSize)
{
int i = 0;
HashTable* hTable = NULL;
if (TableSize <= 0) {
TableSize = DEFAULT_SIZE;
}
hTable = (HashTable*)malloc(sizeof(HashTable));
if (NULL == hTable)
{
printf("HashTable malloc error.\n");
return NULL;
}
hTable->TableSize = TableSize;
//为Hash桶分配内存空间,其为一个指针数组
hTable->Thelists = (List*)malloc(sizeof(List) * TableSize);
if (NULL == hTable->Thelists)
{
printf("HashTable malloc error\n");
free(hTable);
return NULL;
}
//为Hash桶对应的指针数组初始化链表节点
for (i = 0; i < TableSize; i++)
{
hTable->Thelists[i] = (ListNode*)malloc(sizeof(ListNode));
if (NULL == hTable->Thelists[i])
{
printf("HashTable malloc error\n");
free(hTable->Thelists);
free(hTable);
return NULL;
}
else
{
memset(hTable->Thelists[i], 0, sizeof(ListNode));
}
}
return hTable;
}
1.2.4 插入元素
/*从哈希表中根据键值查找元素*/
Element Find(HashTable* HashTable, int key)
{
int i = 0;
List L = NULL;
Element e = NULL;
i = Hash(key, HashTable->TableSize);
L = HashTable->Thelists[i];
e = L->next;
while (e != NULL && e->key != key)
e = e->next;
return e;
}
/*哈希表插入元素,元素为键值对*/
void Insert(HashTable* HashTable, int key, void* value)
{
Element e = NULL, tmp = NULL;
List L = NULL;
e = Find(HashTable, key);
if (NULL == e) // 目前表中还不存在
{
tmp = (Element)malloc(sizeof(ListNode));
if (NULL == tmp)
{
printf("malloc error\n");
return;
}
// 找到属于哪一个桶
L = HashTable->Thelists[Hash(key, HashTable->TableSize)];
tmp->data = value;
tmp->key = key;
tmp->next = L->next;
L->next = tmp; // 经典头插法
}
else
printf("the key alreadye xist\n");
}
1.2.5 查找元素
/*从哈希表中根据键值查找元素*/
Element Find(HashTable* HashTable, int key)
{
int i = 0;
List L = NULL;
Element e = NULL;
i = Hash(key, HashTable->TableSize);
L = HashTable->Thelists[i];
e = L->next;
while (e != NULL && e->key != key)
e = e->next;
return e;
}
1.2.6 删除元素
/*哈希表删除元素,元素为键值对*/
void Delete(HashTable* HashTable, int key)
{
Element e = NULL, last = NULL;
List L = NULL;
int i = Hash(key, HashTable->TableSize);
L = HashTable->Thelists[i];
last = L;
e = L->next;
while (e != NULL && e->key != key) {
last = e;
e = e->next;
}
if (e) {//如果键值对存在
last->next = e->next;
delete(e);
}
}
1.2.7 提取元素数据
/*哈希表元素中提取数据*/
void* Retrieve(Element e)
{
return e ? e->data : NULL;
}
1.2.8 销毁
/*销毁哈希表*/
void Destory(HashTable* HashTable)
{
int i = 0;
List L = NULL;
Element cur = NULL, next = NULL;
for (i = 0; i < HashTable->TableSize; i++)
{
L = HashTable->Thelists[i];
cur = L->next;
while (cur != NULL)
{
next = cur->next;
free(cur);
cur = next;
}
free(L);
}
free(HashTable->Thelists);
free(HashTable);
}
1.3 完整代码实现
bash.h 头文件
#pragma once
#define DEFAULT_SIZE 16
/*哈希表元素定义*/
typedef struct _ListNode
{
struct _ListNode* next;
int key;
void* data;
}ListNode;
typedef ListNode* List;
typedef ListNode* Element;
/*哈希表结构定义*/
typedef struct _HashTable
{
int TableSize;
List* Thelists;
}HashTable;
/*哈希函数*/
int Hash(void* key, int TableSize);
/*初始化哈希表*/
HashTable* InitHash(int ableSize);
/*哈希表插入*/
void Insert(HashTable* HashTable, int key, void* value);
/*哈希表查找*/
Element Find(HashTable* HashTable, int key);
/*哈希表销毁*/
void Destory(HashTable* HashTable);
/*哈希表元素中提取数据*/
void* Retrieve(Element e);
bash.cpp 源文件
#include<stdio.h>
#include<stdlib.h>
#include<string.h>
#include"hash.h"
#include<bits/stdc++.h>
using namespace std;
/*根据key计算索引,定位Hash桶的位置*/
int Hash(int key, int TableSize)
{
return(key % TableSize);
}
/*初始化哈希表*/
HashTable* InitHash(int TableSize)
{
int i = 0;
HashTable* hTable = NULL;
if (TableSize <= 0) {
TableSize = DEFAULT_SIZE;
}
hTable = (HashTable*)malloc(sizeof(HashTable));
if (NULL == hTable)
{
printf("HashTable malloc error.\n");
return NULL;
}
hTable->TableSize = TableSize;
//为Hash桶分配内存空间,其为一个指针数组
hTable->Thelists = (List*)malloc(sizeof(List) * TableSize);
if (NULL == hTable->Thelists)
{
printf("HashTable malloc error\n");
free(hTable);
return NULL;
}
//为Hash桶对应的指针数组初始化链表节点
for (i = 0; i < TableSize; i++)
{
hTable->Thelists[i] = (ListNode*)malloc(sizeof(ListNode));
if (NULL == hTable->Thelists[i])
{
printf("HashTable malloc error\n");
free(hTable->Thelists);
free(hTable);
return NULL;
}
else
{
memset(hTable->Thelists[i], 0, sizeof(ListNode));
}
}
return hTable;
}
/*从哈希表中根据键值查找元素*/
Element Find(HashTable* HashTable, int key)
{
int i = 0;
List L = NULL;
Element e = NULL;
i = Hash(key, HashTable->TableSize);
L = HashTable->Thelists[i];
e = L->next;
while (e != NULL && e->key != key)
e = e->next;
return e;
}
/*哈希表插入元素,元素为键值对*/
void Insert(HashTable* HashTable, int key, void* value)
{
Element e = NULL, tmp = NULL;
List L = NULL;
e = Find(HashTable, key);
if (NULL == e)
{
tmp = (Element)malloc(sizeof(ListNode));
if (NULL == tmp)
{
printf("malloc error\n");
return;
}
L = HashTable->Thelists[Hash(key, HashTable->TableSize)];
tmp->data = value;
tmp->key = key;
tmp->next = L->next;
L->next = tmp;
}
else
printf("the key alreadye xist\n");
}
/*哈希表删除元素,元素为键值对*/
void Delete(HashTable* HashTable, int key)
{
Element e = NULL, last = NULL;
List L = NULL;
int i = Hash(key, HashTable->TableSize);
L = HashTable->Thelists[i];
last = L;
e = L->next;
while (e != NULL && e->key != key) {
last = e;
e = e->next;
}
if (e) {//如果键值对存在
last->next = e->next;
delete(e);
}
}
/*哈希表元素中提取数据*/
void* Retrieve(Element e)
{
return e ? e->data : NULL;
}
/*销毁哈希表*/
void Destory(HashTable* HashTable)
{
int i = 0;
List L = NULL;
Element cur = NULL, next = NULL;
for (i = 0; i < HashTable->TableSize; i++)
{
L = HashTable->Thelists[i];
cur = L->next;
while (cur != NULL)
{
next = cur->next;
free(cur);
cur = next;
}
free(L);
}
free(HashTable->Thelists);
free(HashTable);
}
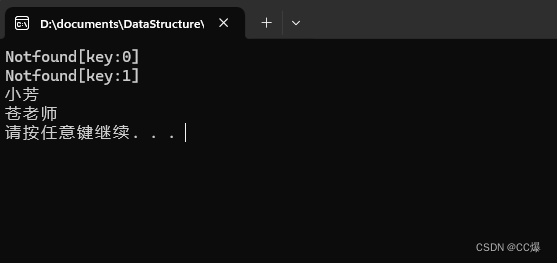
void main(void)
{
const char* elems[] = { "催化","小芳","苍老师"};
int i = 0;
HashTable* HashTable;
HashTable = InitHash(31);
Insert(HashTable, 1, (char*)(elems[0]));
Insert(HashTable, 2, (char*)(elems[1]));
Insert(HashTable, 3, const_cast<char*>(elems[2]));
Delete(HashTable, 1);
for (i = 0; i < 4; i++) {
Element e = Find(HashTable, i);
if (e) {
printf("%s\n", (char *)Retrieve(e));
//cout << (string*)Retrieve(e) << endl;
}
else {
printf("Notfound[key:%d]\n", i);
}
}
system("pause");
}
运行结果:
二、图
2.1 图的原理精讲
2.2 图的算法实现
2.2.1 结构体定义
#include <bits/stdc++.h>
using namespace std;
#define MaxSize 1024
// 与节点连接的边的定义
typedef struct _EdgeNode {
int adjvex; // 领接的顶点
int weight; // 边的权重
struct _EdgeNode* next; // 下一条边
}EdgeNode;
// 顶点节点
typedef struct _VertexNode {
char data; // 节点数据
struct _EdgeNode* first; // 指向邻接的第一条边
}VertexNode,AdjList;
// 定义邻接表
typedef struct _AdjListGraph {
AdjList* adjlist;
int vex; // 顶点数
int edge;// 边数
}AdjListGraph;
2.2.2 初始化创建图
// 图的初始化
void Init(AdjListGraph& G) {
G.adjlist = new AdjList[MaxSize]; // 属于直接创建最大值链表数量
G.vex = 0;
G.edge = 0;
}
// 图的创建
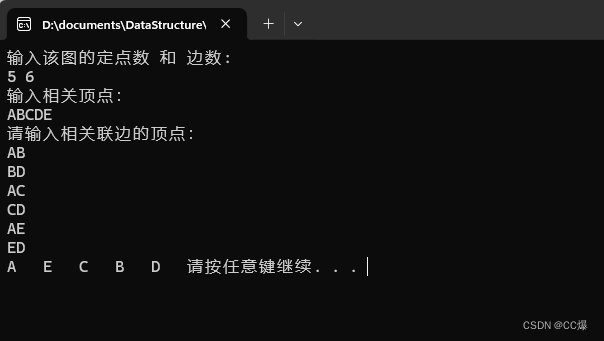
void Great(AdjListGraph& G) {
cout << "输入该图的定点数 和 边数: " << endl;
cin >> G.vex >> G.edge;
cout << "输入相关顶点: " << endl;
for (int i = 0; i < G.vex; i++) {
cin >> G.adjlist[i].data;
G.adjlist[i].first = NULL;
}
char v1 = 0, v2 = 0; //保存输入顶点的字符
int i1, i2; // 保存顶点在数组中的下标
cout << "请输入相关联边的顶点:" << endl;
for (int i = 0; i < G.edge; i++) {
cin >> v1 >> v2; // 代表 v1 v2 之间是相互连接的
i1 = Location(G, v1); // 查找 v1 这个点下标在哪里
i2 = Location(G, v2);
if (i1 != -1 && i2 != -1) { // 代表两个点都找到了
EdgeNode* temp = new EdgeNode;
temp->adjvex = i2; // 代表,1可以找到2,所以1可以访问到2的链表
temp->next = G.adjlist[i1].first; // 头插法
G.adjlist[i1].first = temp;
}
}
}
// 通过顶点对应的字符寻找顶点在图中的领接点
// 用来查找这个点在图的下标是多少
int Location(AdjListGraph& G, char v) {
for (int i = 0; i < G.vex; i++) {
if (G.adjlist[i].data == v)
{
return i;
}
}
return -1;
}
2.3 深度优先遍历
思想:
1 首先以一个未被访问过的顶点作为起始顶点,沿当前顶点的边走到未访问过的顶点;
2 当没有未访问过的顶点时,则回到上一个顶点,继续试探别的顶点,直到所有的顶点都被访问过。
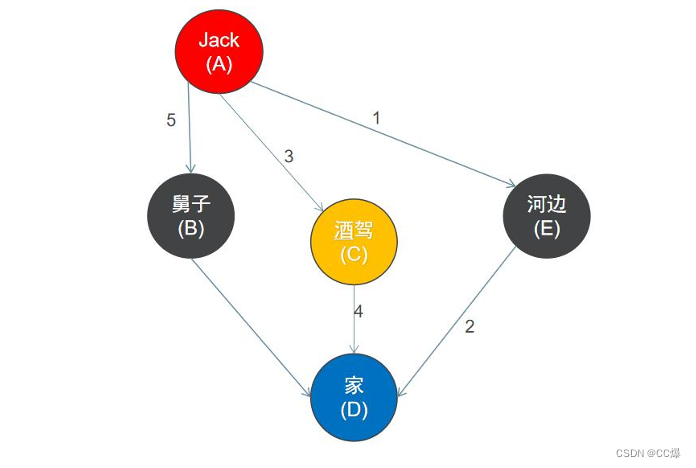
具体过程:
1. 首先从一个未走到过的顶点作为起始顶点,比如A顶点作为起点。
2. 沿A顶点的边去尝试访问其它未走到过的顶点,首先发现E号顶点还没有走到过,于是访问E顶点。
3. 再以E顶点作为出发点继续尝试访问其它未走到过的顶点,接下来访问D顶点。
4. 再尝试以D顶点作为出发点继续尝试访问其它未走到过的顶点。
5. 但是,此时沿D顶点的边,已经不能访问到其它未走到过的顶点,接下来返回到E顶点。
6. 返回到E顶点后,发现沿E顶点的边也不能再访问到其它未走到过的顶点。此时又回到顶点A(D->E->A),再以A顶点作为出发点继续访问其它未走到过的顶点,于是接下来访问C顶点。
7. 。。。。。。。。。。
8. 最终访问的结果是 A->E->D->C->B(这个不唯一的,看自己怎么选择路径)
// 对图上的一个顶点进行深度遍历 DFS
void DFS(AdjListGraph& G, int v) {
int next = -1;
if (visited[v]) { // 代表这个节点被访问过
return;
}
cout << "节点未被访问过:" << G.adjlist[v].data;
visited[v] = true; // 设置为已访问;
EdgeNode* temp = G.adjlist[v].first;
//if (temp != NULL) { // 访问下一个节点
// next = temp->adjvex;
// if (visited[next] == false) {
// DFS(G, next);
// }
// while (temp->next) {
// temp = temp->next;
// next = temp->adjvex;
// if (visited[next] == false) {
// DFS(G, next);
// }
// }
//}
// 直接整合为一步解决
while (temp) {
next = temp->adjvex;
temp = temp->next;
if (visited[next] == false) {
DFS(G, next);
}
}
}
// 所有顶点遍历
void DFS_Main(AdjListGraph& G) {
for (int i =0; i < G.vex; i++) {
if (visited[i] == false) {
DFS(G, i);
}
}
}
2.3 广度优先遍历
思想:
1 首先以一个未被访问过的顶点作为起始顶点,访问其所有相邻的顶点;
2 然后对每个相邻的顶点,再访问它们相邻的未被访问过的顶点,直到所有顶点都被访问过,遍历结束。

// 对一个顶点进行BFS
void BFS(AdjListGraph& G,int v) {
queue<int> q;
int cur = -1; // 当前处理节点
int index = -1; // 位置
q.push(v); // 入队
while (!q.empty()) { // 队列非空
cur = q.front(); // 取队列头元素
if (visited[cur] == false) { // 当前节点未访问
cout << G.adjlist[cur].data << " ";
visited[cur] = true;
}
q.pop(); // 队头出队,因为已经访问过了
EdgeNode* temp = G.adjlist[cur].first;
while (temp != NULL)
{
index = temp->adjvex;
temp = temp->next;
q.push(index);
}
}
}
// 所有顶点遍历广度优先遍历
void BFS_Main(AdjListGraph& G) {
for (int i = 0; i < G.vex; i++) {
if (visited[i] == false) {
BFS(G, i);
}
}
}
2.4 最短路径算法
从起点开始访问所有路径,则到达终点节点的路径有多条,其中路径权值最短的一条则为最短路径。
最短路径算法: 深度优先遍历、广度优先遍历、Bellman-Ford算法、弗洛伊德算法SPFA(ShortestPathFasterAlgorithm)算法和迪杰斯特拉算法等。
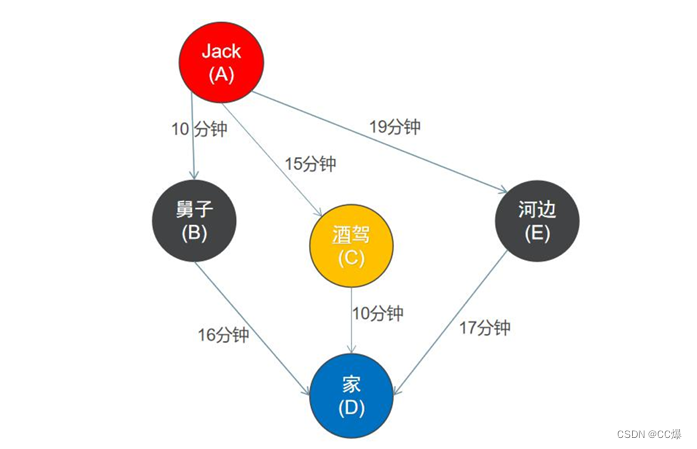
此处时间就相当于权重,我们采用 DFS 搜索算法进行最短路搜索:
此处,和之前写的DFS遍历,就在其基础上引入了权重.
最重要一点:就是回溯 ,因为我们遍历也需要保存路径,需要将每条路就过一遍,就存在,或许有的节点再另一条路劲可能访问过了,就需要回退,设置为未访问。
以下为搜索的主要代码:
int min_weights = 0x7FFFFFFF; // 默认最大
int steps = 0; // 已走过的步数
int path[MaxSize] = { 0 }; // 保存走过的路径
int short_path[MaxSize] = {0}; // 保存最短路劲
// 通过深度遍历,求解起点到终点最短路
void DFS(AdjListGraph& G,int start,int end, int weights) {
int cur = -1; // 用来查看当前下标
if (start == end) { // 代表已经找到终点,不用继续遍历了
for (int i = 0; i < steps; i++) {
cout << G.adjlist[path[i]].data << " "; // 可能的路径
}
cout << endl;
cout << "权重: " <<weights << endl;
if (min_weights > weights) {
min_weights = weights;
memcpy(short_path, path, steps*sizeof(int));
}
}
visited[start] = true; // 设置为已访问
EdgeNode* temp = G.adjlist[start].first;
while (temp) {
int weight = temp->weight; // 权重
cur = temp->adjvex; // 当前下标
// 防止转圈,防止进入环
if (visited[cur] == false) {
visited[cur] = true; // 当前点已访问
path[steps++] = temp->adjvex; // 保存路径到path数组
DFS(G, cur, end, weights + weight);
// 前一步探索完成后,让cur顶点为未选择状态,以便后续节点还可以访问
visited[cur] = false; // 回溯
path[--steps] = 0;
}
temp = temp->next;
}
}
完整代码:
#include <bits/stdc++.h>
using namespace std;
#define MaxSize 1024
// 与节点连接的边的定义
typedef struct _EdgeNode {
int adjvex; // 领接的顶点
int weight; // 边的权重
struct _EdgeNode* next; // 下一条边
}EdgeNode;
// 顶点节点
typedef struct _VertexNode {
char data; // 节点数据
struct _EdgeNode* first; // 指向邻接的第一条边
}VertexNode, AdjList;
// 定义邻接表
typedef struct _AdjListGraph {
AdjList* adjlist; //
int vex; // 顶点数
int edge;// 边数
}AdjListGraph;
bool visited[MaxSize]; //全局数组,用来记录节点是否已被访问
int Location(AdjListGraph& G, char v);
// 图的初始化
void Init(AdjListGraph& G) {
G.adjlist = new AdjList[MaxSize]; // 属于直接创建最大值链表数量
G.vex = 0;
G.edge = 0;
}
// 图的创建
void Great(AdjListGraph& G) {
cout << "输入该图的定点数 和 边数: " << endl;
cin >> G.vex >> G.edge;
cout << "输入相关顶点: " << endl;
for (int i = 0; i < G.vex; i++) {
cin >> G.adjlist[i].data;
G.adjlist[i].first = NULL;
}
char v1 = 0, v2 = 0; //保存输入顶点的字符
int i1, i2; // 保存顶点在数组中的下标
int weight = 0 ; // 保存边的权重
cout << "请输入相关联边的顶点:" << endl;
for (int i = 0; i < G.edge; i++) {
cin >> v1 >> v2 >> weight; // 代表 v1 v2 之间是相互连接的,和这个边权重
i1 = Location(G, v1); // 查找 v1 这个点下标在哪里
i2 = Location(G, v2);
if (i1 != -1 && i2 != -1) { // 代表两个点都找到了
EdgeNode* temp = new EdgeNode;
temp->weight = weight;
temp->adjvex = i2; // 代表,1可以找到2,所以1可以访问到2的链表
temp->next = G.adjlist[i1].first; // 头插法
G.adjlist[i1].first = temp;
}
}
}
// 通过顶点对应的字符寻找顶点在图中的领接点
// 用来查找这个点在图的下标是多少
int Location(AdjListGraph& G, char v) {
for (int i = 0; i < G.vex; i++) {
if (G.adjlist[i].data == v)
{
return i;
}
}
return -1;
}
int min_weights = 0x7FFFFFFF; // 默认最大
int steps = 0; // 已走过的步数
int path[MaxSize] = { 0 }; // 保存走过的路径
int short_path[MaxSize] = {0}; // 保存最短路劲
// 通过深度遍历,求解起点到终点最短路
void DFS(AdjListGraph& G,int start,int end, int weights) {
int cur = -1; // 用来查看当前下标
if (start == end) { // 代表已经找到终点,不用继续遍历了
for (int i = 0; i < steps; i++) {
cout << G.adjlist[path[i]].data << " "; // 可能的路径
}
cout << endl;
cout << "权重: " <<weights << endl;
if (min_weights > weights) {
min_weights = weights;
memcpy(short_path, path, steps*sizeof(int));
}
}
visited[start] = true; // 设置为已访问
EdgeNode* temp = G.adjlist[start].first;
while (temp) {
int weight = temp->weight; // 权重
cur = temp->adjvex; // 当前下标
// 防止转圈,防止进入环
if (visited[cur] == false) {
visited[cur] = true; // 当前点已访问
path[steps++] = temp->adjvex; // 保存路径到path数组
DFS(G, cur, end, weights + weight);
// 前一步探索完成后,让cur顶点为未选择状态,以便后续节点还可以访问
visited[cur] = false; // 回溯
path[--steps] = 0;
}
temp = temp->next;
}
}
int main() {
AdjListGraph G;
// 初始化
Init(G);
//创建图
Great(G);
// 深度遍历
char start, end;
cout << "输入查询起点终点" << endl;
cin >> start >> end;
// 求两点间的最短路径
path[steps++] = Location(G, start);
DFS(G, Location(G, start), Location(G, end), 0);
cout << "最小路劲长度: " << min_weights << endl;
cout << "路径:";
int i = 0;
while (i < MaxSize && short_path[i]) {
cout << G.adjlist[short_path[i]].data << " ";
i++;
}
cout << endl;
system("pause");
return 0;
}
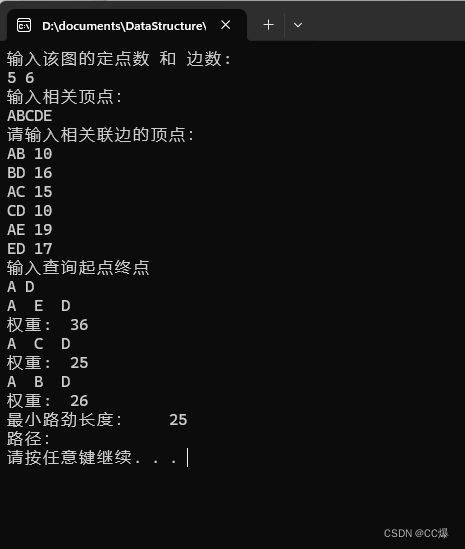
测试结果:
2.5 图的项目案例 (A* 算法)(非常重要)
2.5.1 A*算法原理
什么是A* 算法?
A算法较之传统的路径规划算法,实时性更高、灵活性更强,寻路结果更加接近人工选择的路径结果. A寻路算法并不是找到最优路径,只是找到相对近的路径,因为找最优要把所有可行路径都找出来进行对比,消耗性能太大,寻路效果只要相对近路径就行了*
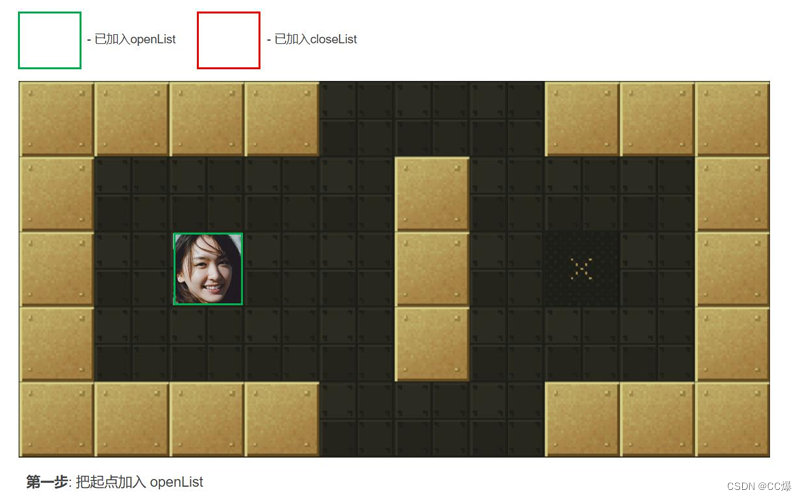
寻路步骤
-
从起点开始, 把它作为待处理的方格存入一个预测可达的节点列表,简称openList, 即把起点放入“预测可达节点列表”,
可达节点列表openList就是一个等待检查方格的列表。 -
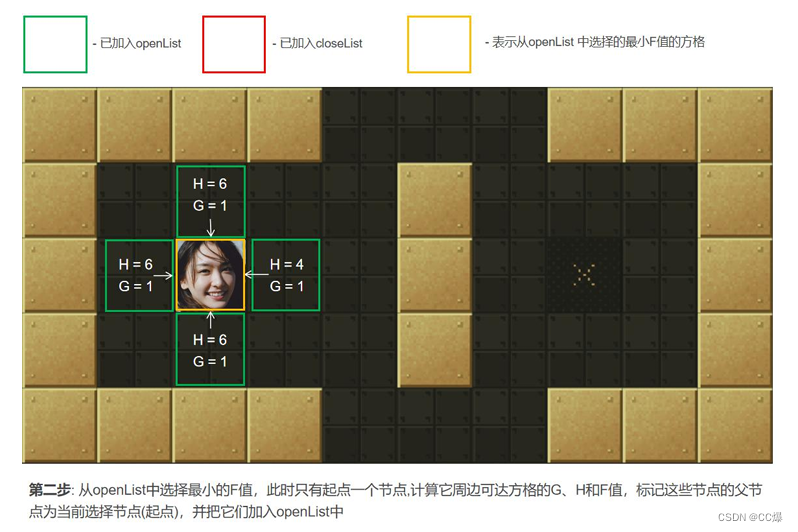
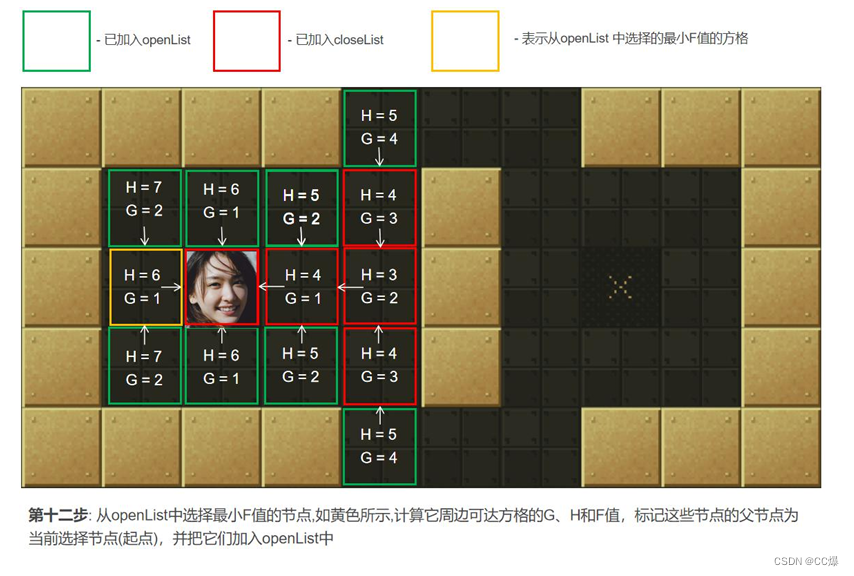
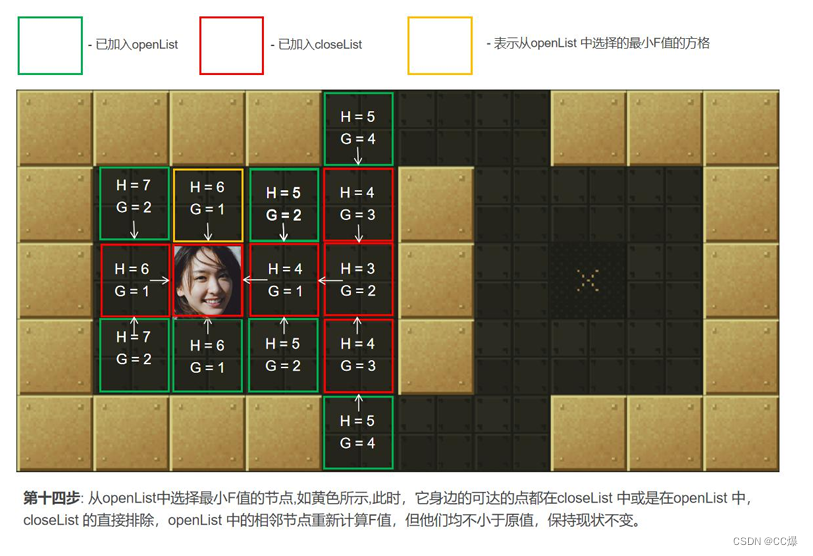
寻找openList中F值最小的点min(一开始只有起点)周围可以到达的方格(可到达的意思是其不是障碍物,也不存
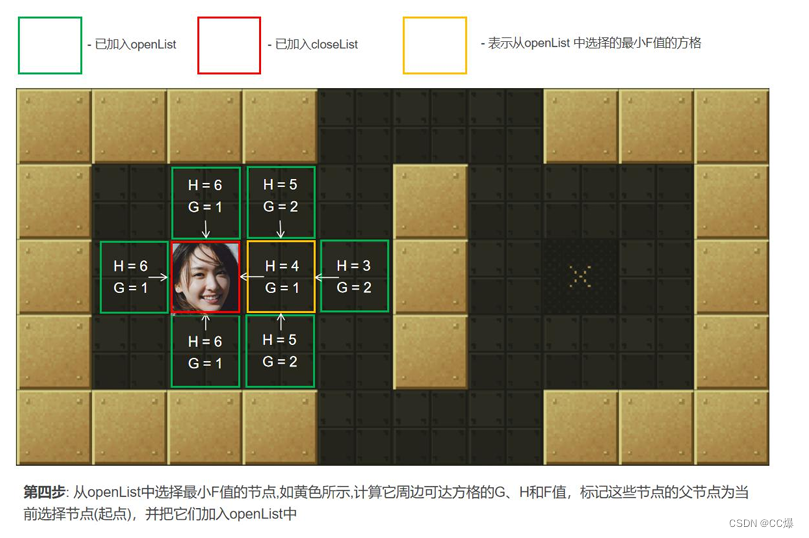
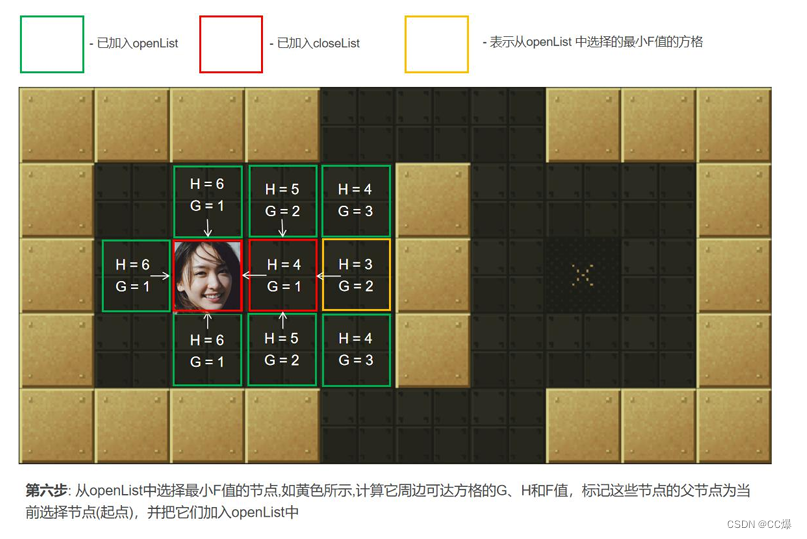
在关闭列表中的方格,即不是已走过的方格)。计算min周围可到达的方格的F值。将还没在openList中点放入其中, 并
设置它们的"父方格"为点min,表示他们的上一步是经过min到达的。如果min下一步某个可到达的方格已经在openList
列表那么并且经min点它的F值更优,则修改F值并把其"父方格"设为点min。 -
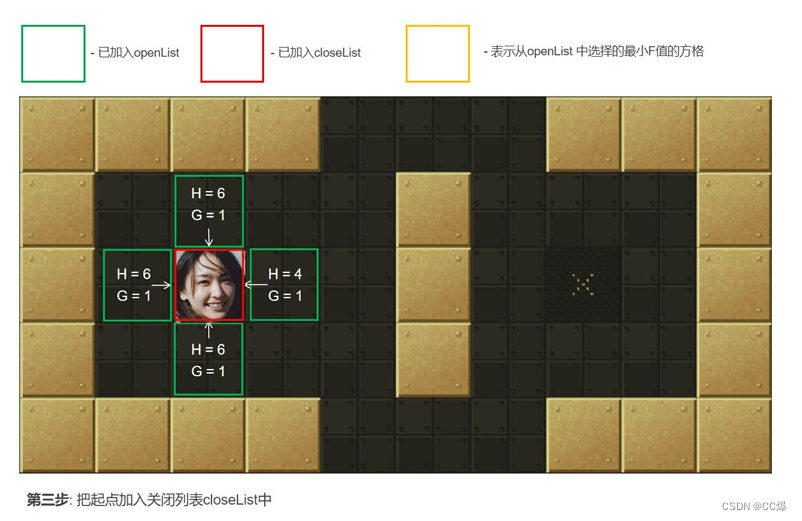
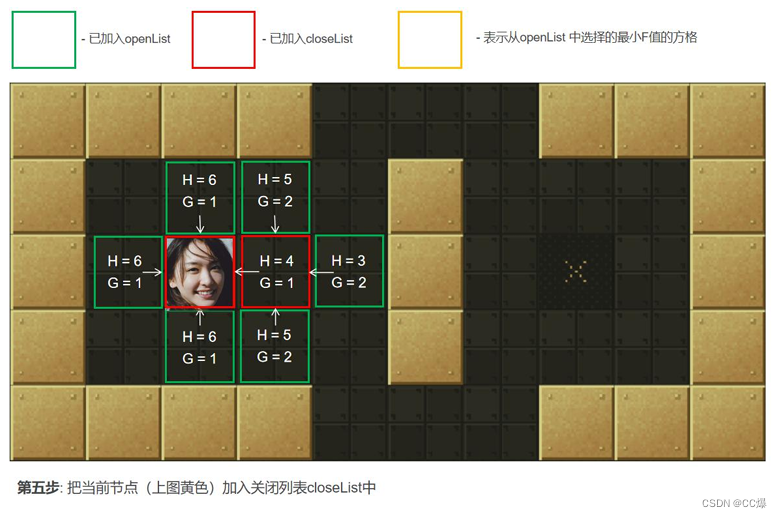
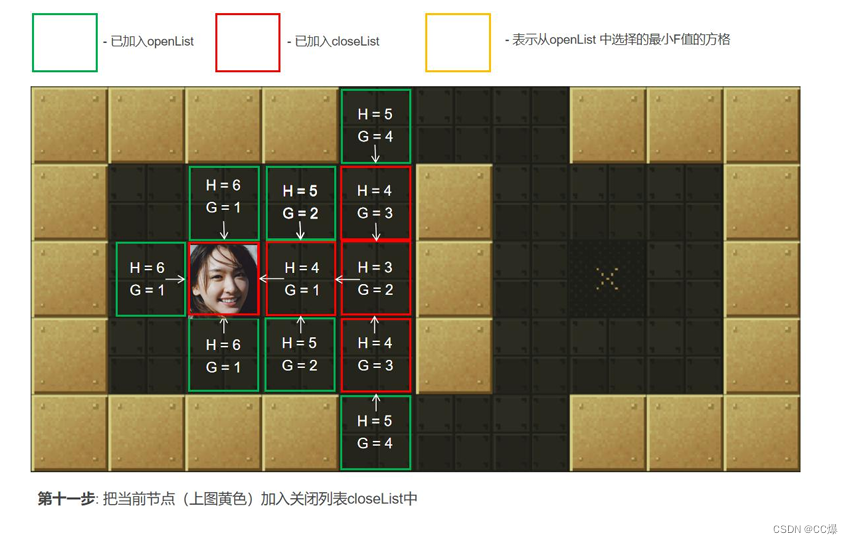
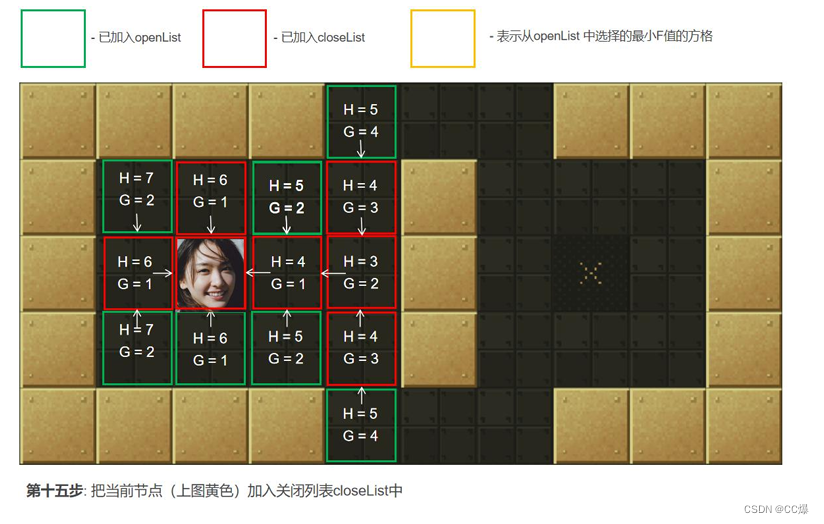
把2中的点min从"开启列表"中删除并存入"关闭列表"closeList中,closeList中存放的都是不需要再次检查的方格。如
果2中点min不是终点并且开启列表的数量大于零,那么继续从第2步开始。如果是终点执行第4步,如果openList列
表数量为零,那么就是找不到有效路径。 -
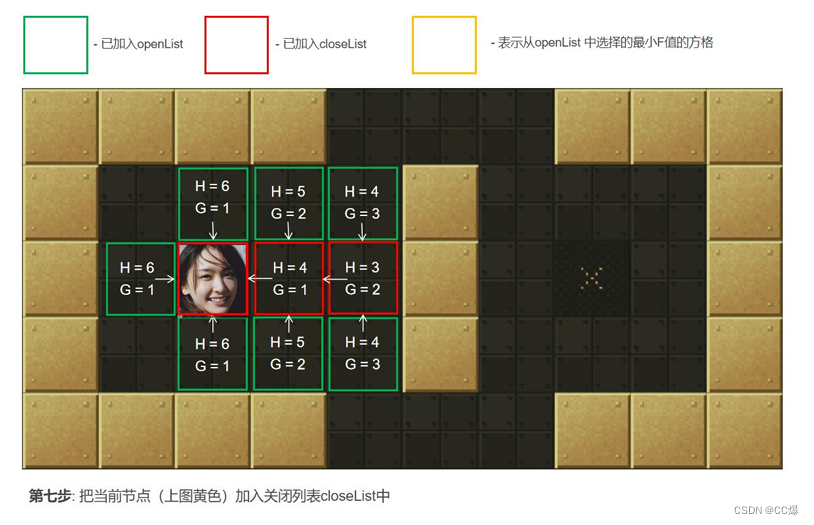
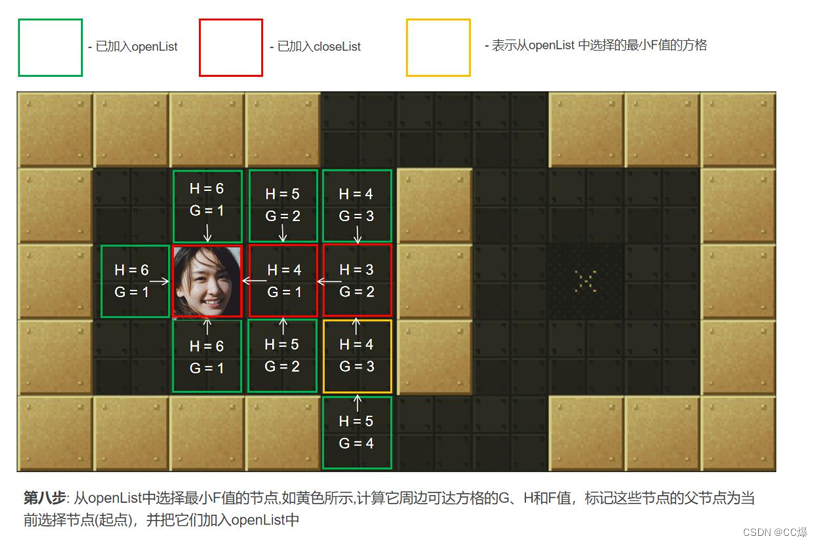
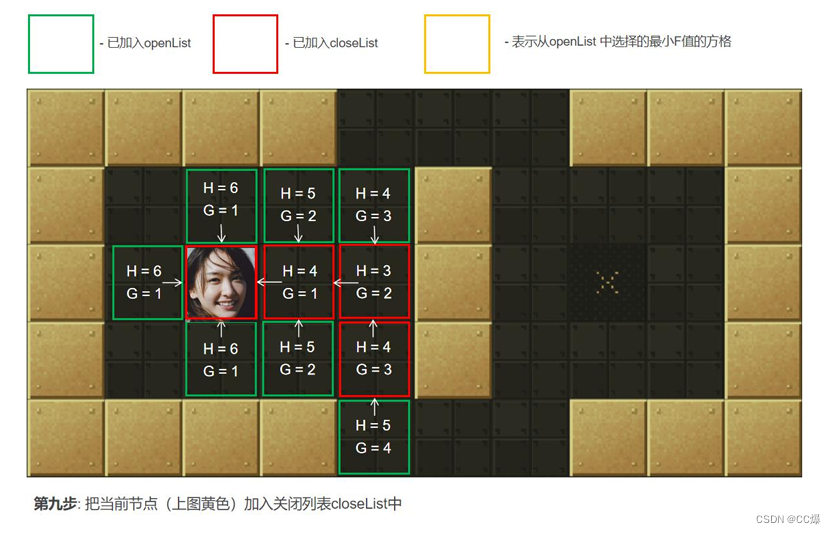
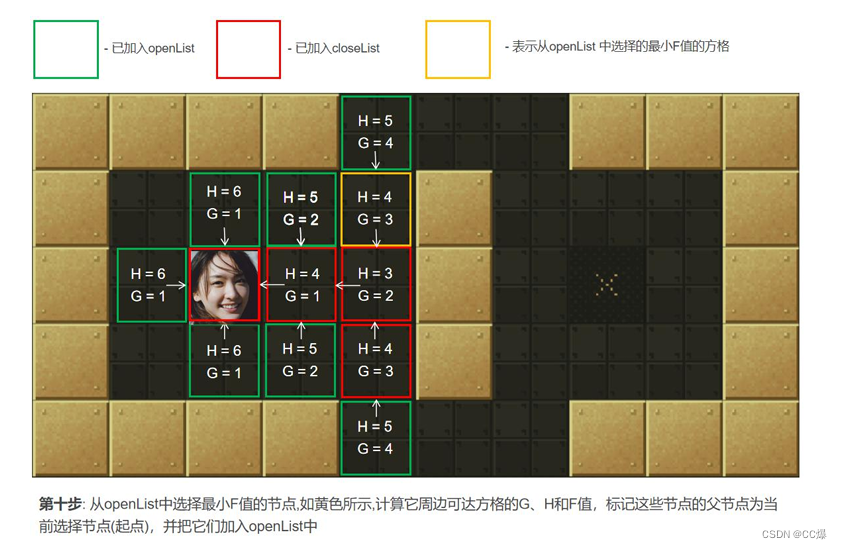
如果第3步中min 是终点,则结束查找,直接追溯父节点到起点的路径即为所选路径。
2.5.2 A*算法图示,一步一步讲解
具体寻路步步骤图示

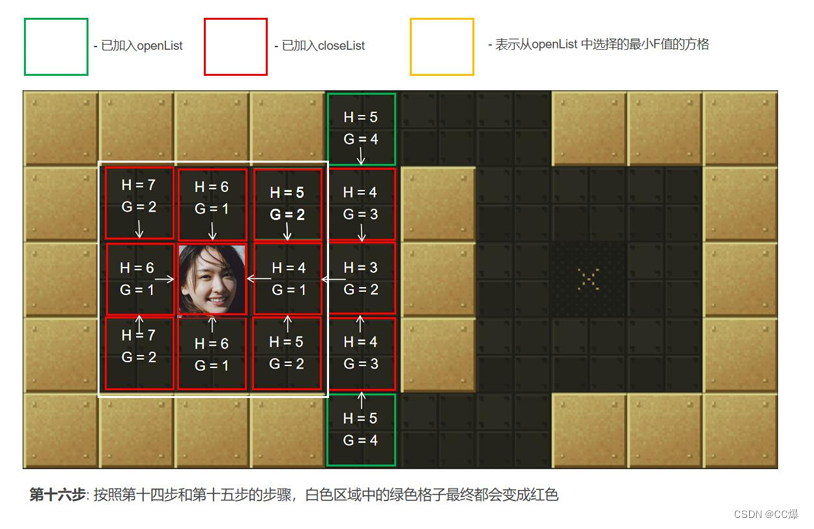
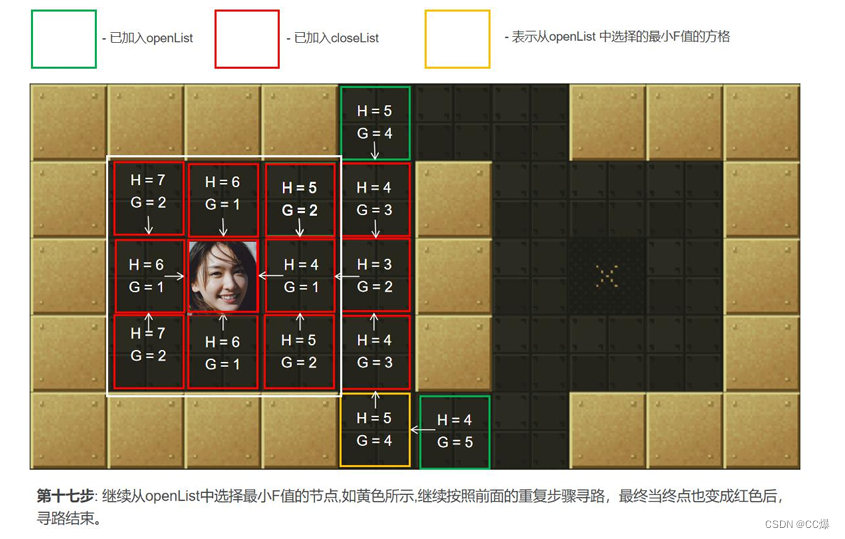
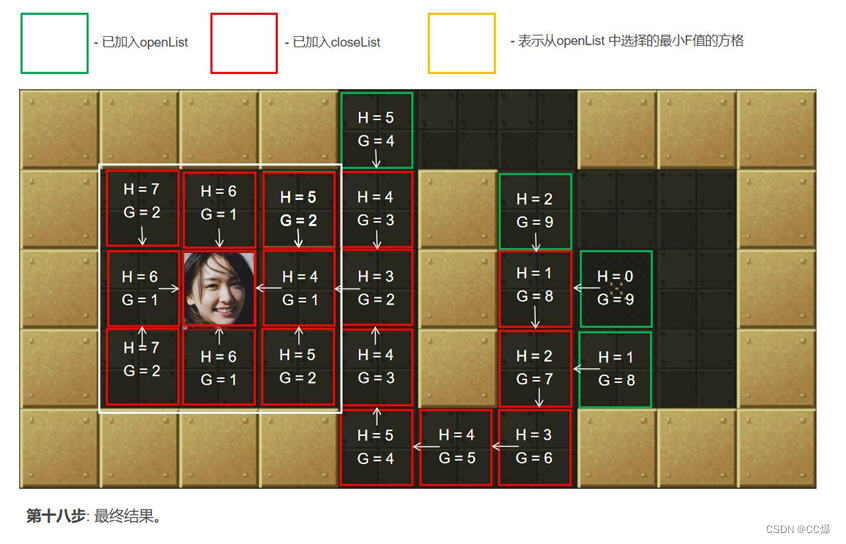
2.5.3 A*算法完整代码
AStar.h
// AStar.h
#pragma once
#include <list>
#include <vector>
using namespace std;
const int kCost1 = 10; // 直移一个消耗
const int kCost2 = 20; // 斜移一个消耗
typedef struct _point {
int x, y; // 点坐标,x为横,y为竖
int F, G, H; // F= G+H , 代表到终点总距离
struct _point* parent; // parent 坐标
}point;
/* 分配一个节点(格子) */
point* AllocPoint(int x, int y);
/* 初始化地图 */
void InitAstarMaze(int* _maze, int _lines, int _colums);
/* 通过A*算法寻找路径 */
list<point*>GetPath(point* startPoint, point* endPoint);
/* 清理资源、结束后调用 */
void ClearAstarMaze();
AStar.cpp
// AStar.cpp
#include<bits/stdc++.h>
#include"AStar.h"
static int* maze; //迷宫对应二维数组,一维指针表示
static int cols; // 二维数组对应列数
static int lines; // 二维数组对应行数
static list<point*> openList; // 开放列表
static list<point*> closeList; // 关闭列表
/* 搜索从起点到终点的最佳路径 */
static point* findPath(point* startPoint, point* endPoint);
/* 从openList里面获取最小的节点 */
static point* getLeastPoint();
/* 获取当前节点的周边节点 */
static vector<point*> getSurroundPoints(const point* curPoint);
/* 判断某点是否可以用于下一步判断 */
static bool isCanreach(const point* curPoint, point* temp);
/* 判断开启/关闭列表中是否包含某点 */
static point* isInList(const std::list<point*>& list, const point* cpoint);
//计算FGH值
static int calG(point* temp_start, point* point);
static int calH(point* cpoint, point* end);
static int calF(point* cpoint);
/* 分配一个节点(格子) */
point* AllocPoint(int x, int y) {
point* temp = new point;
memset(temp, 0, sizeof(point)); // 初始值清0
temp->x = x;
temp->y = y;
return temp;
}
/* 初始化地图 */
void InitAstarMaze(int* _maze, int _lines, int _colums) {
maze = _maze;
lines = _lines;
cols = _colums;
}
/* 通过A*算法寻找路径 */
list<point*> GetPath(point* startPoint, point* endPoint) {
point* result = findPath(startPoint, endPoint);
list<point*> path; // 返回的路径
// 如果没找到路径,则返回空的列表
while (result) {
path.push_front(result);
result = result->parent; // 相当于最后一个节点逆转,比如原本path 是 CBA,逆转为ABC
}
return path;
}
/* 搜索从起点到终点的最佳路径 */
static point* findPath(point* startPoint, point* endPoint) {
// 1. 初始节点放入 OpenList
openList.push_back(AllocPoint(startPoint->x,startPoint->y));
while (!openList.empty()) {
// 2. 寻找openList中最小值
point* curPoint = getLeastPoint(); // 取到最小初始值
// 3. 把当前节点放在关闭列表中
openList.remove(curPoint);
closeList.push_back(curPoint);
// 4. 找到当前节点可到达的周围节点,并计算F值
vector<point*> surroundPoints = getSurroundPoints(curPoint);
vector<point*>::const_iterator it;
for (it = surroundPoints.begin(); it != surroundPoints.end(); it++) {
point* target = *it;
// 对某个格子,如果它不在开放列表中,加入到开启列表,设置当前格为其父节点,计算FGH
point* exist = isInList(openList, target);
if (!exist) {
target->parent = curPoint;
target->G = calG(curPoint, target);
target->H = calH(target, endPoint);
target->F = calF(target);
openList.push_back(target);
}
// 如果已经存在,就从新计算G值,然后看大小更新
else {
int tempG = calG(curPoint, target);
if (tempG < target->G) {
exist->parent = curPoint;
exist->G = tempG;
exist->F = calF(target);
}
delete target;
}
}
// 结束条件
surroundPoints.clear();
point* resPoint = isInList(openList, endPoint); // end 已经在openList,代表已经跑到终点
if (resPoint) {
return resPoint;
}
}
return NULL;
}
/* 从openList里面获取最小的节点 */
static point* getLeastPoint() {
if (!openList.empty()) {
point* resPoint = openList.front();
list<point* >::const_iterator it; // 迭代器
for (it = openList.begin(); it != openList.end(); it++) {
point* p = *it; // 如何获取迭代器数据? 加 *
if (p->F < resPoint->F) {
resPoint = *it;
}
}
return resPoint;
}
return NULL;
}
/* 获取当前节点的周边节点 */
static vector<point*> getSurroundPoints(const point* curPoint) {
vector<point* > surroundPoints;
for (int x = curPoint->x - 1; x <= curPoint->x + 1; x++) {
for (int y = curPoint->y - 1; y <= curPoint->y + 1; y++) { // 周围8个点
point* temp = AllocPoint(x, y);
if (isCanreach(curPoint, temp)) { // 只需要 8个点中的上下左右,斜角此处不考虑
surroundPoints.push_back(temp);
}
else {
delete temp;
}
}
}
return surroundPoints;
}
/* 判断某点是否可以用于下一步判断 */
static bool isCanreach(const point* curPoint, point* temp) { // curPoint 当前点,temp 下一个点
if (temp->x<0 || temp->x>(lines - 1) // x超过范围
|| temp->y<0 || temp->y>(cols - 1) // y超过范围
|| maze[temp->x * cols + temp->y] == 1 // 障碍物
|| maze[temp->x * cols + temp->y] == 2 // 障碍物
|| (temp->x == curPoint->x && temp->y == curPoint->y) // 点从重叠
|| isInList(closeList, temp)) {
return false;
}
// abs 求绝对值
if (abs(curPoint->x - temp->x) + abs(curPoint->y - temp->y) == 1) {
return true;
}
else {
return false;
}
}
/* 判断开启/关闭列表中是否包含某点 */
static point* isInList(const std::list<point*>& list, const point* cpoint){
//判断某个节点是否在列表中,这里不能比较指针,因为每次加入列表是新开辟的节点,只能比较坐标
std::list<point*>::const_iterator itor;
for (itor = list.begin(); itor != list.end(); itor++) {
if ((*itor)->x == cpoint->x && (*itor)->y == cpoint->y) {
return *itor;
}
}
return NULL;
}
//计算FGH值
static int calG(point* temp_start, point* cpoint)
{
// 判断走直线还是斜线,此题只需要直线,斜线以后可以这么写
int extraG = (abs(cpoint->x - temp_start->x) + abs(cpoint->y - temp_start->y)) == 1 ? kCost1 : kCost2;
int parentG = (cpoint->parent == NULL ? NULL : cpoint->parent->G); //如果是初始节点,则其父节点是空
return parentG + extraG;
}
static int calH(point* cpoint, point* end) {
// 就是该点到终点,看成一个矩形,我们就看直线距离,这个看自己怎么算,都可以
return (int)sqrt((double)(end->x - cpoint->x) * (double)(end->x - cpoint->x) +
(double)(end->y - cpoint->y) * (double)(end->y - cpoint->y));
}
static int calF(point* cpoint) {
return cpoint->G + cpoint->H;
}
/* 清理资源、结束后调用 */
void ClearAstarMaze() {
maze = NULL;
lines = 0;
cols = 0;
std::list<point *>::iterator itor;
//清除openList中的元素
cout << openList.size() << endl;
for (itor = openList.begin(); itor != openList.end();) {
delete* itor;
itor = openList.erase(itor);// 获取到下一个节点
}
//清理closeList中的元素
cout << closeList.size() << endl;
for (itor = closeList.begin(); itor != closeList.end();) {
delete* itor;
itor = closeList.erase(itor);
}
}
main.cpp
// main.cpp
#include "AStar.h"
#include<bits/stdc++.h>
#include<windows.h>
using namespace std;
const int ROWS = 13;
const int COLS = 13;
//定义地图数组
int maps[13][13] = {//二维数组在内存顺序存储的
{0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0,},
{0, 1, 0, 1, 0, 1, 0, 1, 0, 1, 0, 1, 0,},
{0, 1, 0, 1, 0, 1, 0, 1, 0, 1, 0, 1, 0,},
{0, 1, 0, 1, 0, 1, 2, 1, 0, 1, 0, 1, 0,},
{0, 1, 0, 1, 0, 1, 0, 1, 0, 1, 0, 1, 0,},
{0, 1, 0, 1, 0, 0, 0, 1, 0, 1, 0, 1, 0,},
{0, 0, 0, 0, 0, 1, 0, 1, 0, 0, 0, 0, 0,},
{2, 0, 1, 1, 0, 0, 0, 1, 0, 1, 1, 0, 2,},
{0, 0, 0, 0, 0, 1, 1, 1, 0, 0, 0, 0, 0,},
{0, 1, 0, 1, 0, 1, 0, 1, 0, 1, 0, 1, 0,},
{0, 1, 0, 1, 0, 1, 0, 1, 0, 1, 0, 1, 0,},
{0, 1, 0, 1, 0, 0, 0, 0, 0, 1, 0, 1, 0,},
{0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0,}
};
void PrintMap(int maps[ROWS][COLS]) {
for (int i = 0; i < ROWS; ++i) {
for (int j = 0; j < COLS; ++j) {
if (maps[i][j] == 0) {
std::cout << '0'; // 通路用空格表示
}
else {
std::cout << '*'; // 墙用星号表示
}
}
std::cout << '\n';
}
}
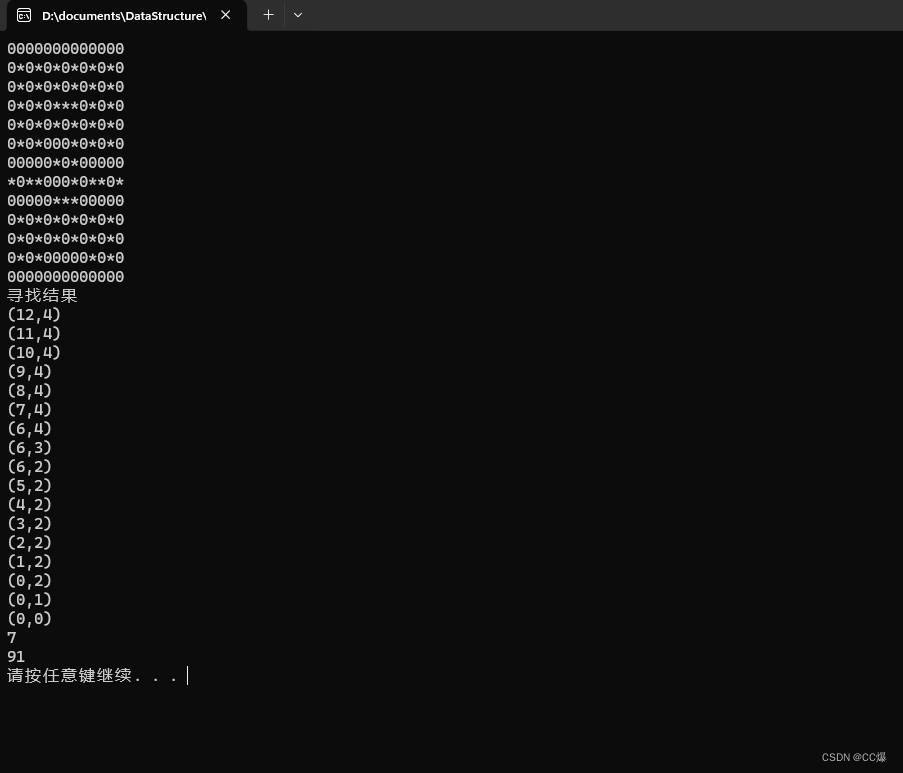
void AStarText() {
InitAstarMaze(&maps[0][0], 13, 13);
PrintMap(maps);
// 设置起始地址 和 终点
point* start = AllocPoint(12, 4);
point* end = AllocPoint(0, 0);
// A* 算法寻找路劲
list<point*> path = GetPath(start, end);
cout << "寻找结果" << endl;
list<point*>::const_iterator it;
for (it = path.begin(); it != path.end(); it++) {
point* cur = *it;
cout << '(' << cur->x << ',' << cur->y << ')' << endl;
//delete cur;
//it = path.erase(it);
Sleep(800);
}
ClearAstarMaze();
}
int main() {
AStarText();
system("pause");
return 0;
}
测试结果: