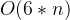A. Stair, Peak, or Neither?
题解:直接比较输出即可。
代码:
#include<bits/stdc++.h>
using namespace std ;
typedef long long ll ;
const int maxn = 2e5 + 7 ;
const int mod = 1e9 + 7 ;
inline ll read() {
ll x = 0, f = 1 ;
char c = getchar() ;
while (c > '9' || c < '0') {
if (c == '-')
f = -1 ;
c = getchar() ;
}
while (c >= '0' && c <= '9') {
x = x * 10 + c - '0' ;
c = getchar() ;
}
return x * f ;
}
char s[maxn] ;
ll t , n , d , k , a[maxn] , ans[maxn] ;
struct Node{
ll val , id ;
bool friend operator < (const Node a , const Node b){
return a.val < b.val ;
}
}b[maxn];
ll sum[maxn] ;
void solve(){
n = read() ;
d = read() ;
k = read() ;
if(n < d && d < k){
cout <<"STAIR" << endl ;
return ;
}
if(n < d && d > k){
cout << "PEAK" << endl ;
return ;
}
cout << "NONE" << endl ;
}
int main(){
t = read() ;
while(t --){
solve() ;
}
return 0 ;
}
B. Upscaling
题解:直接模拟输出即可。
代码:
#include<bits/stdc++.h>
using namespace std ;
typedef long long ll ;
const int maxn = 2e5 + 7 ;
const int mod = 1e9 + 7 ;
inline ll read() {
ll x = 0, f = 1 ;
char c = getchar() ;
while (c > '9' || c < '0') {
if (c == '-')
f = -1 ;
c = getchar() ;
}
while (c >= '0' && c <= '9') {
x = x * 10 + c - '0' ;
c = getchar() ;
}
return x * f ;
}
char s[maxn] ;
ll t , n , d , k , a[maxn] , ans[maxn] ;
struct Node{
ll val , id ;
bool friend operator < (const Node a , const Node b){
return a.val < b.val ;
}
}b[maxn];
ll sum[maxn] ;
void solve(){
n = read() ;
for(int i = 1 ; i <= n ; i ++){
if(i % 2 == 1){
bool f = 1 ;
for(int j = 1 ; j <= n ; j ++){
if(f == 1){
cout << "##" ;
f = 0 ;
}
else{
cout << ".." ;
f = 1 ;
}
}
cout << endl ;
f = 1 ;
for(int j = 1 ; j <= n ; j ++){
if(f == 1){
cout << "##" ;
f = 0 ;
}
else{
cout << ".." ;
f = 1 ;
}
}
cout << endl ;
}
else{
bool f = 0 ;
for(int j = 1 ; j <= n ; j ++){
if(f == 1){
cout << "##" ;
f = 0 ;
}
else{
cout << ".." ;
f = 1 ;
}
}
cout << endl ;
f = 0 ;
for(int j = 1 ; j <= n ; j ++){
if(f == 1){
cout << "##" ;
f = 0 ;
}
else{
cout << ".." ;
f = 1 ;
}
}
cout << endl ;
}
}
}
int main(){
t = read() ;
while(t --){
solve() ;
}
return 0 ;
}
C. Clock Conversion
题意:让你将二十四小时制转成十二小时制。
题解:多注意细节,判断输出即可。
代码:
#include<bits/stdc++.h>
using namespace std ;
typedef long long ll ;
const int maxn = 2e5 + 7 ;
const int mod = 1e9 + 7 ;
inline ll read() {
ll x = 0, f = 1 ;
char c = getchar() ;
while (c > '9' || c < '0') {
if (c == '-')
f = -1 ;
c = getchar() ;
}
while (c >= '0' && c <= '9') {
x = x * 10 + c - '0' ;
c = getchar() ;
}
return x * f ;
}
ll t , n , d , k , a[maxn] , ans[maxn] ;
struct Node{
ll val , id ;
bool friend operator < (const Node a , const Node b){
return a.val < b.val ;
}
}b[maxn];
ll sum[maxn] ;
char s[maxn] ;
void solve(){
scanf("%s" , s + 1) ;
if(s[1] == '0'){
if(s[2] == '0'){
cout << "12" << ":" << s[4] << s[5] << " " ;
cout << "AM" << endl ;
}
else{
cout << s[1] << s[2] << ":" << s[4] << s[5] << " " ;
cout << "AM" << endl ;
}
}
else{
ll sum = s[1] - '0' ;
sum = sum * 10 + (s[2] - '0') ;
if(sum < 12){
cout << s[1] << s[2] << ":" << s[4] << s[5] << " " << "AM" << endl ;
return ;
}
if(sum == 12){
cout << s[1] << s[2] << ":" << s[4] << s[5] << " " ;
cout << "PM" << endl ;
}
if(sum > 12){
if(sum - 12 < 10){
cout << 0 ;
}
cout << sum - 12 << ":" << s[4] << s[5] << " " ;
cout << "PM" << endl ;
}
}
}
int main(){
t = read() ;
while(t --){
solve() ;
}
return 0 ;
}
D. Product of Binary Decimals
题意:问给你一个数字,看能否将数字变成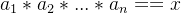 的形式,a数组必须保证是二进制数字。
的形式,a数组必须保证是二进制数字。
题解:看起来很复杂,但是你会发现,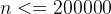 的数字,最多就有6位数字,那么很简单,直接枚举数字从n - 1 到 2,看看数字是否符合二进制数字的限制,如果符合并且能够整除n,那么就除尽为止,最后判断一下数字是否为二进制数字即可。复杂度
的数字,最多就有6位数字,那么很简单,直接枚举数字从n - 1 到 2,看看数字是否符合二进制数字的限制,如果符合并且能够整除n,那么就除尽为止,最后判断一下数字是否为二进制数字即可。复杂度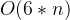
代码:
#include<bits/stdc++.h>
using namespace std ;
typedef long long ll ;
const int maxn = 2e5 + 7 ;
const int mod = 1e9 + 7 ;
inline ll read() {
ll x = 0, f = 1 ;
char c = getchar() ;
while (c > '9' || c < '0') {
if (c == '-')
f = -1 ;
c = getchar() ;
}
while (c >= '0' && c <= '9') {
x = x * 10 + c - '0' ;
c = getchar() ;
}
return x * f ;
}
ll t , n , d , k , a[maxn] , ans[maxn] ;
struct Node{
ll val , id ;
bool friend operator < (const Node a , const Node b){
return a.val < b.val ;
}
}b[maxn];
ll sum[maxn] ;
char s[maxn] ;
bool check(ll x){
while(x){
ll res = x % 10 ;
if(res == 0 || res == 1){
x /= 10 ;
}
else{
return 0 ;
}
}
return 1 ;
}
vector < ll > q ;
void solve(){
n = read() ;
ll N = n ;
if(check(n)){
cout << "YES\n" ;
return ;
}
for(auto it : q){
if(it < N){
while(N % it == 0){
N /= it ;
}
}
}
if(check(N)){
cout << "YES\n" ;
}
else{
cout << "NO\n" ;
}
}
int main(){
t = read() ;
for(ll i = 100000 ; i >= 2 ; i --){
if(check(i)){
q.push_back(i) ;
}
}
while(t --){
solve() ;
}
return 0 ;
}
E. Nearly Shortest Repeating Substring
题意:给你一个字符串n,能否找到最小的字符串k,将字符串k粘贴x遍,使长度和n相同,并且满足做多只有一个字符不同的最小字符串是什么?
题解:很明显,枚举因数存在set中,然后判断能否找到最多只有一个不同的字符串即可。
代码:
#include<bits/stdc++.h>
using namespace std ;
#define int long long
const int maxn = 2e5 + 7 ;
inline int read() {
int x = 0, f = 1 ;
char c = getchar() ;
while (c > '9' || c < '0') {
if (c == '-')
f = -1 ;
c = getchar() ;
}
while (c >= '0' && c <= '9') {
x = x * 10 + c - '0' ;
c = getchar() ;
}
return x * f ;
}
int n , t ;
string s ;
void solve(){
n = read() ;
cin >> s ;
set < int > st ;
for(int i = 1 ; i <= (int)sqrt(n) ; i ++){
if(n % i == 0){
st.insert(i) ;
st.insert(n / i) ;
}
}
int ans = n ;
for(auto it : st){
map < string , int > mp ;
for(int i = 0 ; i <= n - it ; i += it){
string x ;
for(int j = i ; j < i + it ; j ++){
x.push_back(s[j]) ;
}
mp[x]++ ;
}
if(mp.size() == 1){
ans = min(ans , it) ;
break ;
}
if(mp.size() == 2){
vector < string > zz ;
bool ok = 0 ;
for(auto [a , b] : mp){
zz.push_back(a) ;
if(b == 1)
ok = 1 ;
}
if(!ok)
continue ;
int cnt = 0 ;
for(int i = 0 ; i < it ; i++){
if(zz[0][i] != zz[1][i])
cnt ++ ;
}
if(cnt <= 1){
ans = min(ans , it) ;
break ;
}
}
}
cout << ans << endl ;
}
int main()
{
t = read() ;
while(t --){
solve() ;
}
}
F. 0, 1, 2, Tree!
题意:给你三个数字a,b,c,a表示有a个点有两个孩子,b表示有b个点有一个孩子,c表示有c个点有0个孩子,问能否构建符合条件的有根树(有根树是一个没有循环的连通图,有一个称为根的特殊顶点。在有根树中,在由一条边连接的任意两个顶点中,一个顶点是父顶点(靠近根的顶点),另一个顶点是子顶点。)。
题解:我们分情况讨论,首先先看为-1的情况,如果说一个树的根总数不等于c,那么一定无解。如果a为0,那么很明显如果c不等于1,那么一定无解。如果c等于1,那么答案显然就是b。那么如果a不为0,我们可以累加2的次方,看看加到2的第多少次幂会超出a,假设加到第i次幂大于等于a,如果是正好等于a,那么层数为i,否则层数为i + 1。然后再看最后一层一共有多少个孩子, 说正好等于a,那么孩子的数量就是2的i次方,如果不等于a,那么答案就是2的i + 1次方减去前面的总和加上2的i次方减去n,最后看b,如果前面累加不等于a,那么就先把上一层填满,再往下填即可。
注:整道题思维量比较大,代码很简单。希望大家能耐心读完!
代码:
#include<bits/stdc++.h>
using namespace std ;
typedef long long ll ;
const int maxn = 2e5 + 7 ;
const int mod = 1e9 + 7 ;
inline ll read() {
ll x = 0, f = 1 ;
char c = getchar() ;
while (c > '9' || c < '0') {
if (c == '-')
f = -1 ;
c = getchar() ;
}
while (c >= '0' && c <= '9') {
x = x * 10 + c - '0' ;
c = getchar() ;
}
return x * f ;
}
char s[maxn] ;
ll t , n , d , k , a[maxn] , ans[maxn] ;
struct Node{
ll val , id ;
bool friend operator < (const Node a , const Node b){
return a.val < b.val ;
}
}b[maxn];
ll Pow(ll x , ll y){
ll cnt = 1 ;
while(y){
if(y % 2)
cnt = cnt * x ;
x = x * x ;
y >>= 1 ;
}
return cnt ;
}
ll sum[maxn] ;
void solve(){
n = read() ;
d = read() ;
k = read() ;
if(n == 0){
if(k != 1){
cout << -1 << endl ;
return ;
}
else{
cout << d << endl ;
return ;
}
}
ll ans = 0 , sum = 0 , ceng = 1 , ress , rt ;
for(int i = 0 ; i <= 32 ; i ++){
ll xx = Pow(2 , (ll)i) ;
if(ans + xx <= n){
ans += xx ;
}
else{
if(ans == n){
sum = Pow(2 , (ll)i) ;
rt = 0 ;
ceng = i ;
}
else{
sum = Pow(2 , (ll)(i + 1)) - (ans + Pow(2 , (ll)i) - n) ;
rt = Pow(2 , (ll)(i)) - (n - ans) ;
ceng = i + 1 ;
}
break ;
}
}
// cout << rt << endl ;
if(sum != k){
cout << -1 << endl ;
return ;
}
else{
ll B = d ;
if(B - rt <= 0){
cout << ceng << endl ;
return ;
}
else{
B -= rt ;
while(B > 0){
B -= sum ;
ceng ++ ;
}
cout << ceng << endl ;
}
}
}
int main(){
t = read() ;
while(t --){
solve() ;
}
return 0 ;
}
如果喜欢作者的记得点赞收藏加关注哦~
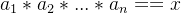 的形式,a数组必须保证是二进制数字。
的形式,a数组必须保证是二进制数字。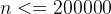 的数字,最多就有6位数字,那么很简单,直接枚举数字从n - 1 到 2,看看数字是否符合二进制数字的限制,如果符合并且能够整除n,那么就除尽为止,最后判断一下数字是否为二进制数字即可。复杂度
的数字,最多就有6位数字,那么很简单,直接枚举数字从n - 1 到 2,看看数字是否符合二进制数字的限制,如果符合并且能够整除n,那么就除尽为止,最后判断一下数字是否为二进制数字即可。复杂度