题目描述
已知有两个等长非降序序列 $S_1$和 $S_2$。先将 $S_1$和 $S_2$ 合并为 $S_3$ ，求 $S_3$的中位数。

输入描述

第一行，序列 $S_1$ 和 $S_2$ 的长度 $N$ ,
第二行，序列 $S_1$ 的 $N$ 个整数,
第三行，序列 $S_2$ 的 $N$ 个整数

输出描述

输出两个序列合并后序列 $S_3$ 的中位数。

思路分析

该题最优时间复杂度可以达到 $O(log(2\times N))$ ，但从暴力的层面时间复杂度是 $O(2\ast N)$ ，因此所有用 sort 排序输出的都是傻瓜。

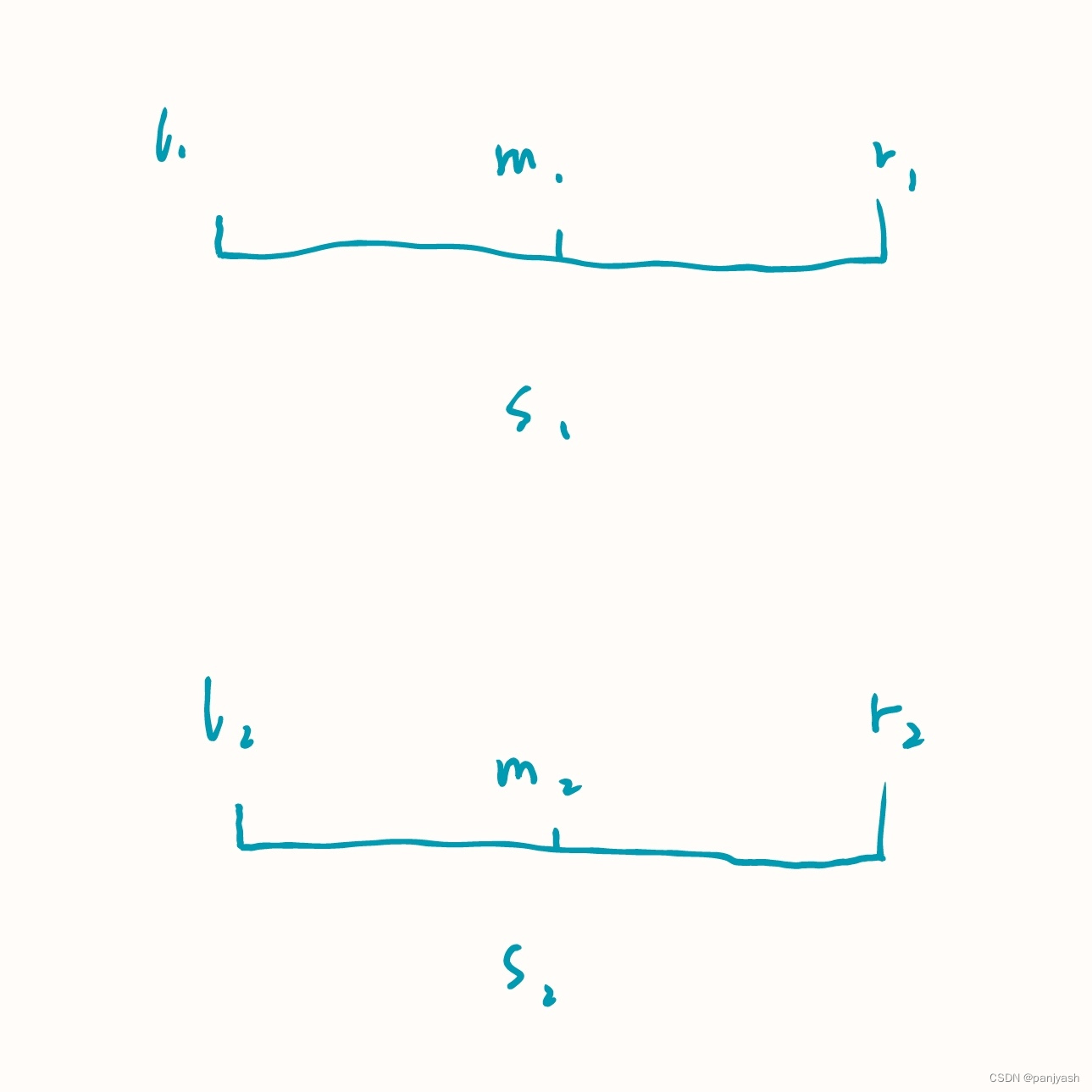
首先我们对 $S_1$ 和 $S_2$ 取一下中位数，当 $S_1$ 的中位数小于 $S_2$ 的中位数时，那么 $S_1$ 中小于 ${S_1}_{m1}$ 一定小于 ${S_2}_{m2}$ ，即中位数不会出现在 ${S_1}_{1-(m1-1)}$ 之中。同理，中位数也不会出现在 ${S_2}_{(m2+1-r2)}$中。由此我们可以将这两部分删去，重新找新区间的中位数即可。如图

需要注意的是，我们递归过程中的出口是什么。一个坑点是合并以后的数集个数一定是偶数，因此我们需要找到 $S_3$ 中的第 $N$ 大的数和第 $N+1$ 大的数。如果我们把出口定在 $l_1==r_1$ 的话就会出现对于两个中位数同时在 $S_1$ 或 $S_2$ 中时答案的误判，因此我们把出口放在 $l_1+1==r_1$ 找一下中位数即可。

代码

#include <bits/stdc++.h>
using namespace std;

#define all(x) x.begin(), x.end()
#define bit1(x) __builtin_popcount(x)
#define Pqueue priority_queue
#define lc p << 1
#define rc p << 1 | 1
#define IOS ios::sync_with_stdio(false), cin.tie(0);
#define fi first
#define se second

typedef long long ll;
typedef long double ld;
typedef unsigned long long ull;
typedef pair<ll, ll> PII;

const ll mod = 1000000007;
const ll N = 1e6 + 10;
const ld eps = 1e-9;
const ll inf = 1e18;
const ll P = 131;
const ll dir[8][2] = {1, 0, 0, 1, -1, 0, 0, -1, 1, 1, 1, -1, -1, 1, -1, -1};

int n;
int s1[N], s2[N];

void merge(int l1, int r1, int l2, int r2)
{
    if (l1 + 1 == r1)
    {
        int a, b;
        if (s1[r1] >= s2[r2])
        {
            a = s2[r2];
            if (s1[l1] <= s2[l2])
                b = s2[l2];
            else
                b = s1[l1];
        }
        else
        {
            a = s1[r1];
            if (s1[l1] <= s2[l2])
                b = s2[l2];
            else
                b = s1[l1];
        }
        cout << (a + b) / 2;
        return;
    }
    int m1 = l1 + r1 >> 1, m2 = l2 + r2 >> 1;
    if (s1[m1] > s2[m2])
        merge(l1, m1, m2, r2);
    else
        merge(m1, r1, l2, m2);
}

void solve()
{
    cin >> n;
    for (int i = 1; i <= n; i++)
        cin >> s1[i];
    for (int i = 1; i <= n; i++)
        cin >> s2[i];
    if (n == 1)
        return void(cout << (s1[1] + s2[1]) / 2); //对长度为1的数集的特判
    merge(1, n, 1, n);
}

int main()
{
    int T = 1;
    // cin>>T;
    while (T--)
        solve();
    return 0;
}

/*
oxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxox
x                                                                                      o
o       _/_/_/_/                                                              _/       x
x      _/                                                                              o
o     _/_/_/_/ _/  _/_/   _/_/   _/_/_/ _/_/   _/_/_/     _/_/    _/_/_/    _/ _/   _/ x
x    _/       _/_/     _/    _/ _/   _/   _/  _/    _/ _/    _/  _/    _/  _/   _/ _/  o
o   _/       _/       _/    _/ _/   _/   _/  _/    _/ _/    _/  _/    _/  _/    _/_/   x
x  _/       _/         _/_/   _/   _/   _/  _/_/_/     _/_/ _/ _/    _/  _/      _/    o
o                                          _/                           _/      _/     x
x                                         _/                        _/_/       _/      o
o                                                                                      x
xoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxoxo
*/