2024-2-26

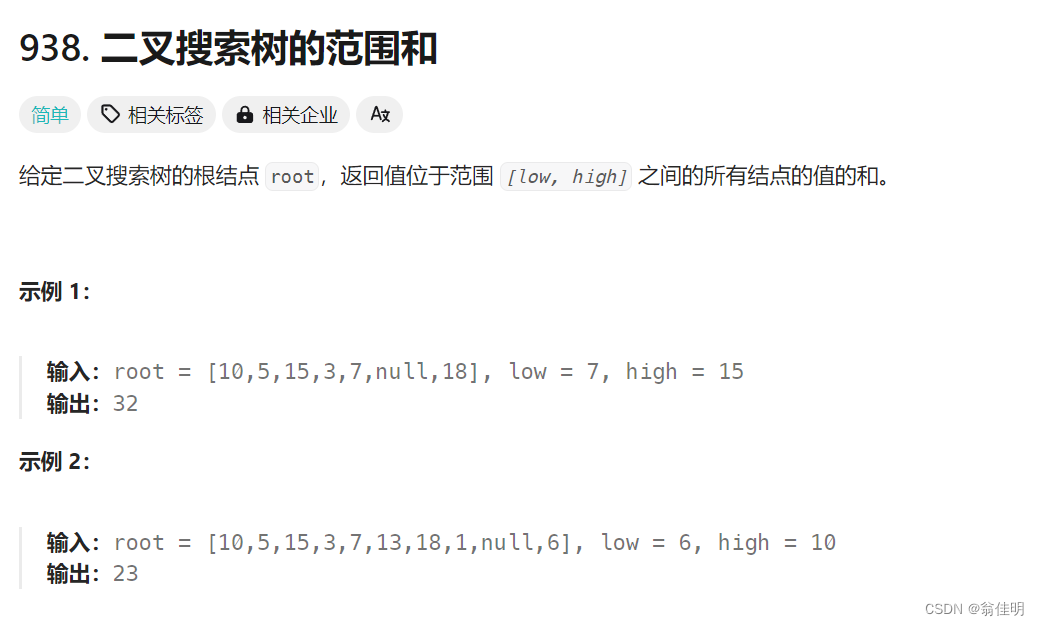
938. 二叉搜索树的范围和

思路：

1.在二叉搜索树中：左子树的结点都小于根节点，右子树的结点都大于根节点，每个结点法左右子树均要符合二叉搜索树

2.如果根节点的值大于hight，就排除右树，只在左树中查找

3.如果根节点的值小于low，就排除左树，只在右树中查找

4.如果正好在两者之间，则左树右树都要考虑，将根节点的值和递归的结果累加

写法一：在中间累加

    //[938. 二叉搜索树的范围和]-写法二
//
    public int rangeSumBST2(TreeNode root, int low, int high) {
        if (root==null){
            return 0;
        }
        int x = root.val;
        int sum = low<=x && x<=high?x:0;
        //根节点的值如果在范围内，返回根节点的值，否则就返回0，相当于排除了不在范围内的值
        if(x>low){
            sum+=rangeSumBST2(root.left,low,high);
        }
        if (x<high){
            sum+=rangeSumBST2(root.right,low,high);
        }
        return sum;
    }

写法二：在最后累加

    //[938. 二叉搜索树的范围和]
    public int rangeSumBST(TreeNode root, int low, int high) {
        if (root == null) {
            return 0;
        }
        int x = root.val;
        if (x > high) {
            return rangeSumBST(root.left, low, high);
            //右树不在范围内，只需要递归左树
        }
        if (x < low) {
            return rangeSumBST(root.right, low, high);
            //左树不在范围内，只需要递归左树
        }
        return x + rangeSumBST(root.left, low, high) + rangeSumBST(root.right, low, high);
        //左右子树都可能
    }