目录
- 2.7 优先级队列
- 1) 无序数组实现
- 2) 有序数组实现
- 3) 堆实现
- 习题
- E01. 合并多个有序链表-Leetcode 23

2.7 优先级队列
1) 无序数组实现
要点
- 入队保持顺序
- 出队前找到优先级最高的出队,相当于一次选择排序
public class PriorityQueue1<E extends Priority> implements Queue<E> {
Priority[] array;
int size;
public PriorityQueue1(int capacity) {
array = new Priority[capacity];
}
@Override // O(1)
public boolean offer(E e) {
if (isFull()) {
return false;
}
array[size++] = e;
return true;
}
// 返回优先级最高的索引值
private int selectMax() {
int max = 0;
for (int i = 1; i < size; i++) {
if (array[i].priority() > array[max].priority()) {
max = i;
}
}
return max;
}
@Override // O(n)
public E poll() {
if (isEmpty()) {
return null;
}
int max = selectMax();
E e = (E) array[max];
remove(max);
return e;
}
private void remove(int index) {
if (index < size - 1) {
System.arraycopy(array, index + 1,
array, index, size - 1 - index);
}
array[--size] = null; // help GC
}
@Override
public E peek() {
if (isEmpty()) {
return null;
}
int max = selectMax();
return (E) array[max];
}
@Override
public boolean isEmpty() {
return size == 0;
}
@Override
public boolean isFull() {
return size == array.length;
}
}
- 视频中忘记了 help GC,注意一下
2) 有序数组实现
要点
- 入队后排好序,优先级最高的排列在尾部
- 出队只需删除尾部元素即可
public class PriorityQueue2<E extends Priority> implements Queue<E> {
Priority[] array;
int size;
public PriorityQueue2(int capacity) {
array = new Priority[capacity];
}
// O(n)
@Override
public boolean offer(E e) {
if (isFull()) {
return false;
}
insert(e);
size++;
return true;
}
// 一轮插入排序
private void insert(E e) {
int i = size - 1;
while (i >= 0 && array[i].priority() > e.priority()) {
array[i + 1] = array[i];
i--;
}
array[i + 1] = e;
}
// O(1)
@Override
public E poll() {
if (isEmpty()) {
return null;
}
E e = (E) array[size - 1];
array[--size] = null; // help GC
return e;
}
@Override
public E peek() {
if (isEmpty()) {
return null;
}
return (E) array[size - 1];
}
@Override
public boolean isEmpty() {
return size == 0;
}
@Override
public boolean isFull() {
return size == array.length;
}
}
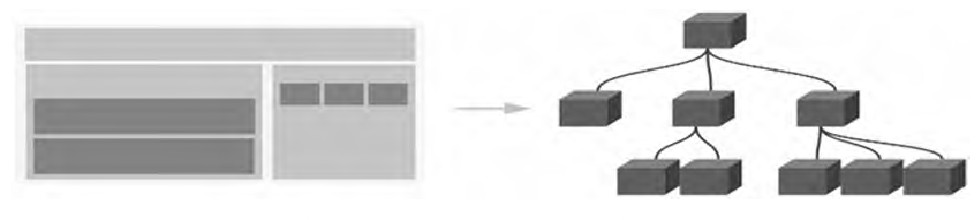
3) 堆实现
计算机科学中,堆是一种基于树的数据结构,通常用完全二叉树实现。堆的特性如下
- 在大顶堆中,任意节点 C 与它的父节点 P 符合 P . v a l u e ≥ C . v a l u e P.value \geq C.value P.value≥C.value
- 而小顶堆中,任意节点 C 与它的父节点 P 符合 P . v a l u e ≤ C . v a l u e P.value \leq C.value P.value≤C.value
- 最顶层的节点(没有父亲)称之为 root 根节点
In computer science, a heap is a specialized tree-based data structure which is essentially an almost complete tree that satisfies the heap property: in a max heap, for any given node C, if P is a parent node of C, then the key (the value) of P is greater than or equal to the key of C. In a min heap, the key of P is less than or equal to the key of C. The node at the “top” of the heap (with no parents) is called the root node
例1 - 满二叉树(Full Binary Tree)特点:每一层都是填满的
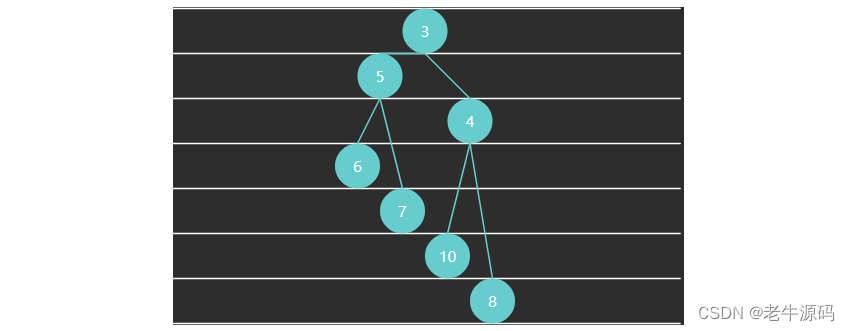
例2 - 完全二叉树(Complete Binary Tree)特点:最后一层可能未填满,靠左对齐
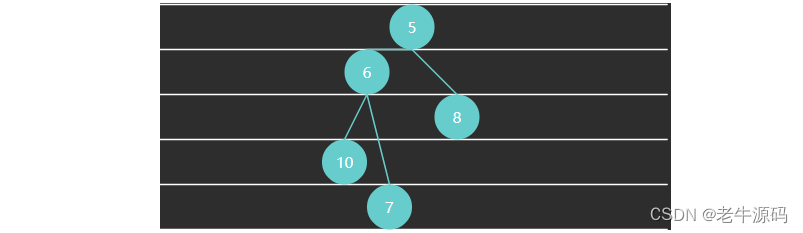
例3 - 大顶堆
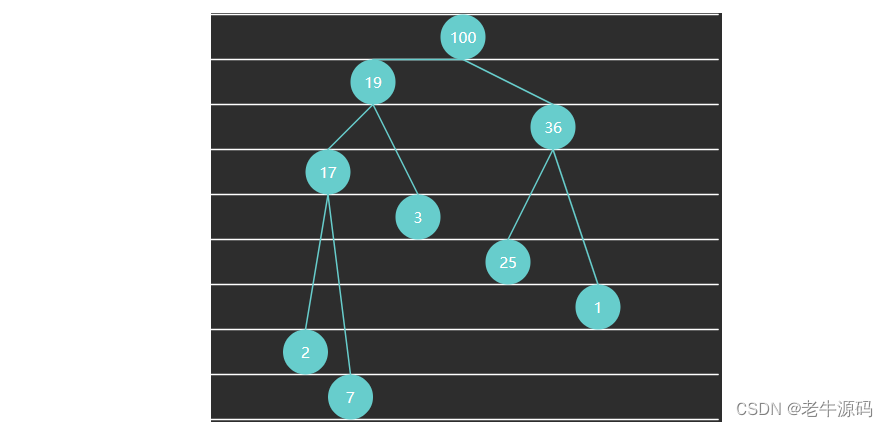
例4 - 小顶堆
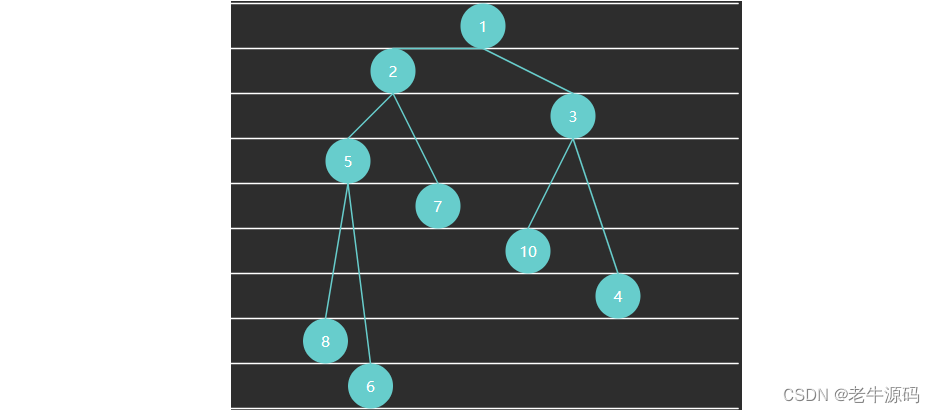
完全二叉树可以使用数组来表示
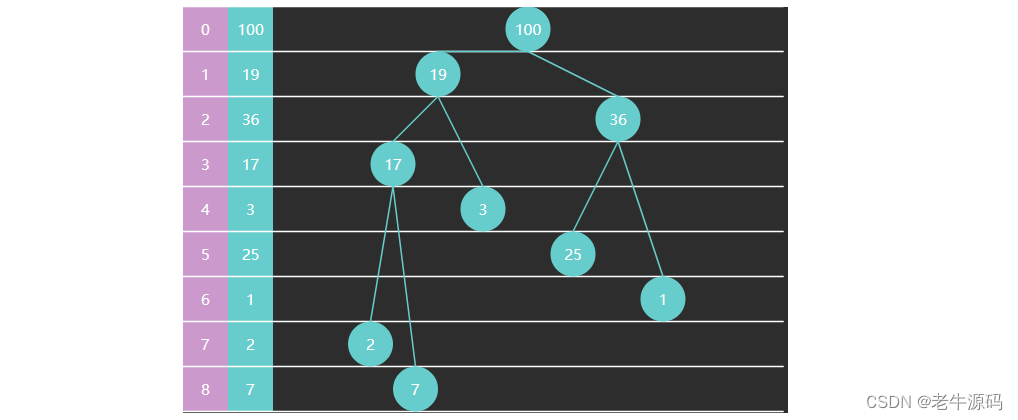
特征
- 如果从索引 0 开始存储节点数据
- 节点 i i i 的父节点为 f l o o r ( ( i − 1 ) / 2 ) floor((i-1)/2) floor((i−1)/2),当 i > 0 i>0 i>0 时
- 节点 i i i 的左子节点为 2 i + 1 2i+1 2i+1,右子节点为 2 i + 2 2i+2 2i+2,当然它们得 < s i z e < size <size
- 如果从索引 1 开始存储节点数据
- 节点 i i i 的父节点为 f l o o r ( i / 2 ) floor(i/2) floor(i/2),当 i > 1 i > 1 i>1 时
- 节点 i i i 的左子节点为 2 i 2i 2i,右子节点为 2 i + 1 2i+1 2i+1,同样得 < s i z e < size <size
代码
public class PriorityQueue4<E extends Priority> implements Queue<E> {
Priority[] array;
int size;
public PriorityQueue4(int capacity) {
array = new Priority[capacity];
}
@Override
public boolean offer(E offered) {
if (isFull()) {
return false;
}
int child = size++;
int parent = (child - 1) / 2;
while (child > 0 && offered.priority() > array[parent].priority()) {
array[child] = array[parent];
child = parent;
parent = (child - 1) / 2;
}
array[child] = offered;
return true;
}
private void swap(int i, int j) {
Priority t = array[i];
array[i] = array[j];
array[j] = t;
}
@Override
public E poll() {
if (isEmpty()) {
return null;
}
swap(0, size - 1);
size--;
Priority e = array[size];
array[size] = null;
shiftDown(0);
return (E) e;
}
void shiftDown(int parent) {
int left = 2 * parent + 1;
int right = left + 1;
int max = parent;
if (left < size && array[left].priority() > array[max].priority()) {
max = left;
}
if (right < size && array[right].priority() > array[max].priority()) {
max = right;
}
if (max != parent) {
swap(max, parent);
shiftDown(max);
}
}
@Override
public E peek() {
if (isEmpty()) {
return null;
}
return (E) array[0];
}
@Override
public boolean isEmpty() {
return size == 0;
}
@Override
public boolean isFull() {
return size == array.length;
}
}
习题
E01. 合并多个有序链表-Leetcode 23
这道题目之前解答过,现在用刚学的优先级队列来实现一下
题目中要从小到大排列,因此选择用小顶堆来实现,自定义小顶堆如下
public class MinHeap {
ListNode[] array;
int size;
public MinHeap(int capacity) {
array = new ListNode[capacity];
}
public void offer(ListNode offered) {
int child = size++;
int parent = (child - 1) / 2;
while (child > 0 && offered.val < array[parent].val) {
array[child] = array[parent];
child = parent;
parent = (child - 1) / 2;
}
array[child] = offered;
}
public ListNode poll() {
if (isEmpty()) {
return null;
}
swap(0, size - 1);
size--;
ListNode e = array[size];
array[size] = null; // help GC
down(0);
return e;
}
private void down(int parent) {
int left = 2 * parent + 1;
int right = left + 1;
int min = parent;
if (left < size && array[left].val < array[min].val) {
min = left;
}
if (right < size && array[right].val < array[min].val) {
min = right;
}
if (min != parent) {
swap(min, parent);
down(min);
}
}
private void swap(int i, int j) {
ListNode t = array[i];
array[i] = array[j];
array[j] = t;
}
public boolean isEmpty() {
return size == 0;
}
}
代码
public class E01Leetcode23 {
public ListNode mergeKLists(ListNode[] lists) {
// 1. 使用 jdk 的优先级队列实现
// PriorityQueue<ListNode> queue = new PriorityQueue<>(Comparator.comparingInt(a -> a.val));
// 2. 使用自定义小顶堆实现
MinHeap queue = new MinHeap(lists.length);
for (ListNode head : lists) {
if (head != null) {
queue.offer(head);
}
}
ListNode s = new ListNode(-1, null);
ListNode p = s;
while (!queue.isEmpty()) {
ListNode node = queue.poll();
p.next = node;
p = node;
if (node.next != null) {
queue.offer(node.next);
}
}
return s.next;
}
}
提问:
- 能否将每个链表的所有元素全部加入堆,再一个个从堆顶移除?
回答:
- 可以是可以,但对空间占用就高了,堆的一个优点就是用有限的空间做事情