- 93.复原IP地址

class Solution { List<String> result = new ArrayList<>(); public List<String> restoreIpAddresses(String s) { StringBuilder sb = new StringBuilder(s); backTracking(sb, 0, 0); return result; } private void backTracking(StringBuilder s, int startIndex, int dotCount){ if(dotCount == 3){ if(isValid(s, startIndex, s.length() - 1)){ result.add(s.toString()); } return; } for(int i = startIndex; i < s.length(); i++){ if(isValid(s, startIndex, i)){ s.insert(i + 1, '.'); backTracking(s, i + 2, dotCount + 1); s.deleteCharAt(i + 1); }else{ break; } } } //[start, end] private boolean isValid(StringBuilder s, int start, int end){ if(start > end) return false; if(s.charAt(start) == '0' && start != end) return false; int num = 0; for(int i = start; i <= end; i++){ int digit = s.charAt(i) - '0'; num = num * 10 + digit; if(num > 255) return false; } return true; } }思路:与day27的分割回文子串类似,主要是要理解isVaild的思路,当dotCount == 3时,还要进行判断,然后将符合的加入result中
- 78.子集

class Solution { List<List<Integer>> result = new ArrayList<>();// 存放符合条件结果的集合 LinkedList<Integer> path = new LinkedList<>();// 用来存放符合条件结果 public List<List<Integer>> subsets(int[] nums) { subsetsHelper(nums, 0); return result; } private void subsetsHelper(int[] nums, int startIndex){ result.add(new ArrayList<>(path));//「遍历这个树的时候,把所有节点都记录下来,就是要求的子集集合」。 if (startIndex >= nums.length){ //终止条件可不加 return; } for (int i = startIndex; i < nums.length; i++){ path.add(nums[i]); subsetsHelper(nums, i + 1); path.removeLast(); } } }思路:和分割问题类似,主要区别是要在每个节点收获结果,所以result.add(new ArrayList<>(path)要放在最上面。
- 90.子集II

class Solution { List<List<Integer>> result = new ArrayList<>();// 存放符合条件结果的集合 LinkedList<Integer> path = new LinkedList<>();// 用来存放符合条件结果 boolean[] used; public List<List<Integer>> subsetsWithDup(int[] nums) { if (nums.length == 0){ result.add(path); return result; } Arrays.sort(nums); used = new boolean[nums.length]; subsetsWithDupHelper(nums, 0); return result; } private void subsetsWithDupHelper(int[] nums, int startIndex){ result.add(new ArrayList<>(path)); if (startIndex >= nums.length){ return; } for (int i = startIndex; i < nums.length; i++){ if (i > 0 && nums[i] == nums[i - 1] && !used[i - 1]){ continue; } path.add(nums[i]); used[i] = true; subsetsWithDupHelper(nums, i + 1); path.removeLast(); used[i] = false; } } }思路:和 40.组合总和II方法一样,都是要进行树层去重。关键是used数组的使用,要确保used[i-1]==false;然后就是每个节点都收获结果。
代码随想录算法训练营29期|day28 任务以及具体安排
本文来自互联网用户投稿,该文观点仅代表作者本人,不代表本站立场。本站仅提供信息存储空间服务,不拥有所有权,不承担相关法律责任。如若转载,请注明出处:/a/342822.html
如若内容造成侵权/违法违规/事实不符,请联系我们进行投诉反馈qq邮箱809451989@qq.com,一经查实,立即删除!相关文章
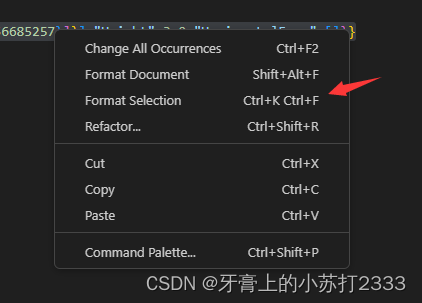
VS Code Json格式化插件-JSON formatter
🦪整个文件格式化 按快捷键Shift Alt F 🥪仅格式化选择内容 需要选择完整的json段落即:{} 或 [] 括起来的部分,再按快捷键Ctrl K F
高分辨率图像合成;可控运动合成;虚拟试衣;在FPGA上高效运行二值Transformer
本文首发于公众号:机器感知
高分辨率图像合成;可控运动合成;虚拟试衣;在FPGA上高效运行二值Transformer
Scalable High-Resolution Pixel-Space Image Synthesis with Hourglass Diffusion Transformers We present the Hourgla…
蓝桥杯真题(Python)每日练Day4
题目
OJ编号2117
题目分析
第一种先采用暴力的思想,从第一根竹子开始,找到连续的高度相同的竹子,砍掉这些竹子,一直循环这个方法,直到所有的竹子高度都为1。很明显,依次遍历竹子的高度复杂度为O&#x…
jdk的安装和Tomcat的安装
jdk的安装
双击jdk,然后一路下一步 公共JRE可以关闭,没多大用,反而会占用内存
计算机–>属性–>高级系统设置–>环境变量 系统变量–新建 JAVA_HOMEjdk的存放路径 修改path 在path的最后面添加(;%JAVA_H…
SpringBoot整合SSE
目录 1.SseController2. SseServiceSseServiceSseServiceImpl 3.SendMessageTask4.将定时任务加入启动类5.参考资料 1.SseController Slf4j
RestController
RequestMapping("sse")
public class SseController {Autowiredprivate SseService sseService;RequestMappi…
cesium应用篇:静态图添加与平移、缩放
应用说明
添加静态图到指定extent通过键盘事件对static image进行调整 向东、西、南、北移动沿东西、南北方向缩放命令行侧输出当前extent,可用于几何匹配
初始化
Earth 初始化
<!DOCTYPE html>
<html lang"en">
<head><meta c…
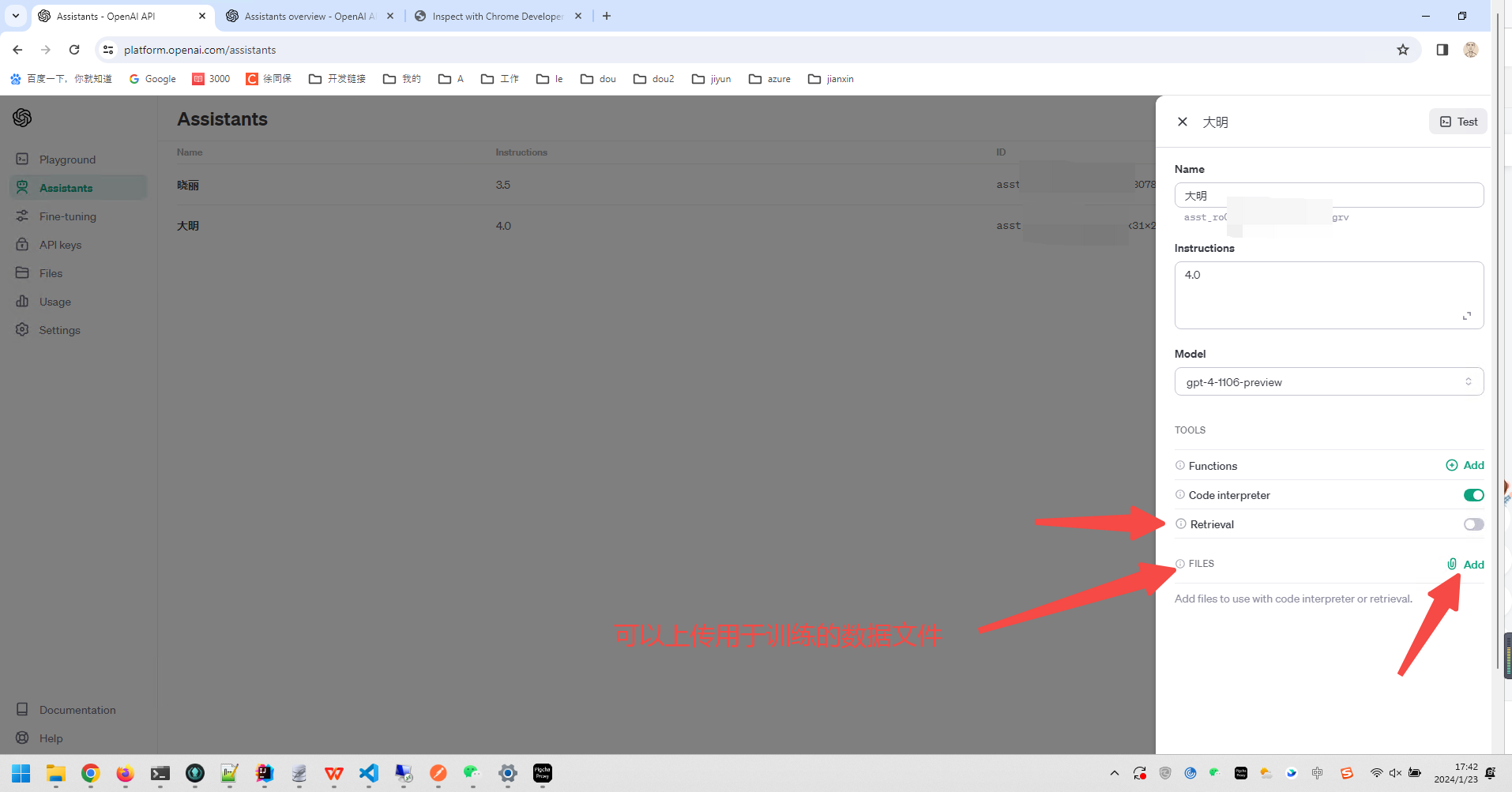
openai assistants api接入微信机器人,实现类GPTs功能
chatgpt网址:https://chat.xutongbao.top 比普通gpt多了代码解释器功能,和上传训练数据文件的功能,这两个功能就是GPTs拥有的,而普通gpt没有拥有的
前后端分离项目中实现图形验证码
图形验证码在我们的日常生活中时经常用到的,一般用于用户的登录、注册等。
图形验证码在互联网应用中的作用是提高安全性、防止滥用和保护用户隐私。它是一种简单而有效的人机验证技术,帮助保护系统和用户免受自动化攻击的影响。
本次我们通过spring b…
Qt采集本地摄像头推流成rtsp/rtmp(可网页播放/支持嵌入式linux)
一、功能特点
支持各种本地视频文件和网络视频文件。支持各种网络视频流,网络摄像头,协议包括rtsp、rtmp、http。支持将本地摄像头设备推流,可指定分辨率和帧率等。支持将本地桌面推流,可指定屏幕区域和帧率等。自动启动流媒体服…
华为认证的HCIP考实验考试么?
HCIP在考试的时候不考实验,只考笔试,只是不同方向的HCIP认证考试的考试科目不同,有的考一科,有的考二科,有的考三科,具体看方向来定。HCIA和HCIP只考笔试。HCIE考笔试和实验。
虽然HCIP不考实操࿰…
MongoDB快速入门
目录
什么是MongoDB?
MongoDB与关系型数据库对比
MongoDB应用场景
MongoDB语法
数据库操作
集合操作
安全认证
MongoDB插入指令
MongoDB查询指令
MongoDB更新指令
MongoDB删除指令
内嵌文档和数组
嵌套型的数组
固定(封顶)集合…
线程的同步和互斥学习笔记
目录
互斥锁的概念和使用
线程通信-互斥 互斥锁的创建和销毁 申请锁-pthread_mutex_lock 释放锁-pthread_mutex_unlock
读写锁的概念和使用
死锁的避免 互斥锁的概念和使用
线程通信-互斥
临界资源
一次只允许一个任务(进程、线程)访问的共享资…
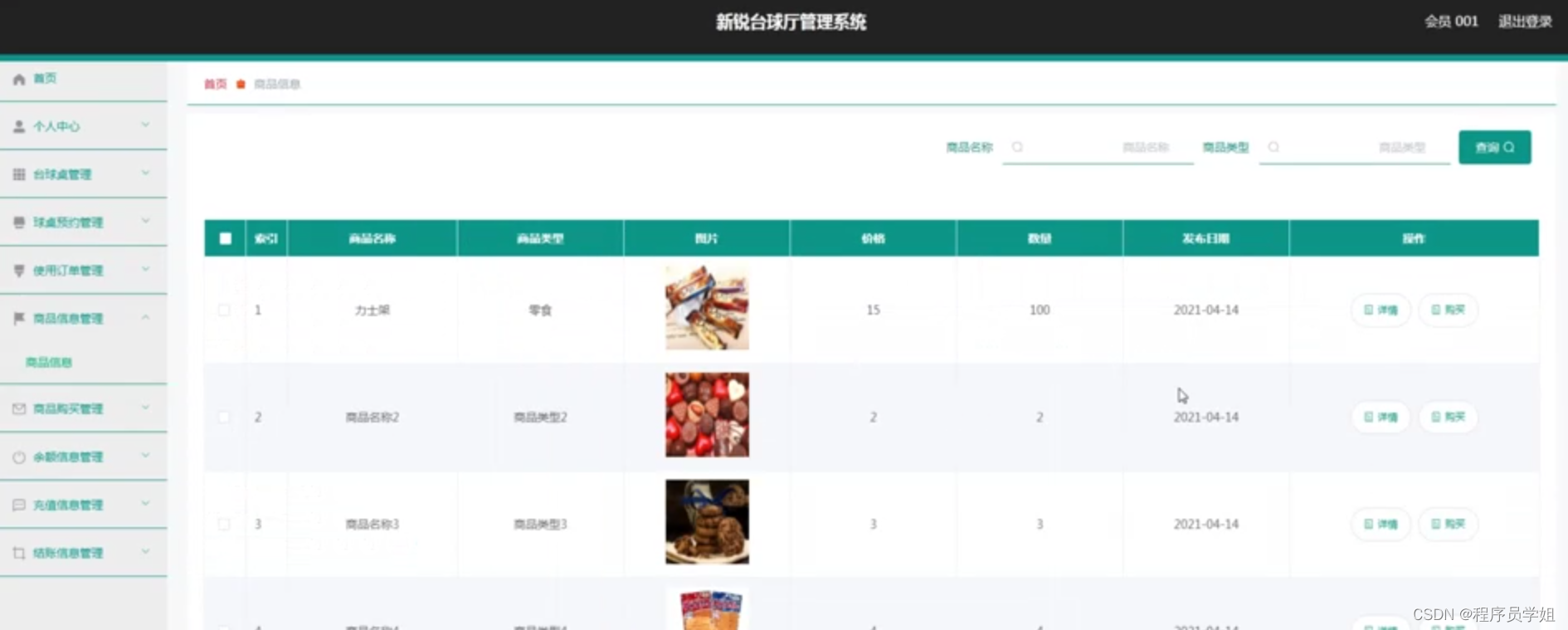
基于springboot+vue的台球管理系统
摘要 台球管理系统是一款基于Spring Boot和Vue.js技术栈构建的现代化系统,旨在提供全面而高效的台球场馆管理服务。该系统通过整合前后端技术,实现了场馆预约、会员管理、比赛统计等核心功能,为台球场馆管理员和玩家提供了便捷、智能的管理和…
leetcode第 381 场周赛最后一题 差分,对称的处理
第 381 场周赛 - 力扣(LeetCode)最后一题3017. 按距离统计房屋对数目 II - 力扣(LeetCode)
dijkstra超时了,看了灵神的解题方法力扣(LeetCode)官网 - 全球极客挚爱的技术成长平台,其…
Linux中普通用户如何使用sudo指令提升权限
提示:文章写完后,目录可以自动生成,如何生成可参考右边的帮助文档 目录
前言
普通用户为何无法使用sudo?
我们来看一下具体操作
总结 前言
世上有两种耀眼的光芒,一种是正在升起的太阳,一种是正在努力…
Facebook的区块链之路:探秘数字货币的未来
近年来,Facebook一直在积极探索区块链技术,并逐渐将目光聚焦在数字货币领域。从推出Libra项目到改名为Diem,Facebook一直在寻求在数字货币领域取得突破性进展。本文将深入探讨Facebook的区块链之路,揭示其对数字货币未来发展的影响…
数字拆分--完全背包问题
一、题目
https://acm.ecnu.edu.cn/problem/3034/
二、思路
本来算法就很弱,加上很久没刷题,做这道题真的是一言难尽~
一开始我以为是找规律写递推式,写到f(9)的时候就觉得不对劲,又想了一会,还是没想到࿰…
【linux】Linux编译器-gcc/g++使用
先写一段代码演示 1 #include<stdio.h>2 #define M 1003 int main()4 {5 printf("hello linux");6 printf("hello linux");7 //printf("hello linux");8 //printf("hello linux");9 //printf("hello linux");10 //pri…
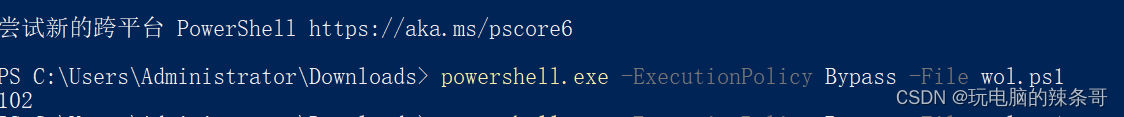
Win10 如何用powershell写个WOL开机脚本
环境:
Win10 专业版
问题描述:
Win10 如何用powershell写个WOL开机脚本
解决方案:
1.脚本内容
$mac b1-10-18-52-11-12
$macBytes $mac -split - | ForEach-Object { [byte](0x $_) }
$broadcastAddress [byte[]](1..6 | ForEach-O…
最新文章
- SpringSecurity初始化的本质
- 【大模型系列篇】DeepSeek-R1如何通过强化学习有效提升大型语言模型的推理能力?
- 无缝对接[系列2]:在VSCode中继续接入本地DeepSeek的完整指南---实现代码协助编写~
- 企业内部真题
- python组备赛笔记(基础篇)
- linux5-多任务--进程fork()
- 【张雪峰高考志愿填报】合集
- 【JS重点知识04】JS执行机制(重点面试题)
- 最新仿抖音短视频开源版+商城+短视频
- # 深入解析C/C++中的关键运算符:i++, ++i, a=i++, a=++i 及复杂表达式的运算顺序
- ##06 神经网络训练基础:一步步构建和完善你的第一个模型
- #LLM入门|Prompt#1.9_总结_Summary