文章目录
- 一【题目类别】
- 二【题目难度】
- 三【题目编号】
- 四【题目描述】
- 五【题目示例】
- 六【解题思路】
- 七【时间频度】
- 八【代码实现】
- 九【提交结果】
一【题目类别】
- 双指针
二【题目难度】
- 困难
三【题目编号】
- 面试题17.21.直方图的水量
四【题目描述】
- 给定一个直方图(也称柱状图),假设有人从上面源源不断地倒水,最后直方图能存多少水量?直方图的宽度为 1。
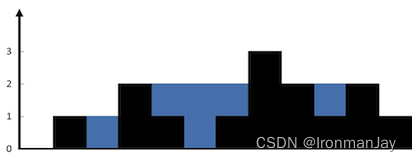
- 上面是由数组 [0,1,0,2,1,0,1,3,2,1,2,1] 表示的直方图,在这种情况下,可以接 6 个单位的水(蓝色部分表示水)。 感谢 Marcos 贡献此图。
五【题目示例】
- 示例:
- 输入: [0,1,0,2,1,0,1,3,2,1,2,1]
- 输出: 6
六【解题思路】
- 本题是双指针的经典应用,解题思路也有一些技巧,我们需要利用木桶的短板效应:一个木桶盛水的多少,由木桶中最短的板子决定
- 所以我们使用leftMax记录左边最长的板子,使用rightMax记录右边最长的板子,使用left记录左边的数组下标,使用right记录右边的数组下标
- 对于任意一个位置,只要右边有比左边高的板子,不管中间是什么情况,此位置能存的雨水量,之和最左边的板子相关
- 对于任意一个位置,只要左边有比右边高的板子,不管中间是什么情况,此位置能存的雨水量,之和最右边的板子相关
- 基于这个思路,遍历整个数组,不停的更新左边最高的板子和右边最高的板子,并求和每个位置可以存的雨水量
- 最后返回结果即可
七【时间频度】
- 时间复杂度: O ( n ) O(n) O(n),其中 n n n为传入的数组的长度
- 空间复杂度: O ( 1 ) O(1) O(1)
八【代码实现】
- Java语言版
class Solution {
public int trap(int[] height) {
int res = 0;
int left = 0;
int right = height.length - 1;
int leftMax = 0;
int rightMax = 0;
while(left <= right){
if(leftMax < rightMax){
res += Math.max(0,leftMax - height[left]);
leftMax = Math.max(leftMax,height[left]);
left++;
}else{
res += Math.max(0,rightMax - height[right]);
rightMax = Math.max(rightMax,height[right]);
right--;
}
}
return res;
}
}
- C语言版
int trap(int* height, int heightSize)
{
int res = 0;
int left = 0;
int right = heightSize - 1;
int leftMax = 0;
int rightMax = 0;
while(left <= right)
{
if(leftMax < rightMax)
{
res += fmax(0,leftMax - height[left]);
leftMax = fmax(leftMax,height[left]);
left++;
}
else
{
res += fmax(0,rightMax - height[right]);
rightMax = fmax(rightMax,height[right]);
right--;
}
}
return res;
}
- Python语言版
class Solution:
def trap(self, height: List[int]) -> int:
res = 0
left = 0
right = len(height) - 1
leftMax = 0
rightMax = 0
while left <= right:
if leftMax < rightMax:
res += max(0,leftMax-height[left])
leftMax = max(leftMax,height[left])
left+=1
else:
res += max(0,rightMax-height[right])
rightMax = max(rightMax,height[right])
right-=1
return res
- C++语言版
class Solution {
public:
int trap(vector<int>& height) {
int res = 0;
int left = 0;
int right = height.size() - 1;
int leftMax = 0;
int rightMax = 0;
while(left <= right)
{
if(leftMax < rightMax)
{
res += max(0,leftMax - height[left]);
leftMax = max(leftMax,height[left]);
left++;
}
else
{
res += max(0,rightMax - height[right]);
rightMax = max(rightMax,height[right]);
right--;
}
}
return res;
}
};
九【提交结果】
-
Java语言版

-
C语言版
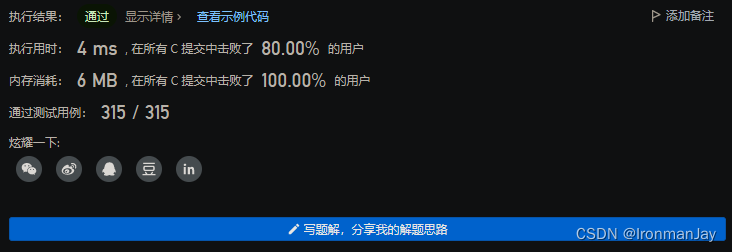
-
Python语言版
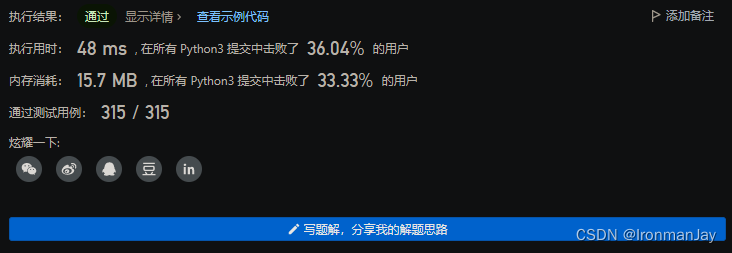
-
C++语言版
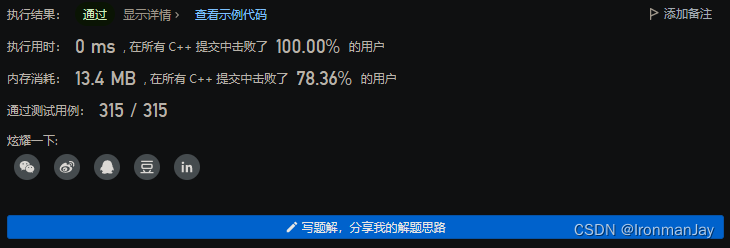


![springcloud3 nacos,sentinel,ribbon,openfegin等整合案例4[fallback+blockhandler完美整合]](https://img-blog.csdnimg.cn/68b8ff40e2554bf9bf56a10ec963a992.png)