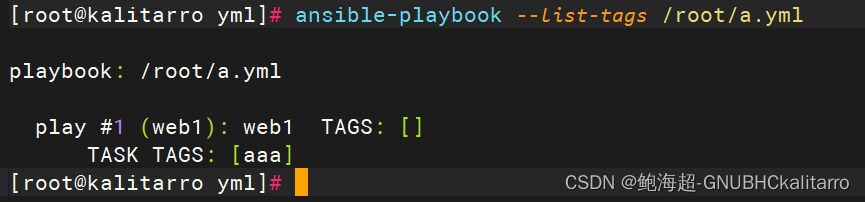
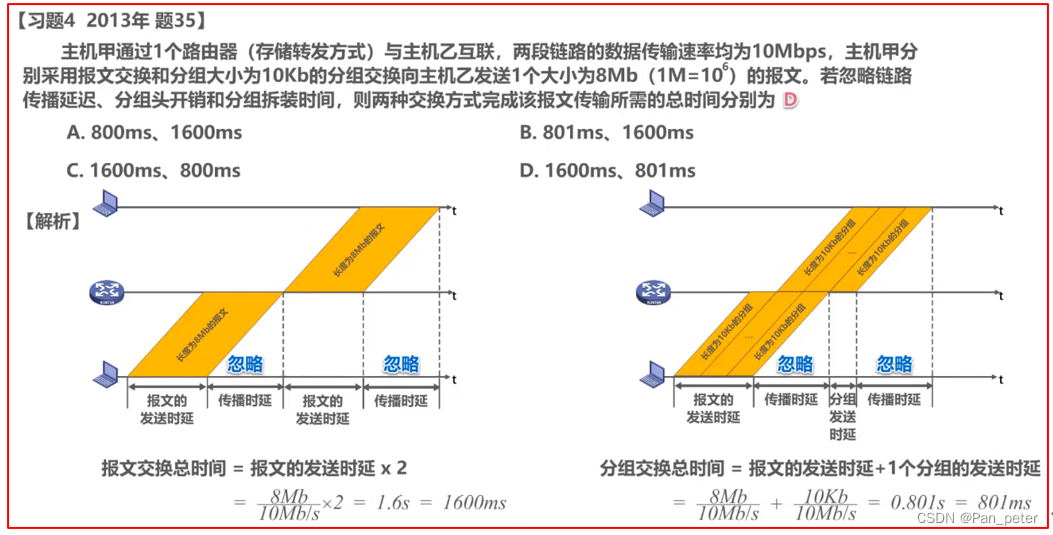
目录:
- 并查集的概念
- 代码实现
- LeetCode例题
并查集的概念
将n个不同的元素划分成一些不相交的集合。开始时,每个元素自成一个单元元素集合,然后按一定的规律将归于同一组元素的集合合并。在此过程中反复遇到查询某一个元素属于那个集合的运算,这种抽象的数据类型称为并查集。
主要思想:用集合中的一个元素代表集合。
代码实现
#include<iostream>
#include<vector>
class UnionFindSet
{
public:
UnionFindSet(size_t n)//构造函数
:_ufs(n,-1)
{}
void Union(int x1,int x2)//合并根
{
int root1 = FindRoot(x1);
int root2 = FindRoot(x2);
if (root1 == root2)//如果本身在一个集合就没必要合并了
return;
_ufs[root1] += _ufs[root2];//2个下标相加
_ufs[root2] = root1;//存一下根的下标
}
int FindRoot(int x)//查找根
{
int parent = x;
while (_ufs[parent] >= 0)//说明不是根
{
parent = _ufs[parent];
}
return parent;//f返回的编号是负数就是根
}
bool InSet(int x1, int x2)
{
return FindRoot(x1) == FindRoot(x2);//相等说明同一个根在同一个集合
}
size_t SetSize()//有几个集合
{
size_t size = 0;
for (size_t i = 0; i < _ufs.size(); ++i)
{
if (_ufs[i] < 0)//判断有几个负数就有几个集合,因为负数是根
{
++size;
}
}
return size;
}
private:
vector<int> _ufs;//编号找人
};
LeetCode例题
例题一:
116. 省份数量
有 n 个城市,其中一些彼此相连,另一些没有相连。如果城市 a 与城市 b 直接相连,且城市 b 与城市 c 直接相连,那么城市 a 与城市 c 间接相连。省份是一组直接或间接相连的城市,组内不含其他没有相连的城市。给你一个 n x n 的矩阵 isConnected ,其中 isConnected[i][j] = 1 表示第 i 个城市和第 j 个城市直接相连,而 isConnected[i][j] = 0 表示二者不直接相连。返回矩阵中省份的数量。
示例 1:
输入:isConnected = [[1,1,0],[1,1,0],[0,0,1]]
输出:2
示例 2:
输入:isConnected = [[1,0,0],[0,1,0],[0,0,1]]
输出:3
提示:
1 <= n <= 200
n == isConnected.length
n == isConnected[i].length
isConnected[i][j] 为 1 或 0
isConnected[i][i] == 1
isConnected[i][j] == isConnected[j][i]
代码解答:
class Solution {
public:
int findCircleNum(vector<vector<int>>& isConnected) {
vector<int> ufs(isConnected.size(),-1);
//手动函数
auto findRoot=[&ufs](int x)
{
while(ufs[x]>=0)//是负数才是根
x=ufs[x];
return x;
};
for(size_t i=0;i<isConnected.size();++i)
{
for(size_t j=0;j<isConnected[i].size();++j)
{
if(isConnected[i][j]==1)
{
//合并集合
int root1=findRoot(i);
int root2=findRoot(j);
if(root1!=root2)
{
ufs[root1]+=ufs[root2];
ufs[root2]=root1;//root2变成root1的孩子,root2的下标存的是root1是0
}
}
}
}
int n=0;
for(auto e: ufs)
{
if(e<0)
++n;
}
return n;
}
};
例题二:
990. 等式方程的可满足性
给定一个由表示变量之间关系的字符串方程组成的数组,每个字符串方程 equations[i] 的长度为 4,并采用两种不同的形式之一:“a等于b” 或 “a!=b”。在这里,a 和 b 是小写字母(不一定不同),表示单字母变量名。只有当可以将整数分配给变量名,以便满足所有给定的方程时才返回 true,否则返回 false。

代码解答:
class Solution {
public:
bool equationsPossible(vector<string>& equations) {
vector<int> ufs (26,-1);//26个字母的映射关系
auto findRoot=[&ufs](int x)
{
while(ufs[x]>=0)
x=ufs[x];
return x;
};
for(auto& str: equations)
{
if(str[1]=='=')
{
int root1=findRoot(str[0]-'a');
int root2=findRoot(str[3]-'a');
if(root1!=root2)
{
ufs[root1]+=ufs[root2];
ufs[root2]=root1;//root2变成root1的孩子
}
}
}
//判断不相等的在不在一个集合,在就相悖并返回false
for(auto& str: equations)
{
if(str[1]=='!')
{
int root1=findRoot(str[0]-'a');
int root2=findRoot(str[3]-'a');
if(root1==root2)
{
return false;
}
}
}
return true;
}
};



![java八股文面试[多线程]——自旋锁](https://img-blog.csdnimg.cn/e78f449da8544084b9af350c12006253.png)