C++ 帕斯卡三角形（Pascal’s Triangle）

帕斯卡三角形是二项式系数的三角形阵列。编写一个函数，以整数值N作为输入，并打印帕斯卡三角形的前N行。

例子：

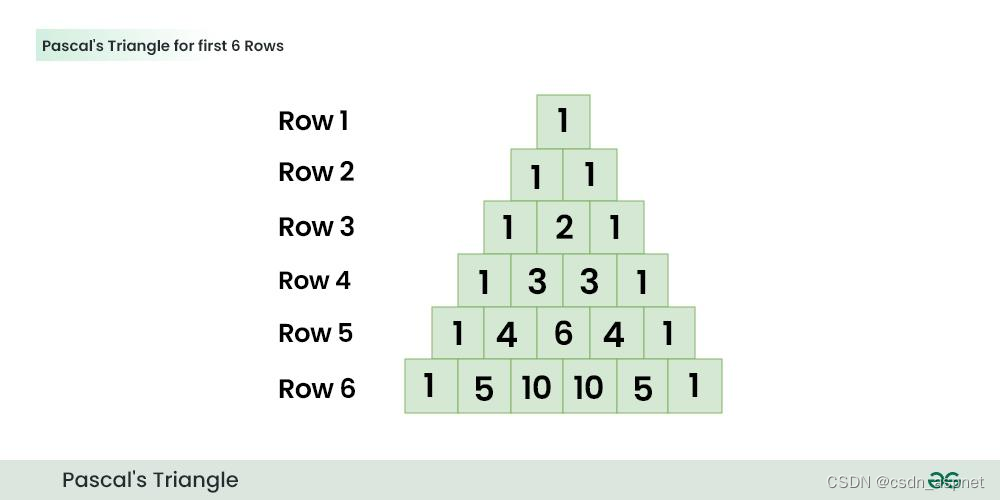
下图显示了 N=6 的帕斯卡三角形

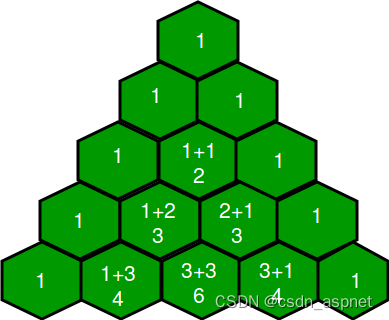
使用二项式系数的帕斯卡三角形：
每行的条目数等于行号。例如，第一行有“1 ”，第二行有“ 1 1 ”，第三行有“1 2 1 ”，等等。一行中的每个条目都是二项式系数的值。行号 line 中第 i 个条目的值为C(line, i)。可以使用以下公式计算该值。

C(line, i) = line! / ( (line-i)! * i! )

算法：

对帕斯卡三角形的每一行（即 1 到N ）运行一个循环。
       对于每一行，对该行的每个元素运行内部循环。
           使用方法中提到的公式计算元素的二项式系数。

下面是上述方法的实现：

// C++ code for Pascal's Triangle
#include <iostream>
using namespace std;

int binomialCoeff(int n, int k);

// Function to print first
// n lines of Pascal's
// Triangle
void printPascal(int n)
{
// Iterate through every line and
// print entries in it
for (int line = 0; line < n; line++) {
// Every line has number of
// integers equal to line
// number
for (int i = 0; i <= line; i++)
cout << " " << binomialCoeff(line, i);
cout << "\n";
}
}

// for details of this function

//https://blog.csdn.net/hefeng_aspnet/article/details/139958642
int binomialCoeff(int n, int k)
{
int res = 1;
if (k > n - k)
k = n - k;
for (int i = 0; i < k; ++i) {
res *= (n - i);
res /= (i + 1);
}

return res;
}

// Driver program
int main()
{
int n = 7;
printPascal(n);
return 0;
}

// This code is contributed by shivanisinghss2110

输出：

1
1 1
1 2 1
1 3 3 1
1 4 6 4 1
1 5 10 10 5 1
1 6 15 20 15 6 1

时间复杂度： O(N^3)，其中 N 是要打印的行数
辅助空间： O(1)

使用动态规划的帕斯卡三角形：
如果我们仔细观察三角形，我们会发现每个条目都是其上方两个值的总和。因此，使用动态规划，我们可以创建一个二维数组来存储先前生成的值。为了在一行中生成一个值，我们可以使用数组中先前存储的值。

案例：

if line == 0 or line == i
arr[line][i] =1
else:
arr[line][i] = arr[line-1][i-1] + arr[line-1][i]

下面是上述方法的实现：

// C++ program for Pascal’s Triangle
// A O(n^2) time and O(n^2) extra space
// method for Pascal's Triangle
#include <bits/stdc++.h>
using namespace std;

void printPascal(int n)
{

// An auxiliary array to store
// generated pascal triangle values
int arr[n][n];

// Iterate through every line and
// print integer(s) in it
for (int line = 0; line < n; line++)
{
// Every line has number of integers
// equal to line number
for (int i = 0; i <= line; i++)
{
// First and last values in every row are 1
if (line == i || i == 0)
arr[line][i] = 1;

// Other values are sum of values just
// above and left of above
else
arr[line][i] = arr[line - 1][i - 1] +
arr[line - 1][i];
cout << arr[line][i] << " ";
}
cout << "\n";
}
}

// Driver code
int main()
{
int n = 5;
printPascal(n);
return 0;
}

// This code is Contributed by Code_Mech.

输出：

1
1 1
1 2 1
1 3 3 1
1 4 6 4 1

时间复杂度：O(N^2)
辅助空间： O(N^2)

注意：此方法可以优化为使用 O(n) 额外空间，因为我们只需要前一行的值。因此，我们可以创建一个大小为 n 的辅助数组并覆盖值。以下是另一种仅使用 O(1) 额外空间的方法。

使用二项式系数的帕斯卡三角形（空间优化）：
该方法基于使用二项式系数的方法。我们知道行号 line 中的第 i 个条目是二项式系数 C(line, i) ，并且所有行都以值 1 开头。这个想法是使用C(line, i-1)计算C(line, i ) 。它可以在 O(1) 时间内计算出来。

C(line, i) = line! / ( (line-i)! * i! )
C(line, i-1) = line! / ( (line – i + 1)! * (i-1)! )

我们可以从以上两个表达式得出以下表达式。

C(line, i) = C(line, i-1) * (line – i + 1) / i

因此，可以在 O(1) 时间内通过 C(line, i-1) 计算出 C(line, i)

以下是该方法的实现：

// C++ program for Pascal’s Triangle
// A O(n^2) time and O(1) extra space
// function for Pascal's Triangle
#include <bits/stdc++.h>

using namespace std;
void printPascal(int n)
{

for (int line = 1; line <= n; line++) {
int C = 1; // used to represent C(line, i)
for (int i = 1; i <= line; i++) {

// The first value in a line is always 1
cout << C << " ";
C = C * (line - i) / i;
}
cout << "\n";
}
}

// Driver code
int main()
{
int n = 5;
printPascal(n);
return 0;
}

// This code is contributed by Code_Mech