【笔记】树状数组 目录
- 简介
- 引入
- 1. 直接暴力
- 2. 维护前缀和数组
- 总结
- 定义
- 前置知识: lowbit \operatorname{lowbit} lowbit 操作
- 区间的表示方法
- 操作
- 单点修改
- 前缀和查询
- 任意区间查询
- 例题1: 单点修改,区间查询
- 例题2: 区间修改,单点查询
- 例题3: 区间修改,区间查询
- (后附极限卡常代码,70ms,较优解)
简介
树状数组是一种树形数据结构,支持在 O ( log n ) O(\log n) O(logn) 的时间复杂度内进行 单点修改 和 查询前缀和 的操作。
- 优点:常数小,码量小,操作灵活简便。
- 缺点:只能用来维护具有 结合律 且 可差分 的信息。例如:区间和、积等,而不能维护区间最大(最小)值。
引入
现在想要让你实现两个操作:
- 单点修改
- 查询 [ 1 , x ] [1,x] [1,x] 的和
在没有学过树状数组的时候你会怎么做?
1. 直接暴力
单点修改虽然方便,但前缀和是 O ( n ) O(n) O(n) 复杂度。
2. 维护前缀和数组
这样做虽然查询是 O ( 1 ) O(1) O(1) 了,但单点修改又是 O ( n ) O(n) O(n)。
总结
- 暴力
- 修改: O ( 1 ) O(1) O(1)
- 查询: O ( n ) O(n) O(n)
- 前缀和
- 修改: O ( n ) O(n) O(n)
- 查询: O ( 1 ) O(1) O(1)
那么我们不妨考虑一个折中的办法,两种操作都是 O ( log n ) O(\log n) O(logn) 的复杂度。
定义
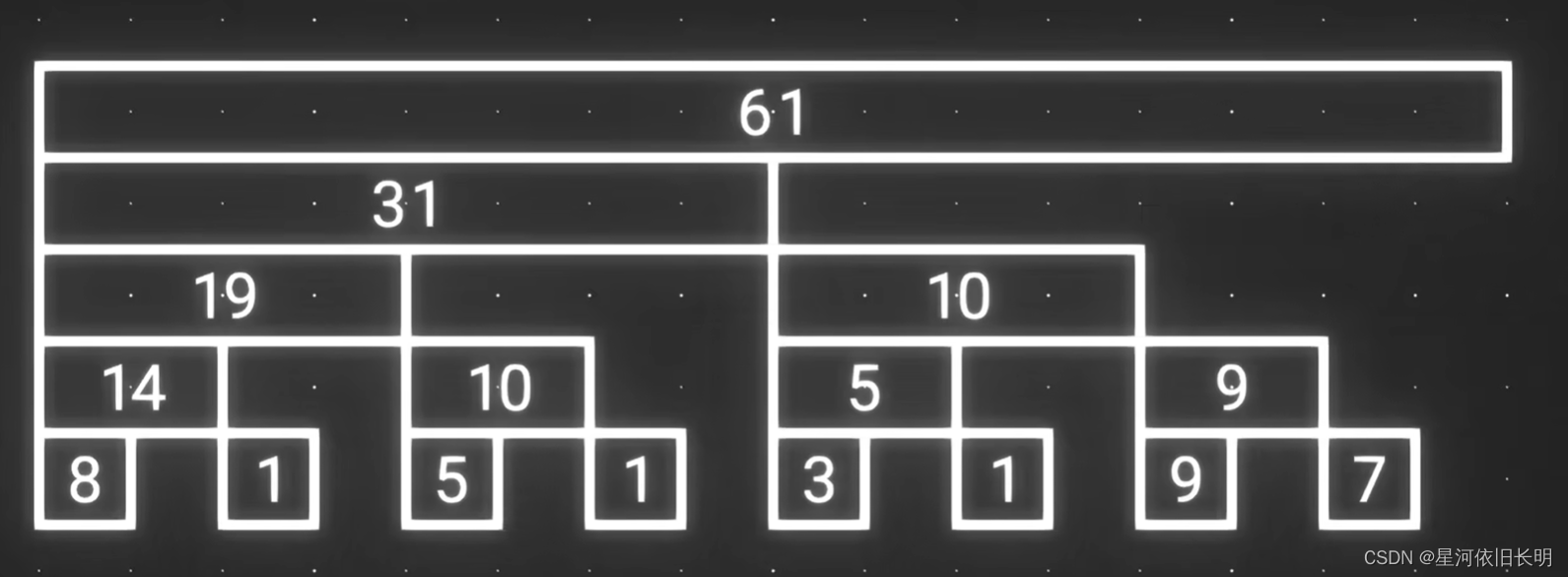
注:这里的数值表示的是该区间所有元素的和,也就是这个节点左下方的所有直接相关节点的总和。
例如:权值为
31
31
31 的节点表示的是权值分别为
19
,
10
,
1
19,10,1
19,10,1 的节点以及原数组中下表为
8
8
8 的元素之和。
显然,我们能求出原数组为
[ 8 , 6 , 1 , 4 , 5 , 5 , 1 , 1 , 3 , 2 , 1 , 4 , 9 , 0 , 7 , 4 ] [8,6,1,4,5,5,1,1,3,2,1,4,9,0,7,4] [8,6,1,4,5,5,1,1,3,2,1,4,9,0,7,4]
这里插一句话:树状数组可以近似看成线段树去掉所有右儿子构成的树。
前置知识: lowbit \operatorname{lowbit} lowbit 操作
一个二进制数的 lowbit \operatorname{lowbit} lowbit 值就是这个数末尾第一个非零的位置的权值。
举个例子: 10001 0 ( 2 ) 100010_{(2)} 100010(2)
这个数的 lowbit \operatorname{lowbit} lowbit 值是 1 0 ( 2 ) 10_{(2)} 10(2),即 2 ( 10 ) 2_{(10)} 2(10)。
那么这个怎么用代码实现呢?
void lowbit(int x)
{
return x & -x;
}
什么?你问为什么这么简单??
这都不知道,赶紧退役吧 h h \color{white}{这都不知道,赶紧退役吧hh} 这都不知道,赶紧退役吧hh
这里涉及到补码的概念。
一个二进制数的补码就是其二进制上的每一位都按位取反之后再 + 1 +1 +1。
还是那个数: 10001 0 ( 2 ) 100010_{(2)} 100010(2)
先按位取反: 01110 1 ( 2 ) 011101_{(2)} 011101(2)
再加一: 1111 0 ( 2 ) 11110_{(2)} 11110(2)
我们惊奇地发现,它们的后两位竟然是一样的!!!
我们把它们进行按位与运算 &,得到的结果是
1
0
(
2
)
10_{(2)}
10(2),即
2
(
10
)
2_{(10)}
2(10),与我们刚才进行手动
lowbit
\operatorname{lowbit}
lowbit 运算的结果相同。
在计算机的运算过程中,由于是按照补码储存的,所以我们需要的 ~x + 1 就可以写成 -x。
因此
lowbit
\operatorname{lowbit}
lowbit 才能写成 x & -x。
区间的表示方法

对于每个标号为 x x x 的节点,我们发现它父节点的标号为 x + lowbit x x+\text{lowbit}\ x x+lowbit x。
而每个区间的范围都是 ( x − lowbit ( x ) , x ] (x-\text{lowbit}(x),x] (x−lowbit(x),x]。
操作
单点修改
对于每个被修改的点,我们需要找到它的所有祖先节点并都进行修改操作。
考虑到它们标号的关系,我们只要每次加一个 lowbit(x) \text{lowbit(x)} lowbit(x) 就能找到所有祖先节点了。

代码:
void add(int x, int c) // 将第 x 个数加 c
{
for (int i = x; i <= n; i += lowbit(i))
tr[i] += c;
}
前缀和查询

实践是检验真理的唯一标准。
经过我们的实践,找到该节点前面的所有节点,只需要每次减 lowbit(x) \text{lowbit(x)} lowbit(x) 即可。
代码:
void query(int x) // 查询 1~x 的和
{
int res = 0;
for (int i = x; i; i -= lowbit(i))
res += tr[i];
return res;
}
任意区间查询
我们都知道前缀和的性质。
∑ i = l r w i = ∑ i = 1 r w i − ∑ i = 1 l − 1 w i \sum_{i=l}^{r}w_i=\sum_{i=1}^{r}w_i-\sum_{i=1}^{l-1}w_i i=l∑rwi=i=1∑rwi−i=1∑l−1wi
代码:
void Query(int l, int r) // 查询 [l,r] 的和
{
return query(r) - query(l - 1);
}
例题1: 单点修改,区间查询
原题链接:P3374 【模板】树状数组 1
操作和上面的相同,直接上代码:
#include <iostream>
using namespace std;
const int N = 500010;
int n, m;
int a[N];
int tr[N];
int lowbit(int x)
{
return x & -x;
}
void add(int x, int c)
{
for (int i = x; i <= n; i += lowbit(i))
tr[i] += c;
}
int sum(int x)
{
int res = 0;
for (int i = x; i; i -= lowbit(i))
res += tr[i];
return res;
}
int main()
{
int op, x, y;
scanf("%d%d", &n, &m);
for (int i = 1; i <= n; i ++ )
scanf("%d", &a[i]), add(i, a[i]);
while (m -- )
{
scanf("%d%d%d", &op, &x, &y);
if (op == 1) add(x, y);
else printf("%d\n", sum(y) - sum(x - 1));
}
return 0;
}
例题2: 区间修改,单点查询
原题链接:P3368 【模板】树状数组 2
同一道题,思路已经在昨天的 【笔记】线段树 里面讲了,无非是维护一个差分数组。
代码:
#include <iostream>
using namespace std;
const int N = 500010;
int n, m;
int a[N], b[N];
int tr[N];
int lb(int x)
{
return x & -x;
}
void add(int x, int v)
{
for (int i = x; i <= n; i += lb(i))
tr[i] += v;
}
int q(int x)
{
int res = 0;
for (int i = x; i; i -= lb(i))
res += tr[i];
return res;
}
int main()
{
cin >> n >> m;
for (int i = 1; i <= n; i ++ )
cin >> a[i], b[i] = a[i] - a[i - 1], add(i, b[i]);
while (m -- )
{
int op, x, y, k;
cin >> op >> x;
if (op == 1)
{
cin >> y >> k;
add(x, k), add(y + 1, -k);
}
else cout << q(x) << endl;
}
}
例题3: 区间修改,区间查询
原题链接:P3372 【模板】线段树 1
不要说我用线段树的题练习树状数组,我找不到树状数组的模板题才用的这个
考虑用树状数组 tr[] 维护差分数组
则求原数组的前缀和
{ a 1 = d 1 a 2 = d 1 + d 2 a 3 = d 1 + d 2 + d 3 . . . . . . a n = d 1 + d 2 + . . . + d n \left\{\begin{matrix} a_1& =& d_1& & & & & & & \\ a_2& =& d_1& +& d_2& & & & & \\ a_3& =& d_1& +& d_2& +& d_3& & & \\ .& .& .& .& .& .& & & & \\ a_n& =& d_1& +& d_2& +& ...& +& d_n& \\ \end{matrix}\right. ⎩ ⎨ ⎧a1a2a3.an===.=d1d1d1.d1++.+d2d2.d2+.+d3...+dn
s i = ∑ i = 1 n a i = { d 1 d 1 + d 2 d 1 + d 2 + d 3 . . . . . . d 1 + d 2 + . . . + d n s_i=\sum_{i=1}^{n}a_i=\left\{\begin{matrix} d_1& & & & & & & \\ d_1& +& d_2& & & & & \\ d_1& +& d_2& +& d_3& & & \\ .& .& .& .& .& .& & & & \\ d_1& +& d_2& +& ...& +& d_n& \\ \end{matrix}\right. si=i=1∑nai=⎩ ⎨ ⎧d1d1d1.d1++.+d2d2.d2+.+d3.....+dn
我们考虑把后面的矩阵补全:

则
s i = ( n + 1 ) × ∑ i = 1 n d i − ∑ i = 1 n ( i × d i ) s_i=(n+1) \times \sum_{i=1}^{n}d_i-\sum_{i=1}^{n}(i \times d_i) si=(n+1)×i=1∑ndi−i=1∑n(i×di)
所以我们需要两个树状数组,tr1[] 维护差分数组,tr2[] 维护
i
×
d
i
i \times d_i
i×di
代码:
#include <iostream>
using namespace std;
typedef long long LL;
const LL N = 1000010;
LL n, m;
LL a[N];
LL t1[N], t2[N];
inline LL lowbit(LL x)
{
return x & -x;
}
inline void add(LL t[], LL x, LL c)
{
for (LL i = x; i <= n; i += lowbit(i))
t[i] += c;
}
inline LL sum(LL t[], LL x)
{
LL res = 0;
for (LL i = x; i; i -= lowbit(i))
res += t[i];
return res;
}
inline LL psum(LL x)
{
return sum(t1, x) * (x + 1) - sum(t2, x);
}
int main()
{
scanf("%lld%lld", &n, &m);
for (LL i = 1; i <= n; i ++ ) scanf("%lld", &a[i]);
for (LL i = 1; i <= n; i ++ )
{
LL b = a[i] - a[i - 1];
add(t1, i, b);
add(t2, i, b * i);
}
while (m -- )
{
char op[2];
LL l, r, d;
scanf("%s%lld%lld", op, &l, &r);
if (op[0] == '2')
{
printf("%lld\n", psum(r) - psum(l - 1));
}
else
{
scanf("%lld", &d);
add(t1, l, d), add(t2, l, l * d);
add(t1, r + 1, -d), add(t2, r + 1, -d * (r + 1));
}
}
return 0;
}
最后,如果觉得对您有帮助的话,点个赞再走吧!
(后附极限卡常代码,70ms,较优解)
#define qwq optimize
#pragma GCC qwq(1)
#pragma GCC qwq(2)
#pragma GCC qwq(3)
#pragma GCC qwq("Ofast")
#pragma GCC qwq("inline")
#pragma GCC qwq("-fgcse")
#pragma GCC qwq("-fgcse-lm")
#pragma GCC qwq("-fipa-sra")
#pragma GCC qwq("-ftree-pre")
#pragma GCC qwq("-ftree-vrp")
#pragma GCC qwq("-fpeephole2")
#pragma GCC qwq("-ffast-math")
#pragma GCC qwq("-fsched-spec")
#pragma GCC qwq("unroll-loops")
#pragma GCC qwq("-falign-jumps")
#pragma GCC qwq("-falign-loops")
#pragma GCC qwq("-falign-labels")
#pragma GCC qwq("-fdevirtualize")
#pragma GCC qwq("-fcaller-saves")
#pragma GCC qwq("-fcrossjumping")
#pragma GCC qwq("-fthread-jumps")
#pragma GCC qwq("-funroll-loops")
#pragma GCC qwq("-fwhole-program")
#pragma GCC qwq("-freorder-blocks")
#pragma GCC qwq("-fschedule-insns")
#pragma GCC qwq("inline-functions")
#pragma GCC qwq("-ftree-tail-merge")
#pragma GCC qwq("-fschedule-insns2")
#pragma GCC qwq("-fstrict-aliasing")
#pragma GCC qwq("-fstrict-overflow")
#pragma GCC qwq("-falign-functions")
#pragma GCC qwq("-fcse-skip-blocks")
#pragma GCC qwq("-fcse-follow-jumps")
#pragma GCC qwq("-fsched-interblock")
#pragma GCC qwq("-fpartial-inlining")
#pragma GCC qwq("no-stack-protector")
#pragma GCC qwq("-freorder-functions")
#pragma GCC qwq("-findirect-inlining")
#pragma GCC qwq("-fhoist-adjacent-loads")
#pragma GCC qwq("-frerun-cse-after-loop")
#pragma GCC qwq("inline-small-functions")
#pragma GCC qwq("-finline-small-functions")
#pragma GCC qwq("-ftree-switch-conversion")
#pragma GCC qwq("-fqwq-sibling-calls")
#pragma GCC qwq("-fexpensive-optimizations")
#pragma GCC qwq("-funsafe-loop-optimizations")
#pragma GCC qwq("inline-functions-called-once")
#pragma GCC qwq("-fdelete-null-pointer-checks")
#include <iostream>
#include <cstdio>
#define lb(x) (x & (-x))
using namespace std;
typedef long long LL;
const LL N = 100010;
LL n, m;
LL a[N];
LL t1[N], t2[N];
char *p1, *p2, buf[N];
#define nc() (p1 == p2 && (p2 = (p1 = buf) +\
fread(buf, 1, N, stdin), p1 == p2) ? EOF : *p1 ++ )
LL read()
{
LL x = 0, f = 1;
char ch = nc();
while (ch < 48 || ch > 57)
{
if (ch == '-') f = -1;
ch = nc();
}
while (ch >= 48 && ch <= 57)
x = (x << 3) + (x << 1) + (ch ^ 48), ch = nc();
return x * f;
}
char obuf[N], *p3 = obuf;
#define putchar(x) (p3 - obuf < N) ? (*p3 ++ = x) :\
(fwrite(obuf, p3 - obuf, 1, stdout), p3 = obuf, *p3 ++ = x)
inline void write(LL x)
{
if (!x)
{
putchar('0');
return;
}
LL len = 0, k1 = x, c[40];
if (k1 < 0) k1 = -k1, putchar('-');
while (k1) c[len ++ ] = k1 % 10 ^ 48, k1 /= 10;
while (len -- ) putchar(c[len]);
}
inline void add(LL t[], LL x, LL c)
{
for (LL i = x; i <= n; i += lb(i))
t[i] += c;
}
inline LL sum(LL t[], LL x)
{
LL res = 0;
for (LL i = x; i; i -= lb(i))
res += t[i];
return res;
}
inline LL psum(LL x)
{
return sum(t1, x) * (x + 1) - sum(t2, x);
}
int main()
{
n = read(), m = read();
for (LL i = 1; i <= n; i ++ ) a[i] = read();
for (LL i = 1; i <= n; i ++ )
{
LL b = a[i] - a[i - 1];
add(t1, i, b);
add(t2, i, b * i);
}
LL op, l, r, d;
while (m -- )
{
op = read(), l = read(), r = read();
if (op == 2) write(psum(r) - psum(l - 1)), putchar(10);
else
{
d = read();
add(t1, l, d), add(t2, l, l * d);
add(t1, r + 1, -d), add(t2, r + 1, -d * (r + 1));
}
}
fwrite(obuf, p3 - obuf, 1, stdout);
return 0;
}