【题目来源】
https://www.luogu.com.cn/problem/P3372
【题目描述】
如题,已知一个数列,你需要进行下面两种操作:
(1)将某区间每一个数加上 k。
(2)求出某区间每一个数的和。
【输入格式】
第一行包含两个整数 n,m,分别表示该数列数字的个数和操作的总个数。
第二行包含 n 个用空格分隔的整数,其中第 i 个数字表示数列第 i 项的初始值。
接下来 m 行每行包含 3 或 4 个整数,表示一个操作,具体如下:
1 x y k:将区间 [x,y] 内每个数加上 k。
2 x y:输出区间 [x,y] 内每个数的和。
【输出格式】
输出包含若干行整数,即为所有操作 2 的结果。
【输入样例】
5 5
1 5 4 2 3
2 2 4
1 2 3 2
2 3 4
1 1 5 1
2 1 4
【输出样例】
11
8
20
【说明/提示】
对于 30% 的数据:n≤8,m≤10。
对于 70% 的数据:n≤10^3,m≤10^4。
对于 100% 的数据:1≤n,m≤10^5。
保证任意时刻数列中所有元素的绝对值之和 ≤10^18。
【算法分析】
● 本题其实就是线段树的模板题,这里用来练习分块。
● 分块是用线段树的分区思想改良的暴力法。代码比线段树简单。效率比普通暴力法高。分块适合求解 m=n=10^5 规模的问题。或 m*sqrt(n)≈10^7 的问题。其中,n 为元素个数,m 为操作次数。
● 分块操作的基本要素
(1)块的大小用 block 表示。通常,令 block=sqrt(n)。其中,n 为元素个数。
(2)块的数量用 cnt 表示。其计算公式为 cnt=(n+block-1)/block。或者,用如下更易理解的代码计算 cnt 的值。即:
int block=sqrt(n);
int cnt=n/block;
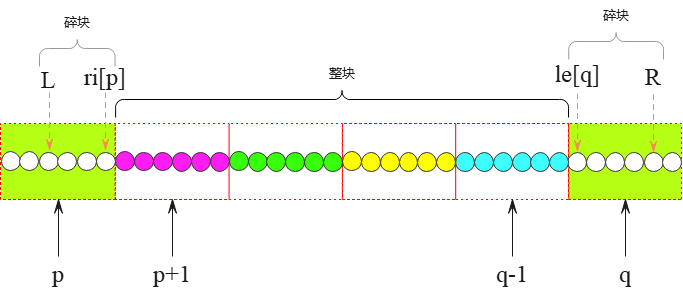
if(n % block) cnt++;(3)块的左边界 le[] 及右边界 ri[]。
若用 le[i] 和 ri[i] 分别表示块 i 的第一个和最后一个元素的位置。则有:
le[1]=1, ri[1]=block;
le[2]=block+1, ri[2]=2*block;
……
le[i]=(i-1)*block+1, ri[i]=i*block;
……
(4)定义 pos[i] 为第 i 个元素所在的块:pos[i]=(i-1)/block+1。其中,block=sqrt(n)。
(5)定义 sum[i] 为第 i 块的区间和。
for(int i=1; i<=n; i++) sum[pos[i]]+=a[i];(6)定义 add[i] 为第 i 块的增量,初始值为 0。
一般情况下,区间 [L,R] 跨越了多个块。
在被 [L,R] 完全包含的整块内,更新 add[i]=add[i]+d;
位于整块两头,不能被 [L,R] 完全包含的两个碎块,分别更新 sum(p)=sum(p)+(ri[p]-L+1)*d,sum(q)=sum(q)+(R-le[q]+1)*d。
【算法代码】
#include <bits/stdc++.h>
using namespace std;
typedef long long LL;
const int maxn=1e5+5;
LL a[maxn];
LL le[maxn],ri[maxn],pos[maxn];
LL sum[maxn],add[maxn];
int cnt;
void build(int n) {
int block=sqrt(n);
int cnt=n/block;
if(n%block) cnt++;
for(int i=1; i<=cnt; i++) {
le[i]=(i-1)*block+1;
ri[i]=i*block;
}
ri[cnt]=n;
for(int i=1; i<=n; i++) pos[i]=(i-1)/block+1;
for(int i=1; i<=n; i++) sum[pos[i]]+=a[i];
}
void update(int L,int R,int d) { //section update
int p=pos[L],q=pos[R];
if(p==q) {
for(int i=L; i<=R; i++) a[i]+=d;
sum[p]+=(R-L+1)*d;
} else {
for(int i=p+1; i<=q-1; i++) add[i]+=d;
for(int i=L; i<=ri[p]; i++) a[i]+=d;
sum[p]+=(ri[p]-L+1)*d;
for(int i=le[q]; i<=R; i++) a[i]+=d;
sum[q]+=(R-le[q]+1)*d;
}
}
LL query(int L,int R) { //LL
int p=pos[L],q=pos[R];
LL ans=0; //LL
if(p==q) {
for(int i=L; i<=R; i++) ans+=a[i];
ans+=add[p]*(R-L+1);
} else {
for(int i=p+1; i<=q-1; i++) ans+=sum[i]+add[i]*(ri[i]-le[i]+1);
for(int i=L; i<=ri[p]; i++) ans+=a[i];
ans+=add[p]*(ri[p]-L+1);
for(int i=le[q]; i<=R; i++) ans+=a[i];
ans+=add[q]*(R-le[q]+1);
}
return ans;
}
int main() {
int n,m;
cin>>n>>m;
for(int i=1; i<=n; i++) cin>>a[i];
build(n);
while(m--) {
int op,a,b,c;
cin>>op>>a>>b;
if(op==1) {
cin>>c;
update(a,b,c);
} else cout<<query(a,b)<<endl;
}
return 0;
}
/*
in:
5 5
1 5 4 2 3
2 2 4
1 2 3 2
2 3 4
1 1 5 1
2 1 4
out:
11
8
20
*/
【参考文献】
https://blog.csdn.net/m0_52398496/article/details/123233908
https://blog.csdn.net/weixin_45539557/article/details/116461380
https://blog.csdn.net/wzh1378008099/article/details/89243459