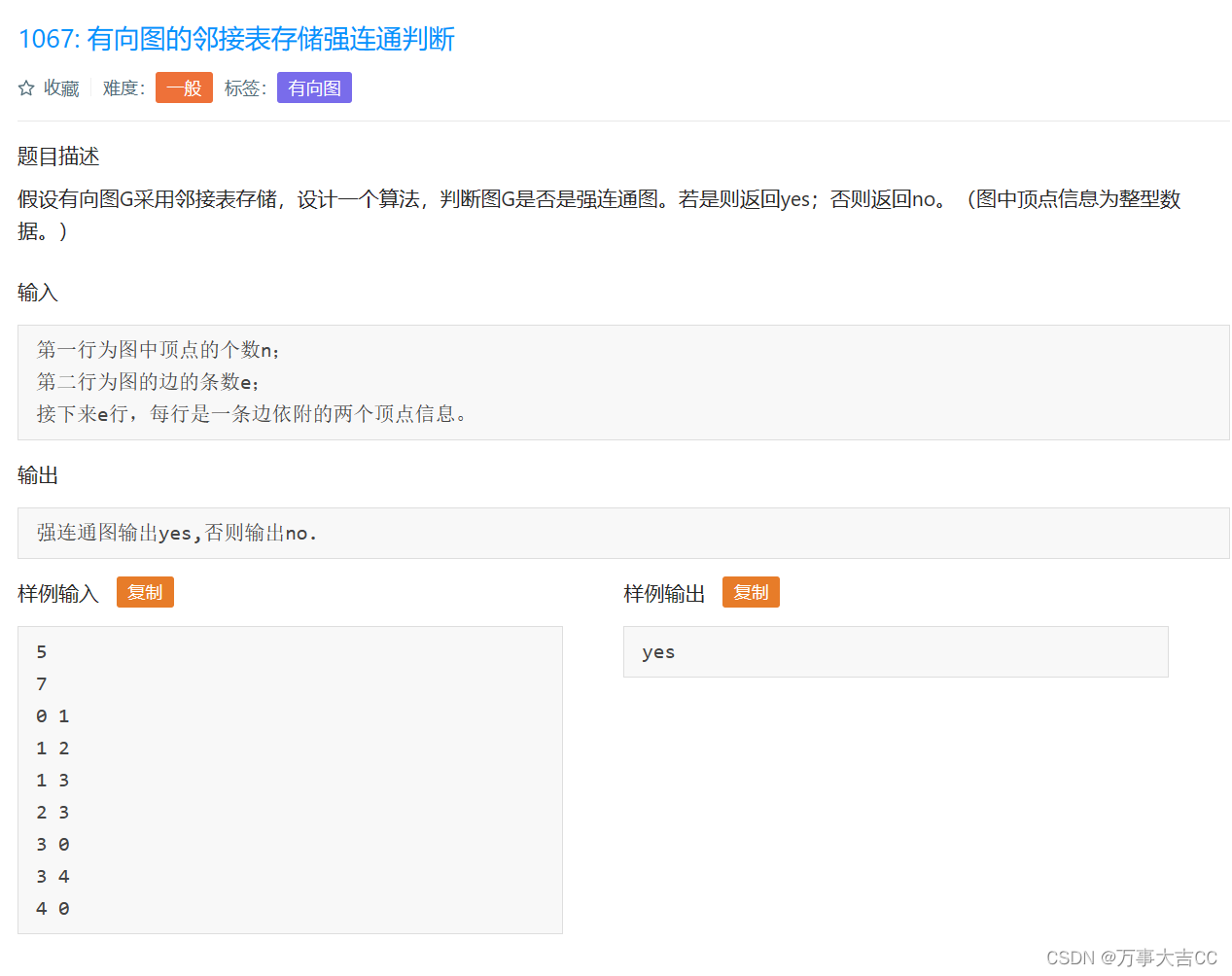
解法：

定理：有向图G是强连通图的充分必要条件是G中存在一条经过所有节点的回路

跟上道题一样

这是错误代码

#include<iostream>
#include<vector>
using namespace std;
int arr[100][100];
void dfs(vector<bool>& a,int u) {
	a[u] = true;
	for (int i = 0; i < a.size(); i++) {
		if (arr[u][i] && !a[i])
			dfs(a, i);
	}
}
int main() {
	int n, e;
	cin >> n >> e;
	vector<bool> vis(n, false);
	while (e--) {
		int x, y;
		cin >> x >> y;
		arr[x][y] = 1;
	}
	dfs(vis, 0);
	for (int i = 0; i < vis.size(); i++) {
		if (!vis[i]) {
			cout << "no";
			return 0;
		}
	}
	cout << "yes";
	return 0;
}

要搜完所有的节点，还要验证回路

回路用从每个节点出发能搜完所有节点处理

#include<iostream>
#include<vector>
using namespace std;
int arr[100][100];
int cnt;
void dfs(vector<int>& a,int u) {
	a[u] = 1;
	cnt++;
	for (int i = 0; i < a.size(); i++) {
		if (arr[u][i] && !a[i]) {
			dfs(a, i);
		}
	}
}
int main() {
	int n, e;
	cin >> n >> e;
	vector<int> vis(n, 0);
	while (e--) {
		int x, y;
		cin >> x >> y;
		arr[x][y] = 1;
	}
	for (int i = 0; i < n; i++) {
		cnt = 0;
		for (int& x : vis) x = 0;
		dfs(vis, i);
		if (cnt != n) {
			cout << "no";
			return 0;
		}
	}
	cout << "yes";
	return 0;
}