❣博主主页: 33的博客❣
▶️文章专栏分类:八大排序◀️
🚚我的代码仓库: 33的代码仓库🚚
🫵🫵🫵关注我带你了解更多排序知识

目录
- 1.前言
- 2.快速排序
- 2.1概念
- 2.2画图理解
- 2.3递归代码实现
- 2.3.1Hoare法
- 2.3.2挖坑法
- 2.3.3前后指针法
- 2.3.4优化
- 2.4非递归代码实现
- 3.总结
1.前言
关于排序的知识,我们已经介绍了直接插入排序,希尔排序,选择排序和堆排序,这篇文章博主就继续和大家分享快速排序的知识。
2.快速排序
2.1概念
快速排序是Hoare于1962年提出的一种二叉树结构的交换排序方法,其基本思想:任取待排序元素序列中的某元素作为基准值,按照该排序码将待排序集合分割城两个子序,左子序中的元素均小于基准值,右子序的元素均大于基准值,然后最左右子序列重复该过程,直到所有元素都排列在相应位置上为止。
2.2画图理解
选择排序
2.3递归代码实现
2.3.1Hoare法

public int[] quickOrder(int[] arr){
int p=0;
quick(0,arr.length-1,arr);
return arr;
}
private void quick(int start, int end, int[] arr) {
if (start>=end){
return;
}
int p=partionHoare(start,end,arr);
quick(start,p-1,arr);
quick(p+1,end,arr);
}
public int partionHoare(int l,int r,int[] arr){
int tmp=l;
while (l<r){
while (l<r&&arr[l]<arr[tmp]){
l++;
}
while (r>l&&arr[r]>arr[tmp]){
r--;
}
swap(l,r,arr);
}
//l==r
swap(l,tmp,arr);
return l;
}
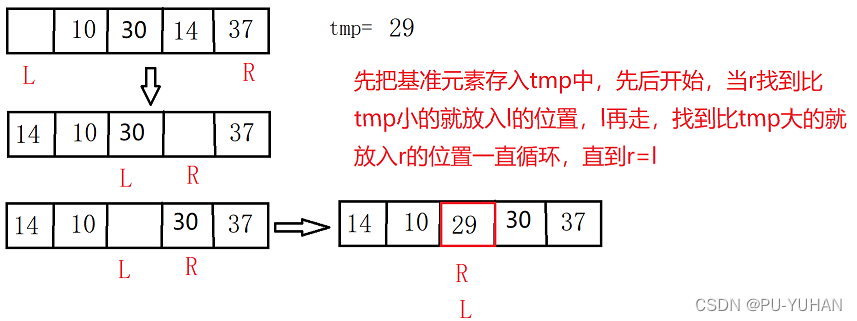
2.3.2挖坑法
private void quick(int start, int end, int[] arr) {
if (start>=end){
return;
}
int p=partitionHole(start,end,arr);
quick(start,p-1,arr);
quick(p+1,end,arr);
}
public int partitionHole(int l,int r,int[] arr){
int tmp=arr[l];
while (l<r){
if (l<r&&arr[r]>tmp){
r--;
}
arr[l]=arr[r];
if (l<r&&arr[l]<tmp){
l++;
}
arr[r]=arr[l];
}
arr[l]=tmp;
return l;
}
2.3.3前后指针法

private void quick(int start, int end, int[] arr) {
if (start>=end){
return;
}
int p=partition(start,end,arr);
quick(start,p-1,arr);
quick(p+1,end,arr);
}
private int partition( int left, int right,int[] array) {
int prev = left ;
int cur = left+1;
while (cur <= right) {
if(array[cur] < array[left] && array[++prev] != array[cur]) {
swap(cur,prev,array);
}
cur++;
}
swap(prev,left,array);
return prev;
2.3.4优化
观察上述代码,我们发现我们的基准值都是第一个元素,如果一个了比较有序的数组,我们进行快排,就可能形成当分支的树,所有我们要堆基准值进行优化,选取最左边,最右边元素和中间元素比较大小,把值为中间的作为基准元素。
public int[] quickOrder(int[] arr){
int p=0;
quick(0,arr.length-1,arr);
return arr;
}
private void quick(int start, int end, int[] arr) {
if (start>=end){
return;
}
if (end-start>=15){
insertOrder(start,end,arr);
return;
}
int m=mid(start,end, arr);
swap(start,m,arr);
int p=int p=partitionHole(start,end,arr);
quick(start,p-1,arr);
quick(p+1,end,arr);
}
public void insertOrder(int l,int r ,int[] arr){
for (int i=l+1;i<=r;i++){
int tmp=arr[i];
int j=i-1;
for (;j>=0;j--){
if(arr[j]>tmp){
arr[j+1]=arr[j];
}else break;
}
arr[j+1]=tmp;
}
}
public int mid(int l,int r,int[] arr){
int mid=(l+r)/2;
if (arr[l]<arr[r]){
if(arr[mid]<arr[l]){
return l;
}else if (arr[mid]>arr[r]){
return r;
}else {
return mid;
}
}else {
//arr[l]>=arr[r]
if (arr[mid]>arr[l]){
return l;
}else if (arr[mid]<arr[r]){
return r;
}else {
return mid;
}
}
}
public int partitionHole(int l,int r,int[] arr){
int tmp=arr[l];
while (l<r){
if (l<r&&arr[r]>tmp){
r--;
}
arr[l]=arr[r];
if (l<r&&arr[l]<tmp){
l++;
}
arr[r]=arr[l];
}
arr[l]=tmp;
return l;
}
2.4非递归代码实现
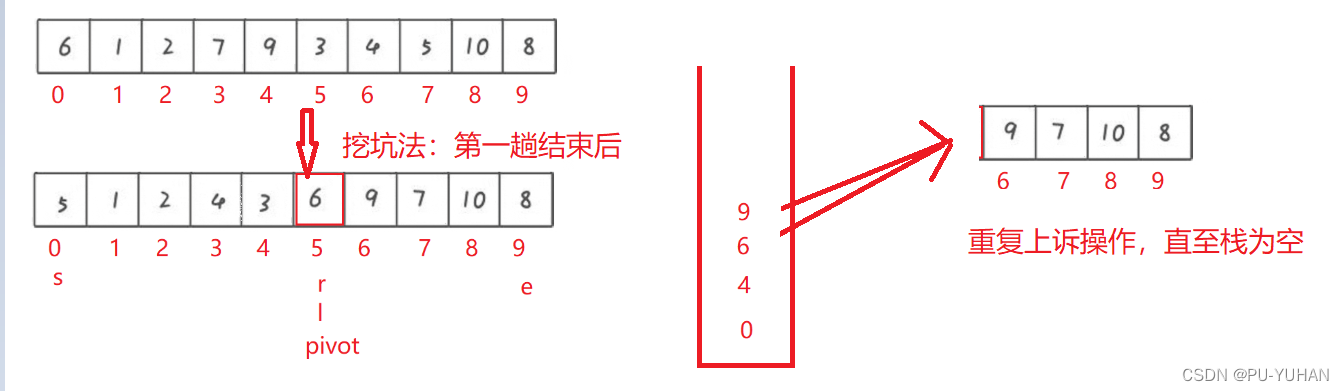
public int[] quickOrderNor(int[] arr){
Stack<Integer> stack=new Stack<>();
int s=0;
int e=arr.length-1;
int pivot=partitionHole(s,e,arr);
if (pivot-1>s){
stack.push(s);
stack.push(pivot-1);
}
if (e-1>pivot){
stack.push(pivot+1);
stack.push(e);
}
while (!stack.isEmpty()){
e=stack.pop();
s=stack.pop();
pivot=partitionHole(s,e,arr);
if (pivot-1>s){
stack.push(s);
stack.push(pivot-1);
}
if (e-1>pivot){
stack.push(pivot+1);
stack.push(e);
}
}
return arr;
}
时间复杂度:
最好的情况下:O(NlogN)
最坏情况下:O(N^2) 逆序/有序
空间复杂度:
最好的情况下:O(logN)
最坏情况下:O(N) 逆序/有序
稳定性:不稳定*
3.总结
快速排序是一个比较重要的排序算法,它可以用多种方法来实现,但不同方法的时间复杂度和空间复杂度。
下期预告:归并排序