1. 队列(Queue)——FIFO，先入先出的数据结构

队列是一种典型的FIFO数据结构。

FIFO的数据结构中，将首先处理添加到队列中的第一个元素。

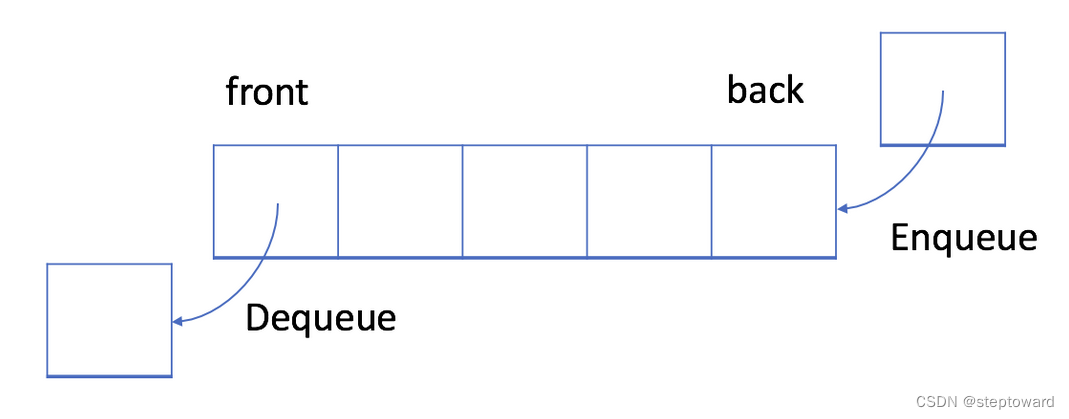
入队(Enqueue)：队列中，插入（insert）称作入队， 新插入的元素将被添加到队列的末尾。

出队(Dequeue)：出队时， 与入队相反，首先被操作的，是第一个元素。

1.1 循环队列

普通队列就不讲了，一旦一个队列满了，即使在队列前面仍有空间也不能插入下一个元素，这在实际上并不常用。
循环队列就是主要设计出来解决上述问题的。

class MyCircularQueue {
private:
    vector<int> data;
    int head;
    int tail;
    int size;
public:
    /** Initialize your data structure here. Set the size of the queue to be k. */
    MyCircularQueue(int k) {
        data.resize(k);
        head = -1;
        tail = -1;
        size = k;
    }
    
    /** Insert an element into the circular queue. Return true if the operation is successful. */
    bool enQueue(int value) {
        if (isFull()) {
            return false;
        }
        if (isEmpty()) {
            head = 0;
        }
        tail = (tail + 1) % size;
        data[tail] = value;
        return true;
    }
    
    /** Delete an element from the circular queue. Return true if the operation is successful. */
    bool deQueue() {
        if (isEmpty()) {
            return false;
        }
        if (head == tail) {
            head = -1;
            tail = -1;
            return true;
        }
        head = (head + 1) % size;
        return true;
    }
    
    /** Get the front item from the queue. */
    int Front() {
        if (isEmpty()) {
            return -1;
        }
        return data[head];
    }
    
    /** Get the last item from the queue. */
    int Rear() {
        if (isEmpty()) {
            return -1;
        }
        return data[tail];
    }
    
    /** Checks whether the circular queue is empty or not. */
    bool isEmpty() {
        return head == -1;
    }
    
    /** Checks whether the circular queue is full or not. */
    bool isFull() {
        return ((tail + 1) % size) == head;
    }
};

/**
 * Your MyCircularQueue object will be instantiated and called as such:
 * MyCircularQueue obj = new MyCircularQueue(k);
 * bool param_1 = obj.enQueue(value);
 * bool param_2 = obj.deQueue();
 * int param_3 = obj.Front();
 * int param_4 = obj.Rear();
 * bool param_5 = obj.isEmpty();
 * bool param_6 = obj.isFull();
 */

1.2 内置队列的常用方法（C++）

当你想要按顺序处理元素时，使用队列可能是一个很好的选择。不过大多数流行语言都提供内置的队列库，因此无需自己重新发明轮子。
empty(): 判空 (和stack一样)
pop(): 出 (和stack一样)
push(): 进 (和stack, vector一样)
front(): 获取第一个
back():获取最后一个

1.3 广度优先搜索（BFS）

广度优先搜索（BFS）的一个常见应用是找出从根结点到目标结点的最短路径.
第一轮处理根结点;
第二轮处理根结点旁边的结点；
第三轮处理距根结点两步的结点；
如果在第 k 轮中将结点 X 添加到队列中，则根结点与 X 之间的最短路径的长度恰好是 k。
BFS的模板代码（JAVA）：
每一轮中，逐个处理已经在队列中的结点，并将所有邻居添加到队列中。新添加的节点不会立即遍历，而是在下一轮中处理。

/**
 * Return the length of the shortest path between root and target node.
 */
int BFS(Node root, Node target) {
    Queue<Node> queue;  // store all nodes which are waiting to be processed
    int step = 0;       // number of steps neeeded from root to current node
    // initialize
    add root to queue;
    // BFS
    while (queue is not empty) {
        step = step + 1;
        // iterate the nodes which are already in the queue
        int size = queue.size();
        for (int i = 0; i < size; ++i) {
            Node cur = the first node in queue;
            return step if cur is target;
            for (Node next : the neighbors of cur) {
                add next to queue;
            }
            remove the first node from queue;
        }
    }
    return -1;          // there is no path from root to target
}

2.栈(Stack)——LIFO， 后入先出的数据结构

栈是一种典型的LIFO数据结构。

LIFO的数据结构中，将首先处理添加到队列中的第一个元素。

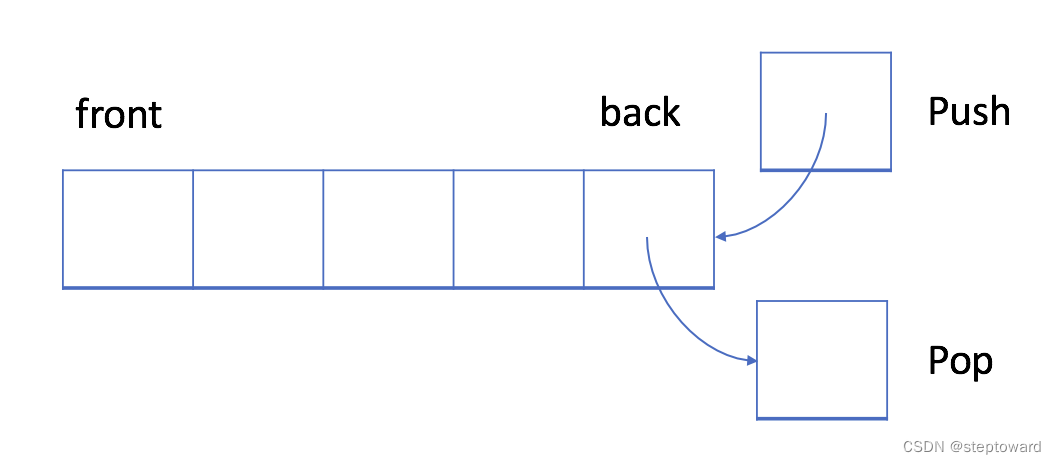
入栈(Push)：栈中，插入操作被称为入栈， 新插入的元素将被添加到堆栈的末尾。

出栈(Pop)：出栈时， 与入栈相同，首先被操作的，是最后一个元素。

2.1 栈的用法(C++)

push(): 入栈
pop(): 退栈
empty(): 判空
top(): 获取第一个

2.2 深度优先搜索（DFS）

深度优先搜索（DFS）也可用于查找从根结点到目标结点的路径。与 BFS 不同，更早访问的结点可能不是更靠近根结点的结点。因此，在 DFS 中找到的第一条路径可能不是最短路径。

DFS的模板代码（JAVA）：
显式栈实现 DFS:

/*
 * Return true if there is a path from cur to target.
 */
boolean DFS(int root, int target) {
    Set<Node> visited;
    Stack<Node> s;
    add root to s;
    while (s is not empty) {
        Node cur = the top element in s;
        return true if cur is target;
        for (Node next : the neighbors of cur) {
            if (next is not in visited) {
                add next to s;
                add next to visited;
            }
        }
        remove cur from s;
    }
    return false;
}