binary tree Leetcode 二叉树算法题

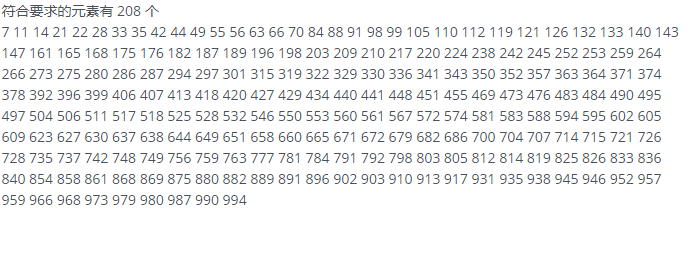
144.二叉树的前序遍历

前序遍历是：根-左-右

所以记录序列的的时候放在最前面

递归

class Solution {
    List<Integer> ans = new ArrayList<>();
    public List<Integer> preorderTraversal(TreeNode root) {
        if(root == null) return ans;
        ans.add(root.val);
        preorderTraversal(root.left);
        preorderTraversal(root.right);
        return ans;
    }
}

迭代

用栈模拟递归

  • 首先访问根节点，存储根节点的值
  • 随后访问左节点，每个左节点又是当前根节点，所以存储当前根节点的值，直到没有左节点就跳出循环
  • 跳出循环后，访问当前节点的右节点，从栈顶取出当前节点，同时由于当前节点的左右节点都被要访问了，所以直接弹出当前节点，然后访问当前节点的右节点
  • 右节点变成了当前根节点，记录值。回到步骤2判断是否有左节点
class Solution {
    public List<Integer> preorderTraversal(TreeNode root) {
        List<Integer> ans = new ArrayList<>();
        if(root == null) return ans;
        Deque<TreeNode> stack = new LinkedList<>();

        TreeNode node = root;
        while(node != null || !stack.isEmpty()){
            while(node != null){
                ans.add(node.val);
                stack.push(node);
                node = node.left;
            }
            node = stack.pop();
            node = node.right;
        }
        return ans;
    }
}

94.二叉树的中序遍历

递归

class Solution {
    List<Integer> ans = new ArrayList<>();
    public List<Integer> inorderTraversal(TreeNode root) {
        if(root == null) return ans;
        inorderTraversal(root.left);
        ans.add(root.val);
        inorderTraversal(root.right);
        return ans;
    }
}

迭代

class Solution {
   
    public List<Integer> inorderTraversal(TreeNode root) {
         List<Integer> ans = new ArrayList<>();
        if(root == null) return ans;
        
        Deque<TreeNode> stack = new LinkedList<>();
        TreeNode node = root;
        while(node != null || !stack.isEmpty()){
            while(node != null){
                stack.push(node);
                node = node.left;
            }
            node = stack.pop();
            ans.add(node.val);
            node = node.right;
        }
        return ans;
    }
}

145.二叉树的后序遍历

递归

class Solution {
    List<Integer> ans = new ArrayList<>();
    public List<Integer> postorderTraversal(TreeNode root) {
        if(root == null) return ans;
        postorderTraversal(root.left);
        postorderTraversal(root.right);
        ans.add(root.val);
        return ans;
    }
}

迭代

后序遍历：左-右-中

  • 遍历完左子树后，需要从栈中获得当前节点，来获得右子树，但是后续还需要遍历中间节点，所以需要加回去栈中
  • 但是当左右子树遍历完，就不需要再加回栈中了，只需要记录中间节点的值了
  • 通过prev记录上一个遍历的右节点，然后判断当前节点的右节点和prev是否指向同一个，就可以判断当前节点是否还需要继续遍历右节点了
class Solution {
    public List<Integer> postorderTraversal(TreeNode root) {
         List<Integer> ans = new ArrayList<>();
        if(root == null) return ans;
        
        Deque<TreeNode> stack = new LinkedList<>();
        TreeNode node = root, prev = null;
        while(node != null || !stack.isEmpty()){
            while(node != null){
                stack.push(node);
                node = node.left;
            }
            node = stack.pop();
            if(node.right == null || prev == node.right){
                ans.add(node.val);
                prev = node;
                node = null;
            }else{
                stack.push(node);
                node = node.right;
            }
        }
        return ans;
    }
}

102.二叉树的层序遍历

BFS

class Solution {
    public List<List<Integer>> levelOrder(TreeNode root) {
        List<List<Integer>> ans = new ArrayList<>();
        if(root == null) return ans;
        
        Deque<TreeNode> queue = new LinkedList<>();
        queue.offer(root);
        while(!queue.isEmpty()){
            int curLevel = queue.size();
            List<Integer> ansLevel = new ArrayList<>();
            for(int i = 0; i < curLevel; ++ i){
                TreeNode node = queue.poll();
                ansLevel.add(node.val);
                if(node.left != null) queue.offer(node.left);
                if(node.right != null) queue.offer(node.right);
            }
            ans.add(ansLevel);
        }
        return ans;
    }
}

我的代码

class Solution {
    public List<List<Integer>> levelOrder(TreeNode root) {
        List<List<Integer>> ans = new ArrayList<>();
        if(root == null) return ans;
        List<TreeNode> levelNode = new ArrayList<>();
        levelNode.add(root);
        while(levelNode.size() != 0){
            List<Integer> levelAns = new ArrayList<>();
            List<TreeNode> nextLevelNode = new ArrayList<>();
            for(TreeNode node : levelNode){
                levelAns.add(node.val);
                System.out.println(node.val);
                if(node.left != null)
                    nextLevelNode.add(node.left);
                if(node.right != null)
                    nextLevelNode.add(node.right);
            }
            levelNode = nextLevelNode;
            ans.add(levelAns);
        }
        return ans;
    }
}

226.翻转二叉树

递归

class Solution {
    public TreeNode invertTree(TreeNode root) {
        if(root == null) return root;

        TreeNode right = invertTree(root.left);
        TreeNode left = invertTree(root.right);
        root.right = right;
        root.left = left;
        return root;
    }
}

101.对称二叉树

根节点的左右子树是对称的，所以让两个子树同时往下遍历

左子树的左节点等于右子树的右节点，反之亦然

并且如果是迭代则让应该相等的节点放在临近的一起

递归

class Solution {
    public boolean isSymmetric(TreeNode root) {
        return check(root.left, root.right);
    }

    public boolean check(TreeNode l, TreeNode r){
        if(l == null && r == null) return true;
        if(l == null || r == null) return false;
        boolean check1 = check(l.left, r.right);
        boolean check2 = check(l.right, r.left);
        return l.val == r.val && check1 && check2;
    }
}

迭代

注意遍历到叶子节点的时候要 continue

class Solution {
    public boolean isSymmetric(TreeNode root) {
        Deque<TreeNode> queue = new LinkedList<>();
        queue.offer(root.left);
        queue.offer(root.right);
        while(!queue.isEmpty()){
            TreeNode l = queue.poll();
            TreeNode r = queue.poll();
            if(l == null && r == null) continue;
            if(l == null || r == null || l.val != r.val) return false;
            
            queue.offer(l.left);
            queue.offer(r.right);

            queue.offer(l.right);
            queue.offer(r.left);
        }
        return true;
    }
}

104.二叉树的最大深度

递归写法：

class Solution {
    public int maxDepth(TreeNode root) {
        if(root == null) return 0;
        int lnum = maxDepth(root.left);
        int rnum = maxDepth(root.right);
        return Math.max(lnum, rnum) + 1;
    }
}

111.二叉树的最小深度

class Solution {
    public int minDepth(TreeNode root) {
        if(root == null) return 0;
        int lmin = minDepth(root.left);
        int rmin = minDepth(root.right);
        if(lmin == 0 && rmin == 0) return 1;
        else if(lmin == 0 || rmin == 0) return Math.max(lmin, rmin)+1;
        return Math.min(lmin, rmin)+1;
    }
}

222.完全二叉树的节点个数

完全二叉树的性质

分左右子树遍历：

  • 如果左子树高度和右子树高度相等，则说明左子树已满，所以左子树的节点个数可以计算为 2 l L e v e l 2^{lLevel} 2lLevel，只需要计算右子树节点个数了
  • 如果左子树高度和右子树高度不相等，则右子树已满，所以右子树的节点个数可以计算为 2 r L e v e l 2^{rLevel} 2rLevel，只需要计算左子树节点个数了

img.png

class Solution {
    public int countNodes(TreeNode root) {
        if(root == null) return 0;
        int lLevel = countLevel(root.left);
        int rLevel = countLevel(root.right);
        if(lLevel == rLevel){
            return countNodes(root.right) + (1<<lLevel);
        }else{
            return countNodes(root.left) + (1<<rLevel);
        }
    }

    public int countLevel(TreeNode root){
        int level = 0;
        while(root != null){
            ++level;
            root = root.left;
        }
        return level;
    }
}

常规递归遍历

class Solution {
    public int countNodes(TreeNode root) {
        if(root == null) return 0;
        return countNodes(root.left) + countNodes(root.right) + 1;
    }
}

110.平衡二叉树

自顶向下递归

class Solution {
    public boolean isBalanced(TreeNode root) {
        if(root == null) return true;
        int lLevel = countLevel(root.left);
        int rLevel = countLevel(root.right);
        if(Math.abs(lLevel-rLevel) > 1) return false;
        return isBalanced(root.left) && isBalanced(root.right);
    }

    public int countLevel(TreeNode root){
        if(root == null) return 0;
        return Math.max(countLevel(root.left), countLevel(root.right)) + 1;
    }
}

countLevel被重复调用了

自底向上递归

  • 如果平衡就返回高度
  • 如果不平衡就返回一个负数
  • 后面判断是否子树已经不平衡，通过高度是否是负数检测
class Solution {
    public boolean isBalanced(TreeNode root) {
        return countLevel(root) >= 0;
    }

    public int countLevel(TreeNode root){
        if(root == null) return 0;
        int lLevel = countLevel(root.left);
        int rLevel = countLevel(root.right);
        if(lLevel == -1 || rLevel == -1 || Math.abs(lLevel-rLevel) > 1){
            return -1;
        }
        return Math.max(lLevel, rLevel) + 1;
    }
}

左子树已经不平衡，右子树就没必要递归下去了，直接 return

class Solution {
    public boolean isBalanced(TreeNode root) {
        return countLevel(root) >= 0;
    }

    public int countLevel(TreeNode root){
        if(root == null) return 0;
        int lLevel = countLevel(root.left);
        if(lLevel == -1) return -1;
        int rLevel = countLevel(root.right);
        if(rLevel == -1) return -1;
        if(Math.abs(lLevel-rLevel) > 1){
            return -1;
        }
        return Math.max(lLevel, rLevel) + 1;
    }
}

257.二叉树的所有路径

遇到叶子节点就把路径计入答案，否则把当前节点计入路径中

class Solution {
    public List<String> binaryTreePaths(TreeNode root) {
        List<String> paths = new ArrayList<>();
        TreePath(root, "", paths);
        return paths;
    }

    public void TreePath(TreeNode root, String path, List<String> paths){
        if(root == null) return;
        if(root.left == null && root.right == null){
            paths.add(path + root.val);
            return;
        }
        path += root.val + "->";
        TreePath(root.left, path, paths);
        TreePath(root.right, path, paths);
    }
}

String 的加法会不断的创建拷贝生成新的字符串，耗费时间和空间。可以用 StringBuilder 或者 StringBuffer 优化

但是它们属于引用类型，后面对其字符串的更改，回溯后更改依然存在，所以在回溯后需要delete掉多余的内容

class Solution {
    public List<String> binaryTreePaths(TreeNode root) {
        List<String> paths = new ArrayList<>();
        TreePath(root, new StringBuilder(), paths);
        return paths;
    }

    public void TreePath(TreeNode root, StringBuilder path, List<String> paths){
        if(root == null) return;
        if(root.left == null && root.right == null){
            paths.add(path.toString() + root.val); // toString 一定在前，+val在后
            return;
        }
        int prevLen = path.length();
        path.append(root.val).append("->");
        
        TreePath(root.left, path, paths);
        TreePath(root.right, path, paths);

        path.setLength(prevLen);
    }
}

404.左叶子之和

https://leetcode.cn/problems/sum-of-left-leaves/

  1. 首先必须有左节点
  2. 然后必须是叶子节点
  3. 每次的递归累计值不能丢失
class Solution {
    public int sum = 0;
    public int sumOfLeftLeaves(TreeNode root) {
        if(root == null) return sum;
        if(root.left != null && root.left.left == null && root.left.right == null)
            sum = sum + root.left.val;
        sumOfLeftLeaves(root.left);
        sumOfLeftLeaves(root.right);
        return sum;
    }
}

513.找树左下角的值

左下角的值，也就是从左往右dfs最深的一层的第一个节点

或者，bfs从左往右的第一个节点（从右往左的最后一个节点）

dfs

记录选择节点的深度

public class Solution {
    int curDepth = 1;
    int maxDepth = 0;
    int curVal = 0;
    public int findBottomLeftValue(TreeNode root) {
        if(root == null) return curVal;
        if(root.left == null && root.right == null){
            if(maxDepth < curDepth){
                maxDepth = curDepth;
                curVal = root.val;
                return curVal;
            }
        }
        curDepth += 1;
        findBottomLeftValue(root.left);
        findBottomLeftValue(root.right);
        curDepth -= 1;
        return curVal;
    }
}

112.路径总和

easy

求二叉树路径上所有节点的和是否等于某个数

每次向下递归的时候减去当前节点的值，直到叶子节点看是否减到0了

左右子树只要有一个返回 true，就存在

class Solution {
    public boolean hasPathSum(TreeNode root, int targetSum) {
        if(root == null) return false;
        if(root.left == null && root.right == null){
            if(targetSum - root.val == 0) return true;
            return false;
        }
        return hasPathSum(root.left, targetSum - root.val) 
                || hasPathSum(root.right, targetSum - root.val);
    }
}

106.从中序与后序遍历序列构造二叉树

中序遍历：左 [中] 右 ：获取左右子树的范围

后序遍历：左 右 [中] ：获取根节点

所以后序遍历的最后一个节点一定是根节点

  1. 首先根据后序遍历，其最后一个位置就是根节点idx
  2. 找到根节点idx对应中中序遍历的位置 mid ，获取左右子树的范围 [l, mid-1], [mid+1, r]
  3. 递归的在 [l, mid-1], [mid+1, r] 区间重复上述两个过程；
  4. 由于后序遍历是先左，再右，所以下一个根节点也就是–idx是右子树的根节点。所以必须先递归右区间，再递归左区间
class Solution {
    Map<Integer, Integer> hash;
    int idx;
    public TreeNode buildTree(int[] inorder, int[] postorder) {
        hash = new HashMap<>();
        for(int i = 0; i < inorder.length; ++ i){
            hash.put(inorder[i], i);
        }
        idx = inorder.length-1;
        return build(inorder, postorder, 0, inorder.length-1);
    }

    public TreeNode build(int[] inorder, int[] postorder, int l, int r){
        if(l > r){
            return null;
        }
        // if(l == r){
        //     idx--;
        //     return new TreeNode(inorder[l]);
        // }
        TreeNode root = new TreeNode(postorder[idx]);
        int mid = hash.get(postorder[idx--]);

        root.right = build(inorder, postorder, mid + 1, r);
        root.left = build(inorder, postorder, l, mid-1);
        return root;
    }
}

654.最大二叉树

给定一个不重复的整数数组 nums 。 最大二叉树 可以用下面的算法从 nums 递归地构建:

  • 创建一个根节点，其值为 nums 中的最大值。
  • 递归地在最大值 左边 的 子数组前缀上 构建左子树。
  • 递归地在最大值 右边 的 子数组后缀上 构建右子树。

递归

每次在指定区间内找到最大值作为根节点

class Solution {
    public TreeNode constructMaximumBinaryTree(int[] nums) {
        return build(nums, 0, nums.length-1);
    }

    public TreeNode build(int[] nums, int l, int r){
        if(l > r){
            return null;
        }
        int mid = l;
        for(int i = l + 1; i <= r; ++ i){
            if(nums[i] > nums[mid]){
                mid = i;
            }
        }

        TreeNode root = new TreeNode(nums[mid]);
        root.left = build(nums, l, mid - 1);
        root.right = build(nums, mid + 1, r);
        return root;
    }
}

单调栈

找到每个节点，左边第一个和右边第一个比它大的元素，其中较小的元素为当前节点的父节点

找到每个元素第一个比它大的元素可以用单调栈：

构造一个单调递减的栈，每当加入当前元素：

  • 如果栈顶元素比当前元素小，那么当前元素就是栈顶元素右边第一个比它大的
  • 如果栈顶元素比当前元素大，那么栈顶元素就是当前元素左边第一个比它大的
class Solution {
    public TreeNode constructMaximumBinaryTree(int[] nums) {
        int n = nums.length;
        int[] left = new int[n];
        int[] right = new int[n];
        Arrays.fill(left, -1);
        Arrays.fill(right, -1);
        Deque<Integer> stack = new ArrayDeque<>();
        TreeNode[] nodes = new TreeNode[n];
        for(int i = 0; i < n; ++ i){
            nodes[i] = new TreeNode(nums[i]);
            while(!stack.isEmpty() && nums[i] > nums[stack.peek()]){
                right[stack.pop()] = i;
            }
            if(!stack.isEmpty()){
                left[i] = stack.peek();
            }
            stack.push(i);
        }

        TreeNode root = null;
        for(int i = 0; i < n; ++ i){
            if(left[i] == -1 && right[i] == -1){
                root = nodes[i];
            }else if(left[i] == -1 || (right[i] != -1 && nums[left[i]] > nums[right[i]])){
                nodes[right[i]].left = nodes[i];
            }else{
                nodes[left[i]].right = nodes[i];
            }
        }
        return root;
    }
}

617.合并二叉树

class Solution {
    public TreeNode mergeTrees(TreeNode root1, TreeNode root2) {
        if(root1 == null && root2 == null){
            return null;
        }
        int val1 = root1 == null ? 0 : root1.val;
        int val2 = root2 == null ? 0 : root2.val;
        TreeNode root = new TreeNode(val1 + val2);
        root.left = mergeTrees(root1 == null ? null : root1.left, root2 == null ? null : root2.left);
        root.right = mergeTrees(root1 == null ? null : root1.right, root2 == null ? null : root2.right);
        return root;
    }
}

更简介的代码：

不需要判断两个通知为 null 才返回 null

只要有一方为 null，就返回另一方

class Solution {
    public TreeNode mergeTrees(TreeNode root1, TreeNode root2) {
        if(root1 == null){
            return root2;
        }
        if(root2 == null){
            return root1;
        }
        TreeNode root = new TreeNode(root1.val + root2.val);
        root.left = mergeTrees(root1.left, root2.left);
        root.right = mergeTrees(root1.right, root2.right);
        return root;
    }
}

700.二叉搜索树中的搜索

class Solution {
    public TreeNode searchBST(TreeNode root, int val) {
        if(root == null){
            return null;
        }
        if(root.val == val){
            return root;
        }
        if(root.val > val){
            return searchBST(root.left, val);
        }
        return searchBST(root.right, val);
    }
}

98.验证二叉搜索树

递归判断区间范围

class Solution {
    public boolean isValidBST(TreeNode root) {
        return check(root, Long.MIN_VALUE, Long.MAX_VALUE);
    }

    public boolean check(TreeNode root, long left, long right){
        if(root == null) return true;
        if(root.val <= left || root.val >= right) return false;

        return check(root.left, left, root.val) && check(root.right, root.val, right);
    }
}

中序遍历

迭代版本的，便于拿到前一个节点的值

530.二叉搜索树的最小绝对差

二叉搜索树的中序遍历结果中，相邻数字相减的最小值

迭代：

class Solution {
    public int getMinimumDifference(TreeNode root) {
        
        Deque<TreeNode> stack = new ArrayDeque<>();
        int pre = -1, res = Integer.MAX_VALUE;
        while(root != null || !stack.isEmpty()){
            while(root != null){
                stack.push(root);
                root = root.left;
            }
            root = stack.pop();
            if(pre != -1){
                res = Math.min(res, root.val - pre);
            }
            pre = root.val;
            root = root.right;
        }
        return res;
    }
}

中序遍历模板：

class Solution{
    public void minSearch(TreeNode root){
        Deque<TreeNode> stack = new ArrayDeque<>();
        // 节点不为空，或者栈里面还有剩下没被遍历的左节点们
        while(root != null || !stack.isEmpty()){
            // 中序遍历，先遍历到极左：
            while(root != null){
                stack.push(root);
                root = root.left;
            }
            // 中序遍历，极左遍历完了，遍历中间的节点：
            root = stack.pop(); // 栈中弹出最后遍历到的左节点
            root = root.rigth; // 最后遍历右节点
        }
    }
}

递归：

class Solution {

    int pre = -1;
    int ans = Integer.MAX_VALUE;

    public int getMinimumDifference(TreeNode root) {
        if(root == null){
            return ans;
        }
        getMinimumDifference(root.left);
        if(pre != -1){
            ans = Math.min(ans, root.val - pre);
        }
        pre = root.val;
        getMinimumDifference(root.right);
        return ans;
    }
}

236.二叉树的最近公共祖先

dfs

回溯的时候判断，该节点 x 本身及其子节点是否包含p或q中的一个，判断条件：

  • x == p || x == q || lson || rson

  • 如果一个节点 x 的左子树 lson 和 右子树 rson 各自包含一个 p 或者 q 节点，那么 x 就是最近公共祖先

  • 如果一个节点 x 本身是 p 或者 q 节点，x 的 lson 或者 rson 包含剩下的 p 或者 q 节点，那么 x 就是最近公共祖先

转换为：

  • (lson && rson) || ((x == p || x == q) && (lson || rson))
class Solution {
    TreeNode ans;
    public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
        ans = null;
        dfs(root, p, q);
        return ans;
    }

    public boolean dfs(TreeNode root, TreeNode p, TreeNode q){
        if(root == null) return false;

        boolean lson = dfs(root.left, p, q);
        boolean rson = dfs(root.right, p, q);

        if((lson && rson) || ((root.val == p.val || root.val == q.val)&&(lson || rson))){
            ans = root;
            return true;
        }

        return root.val == p.val || root.val == q.val || lson || rson;
    }
}

优雅的解法：

class Solution {
    public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
        if (root == null || root == p || root == q) {
            return root;     // 1)一个是另外一个的祖先
        }
        TreeNode left = lowestCommonAncestor(root.left, p, q);
        TreeNode right = lowestCommonAncestor(root.right, p, q);
        if (left != null && right != null) {
            return root;     // 2)左右两边各自有一个o1、o2，返回这个祖先"
        }
        return left != null ? left : right;
        // 1) / 2) 找不到，回溯时一直是null，如果找到了，那么将找到的值往上窜！
    }
}

存储父节点

从根节点 dfs，存储每个节点的父节点

从 p 开始向上搜索，将遇到的节点进行记录

再次从 q 开始向上搜索，如果遇到了之前遇到的节点，这个节点就是最近公共祖先

235.二叉搜索树的最近公共祖先

一次搜索两个节点

如果 p.val <= root.val <= q.val 那么 root 就是 LCA

class Solution {
    TreeNode ans = null;
    public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
        ans = null;
        dfs(root, p, q);
        return ans;
    }

    public void dfs(TreeNode root, TreeNode p, TreeNode q){
        if(root == null) return;
        if(root.val > p.val && root.val > q.val) dfs(root.left, p, q);
        else if(root.val < p.val && root.val < q.val) dfs(root.right, p, q);
        else ans = root;
    }
}

两次搜索

两次搜索获取两个目标节点的路径，路径的分叉点就是 LCA

class Solution {
    public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
        List<TreeNode> path_p = new ArrayList<>();
        List<TreeNode> path_q = new ArrayList<>();
        dfs(path_p, root, p);
        dfs(path_q, root, q);
        int i = 0;
        TreeNode ans = null;
        while(i < path_p.size() && i < path_q.size()){
            if(path_p.get(i).val == path_q.get(i).val){
                ans = path_p.get(i);
            }else break;
            ++i;
        }
        return ans;
    }

    public void dfs(List<TreeNode> path, TreeNode root, TreeNode target){
        path.add(root);
        if(root == target) return;
        if(root.val > target.val) dfs(path, root.left, target);
        else dfs(path, root.right, target);
    }
}

701.二叉搜索树中的插入操作

优雅写法

用递归时的方向直接判断左还是右

返回 root 节点给父节点，重建树

class Solution {
    boolean add = false;
    public TreeNode insertIntoBST(TreeNode root, int val) {
        return dfs(root, val);
    }
    public TreeNode dfs(TreeNode root, int val){
        if(root == null) return new TreeNode(val);
        if(val < root.val){
            root.left = dfs(root.left, val);
        }else{
            root.right = dfs(root.right, val);
        }
        return root;
    }
}

我的代码

找到插入的叶子节点，判断插入的方向。插入后限制不可插入：

class Solution {
    boolean add = false;
    TreeNode node = null;
    public TreeNode insertIntoBST(TreeNode root, int val) {
        node = new TreeNode(val);
        dfs(root, val);
        return root == null ? node : root;
    }
    public void dfs(TreeNode root, int val){
        if(root == null) return;
        int dir = 0;
        if(root.val > val) {
            dfs(root.left, val);
            dir = 1;
        }else dfs(root.right, val);
        if(add == false){
            if(dir == 0){
                root.right = node;
            }else{
                root.left = node;
            }
            add = true;
        }
    }
}

450.删除二叉搜索树中的节点

首先找到要删除的节点，然后分类讨论：

  • 如果删除节点左右子树为空，则直接返回 null
  • 如果删除节点左子树为空，返回右子树
  • 如果删除节点右子树为空，返回左子树
  • 如果删除节点左右子树都不为空：
    • 找到删除节点的后继节点，也就是 delete 节点的右子树的最左节点 node
    • 该 node 节点是仅仅只大于 delete节点的，所以用 node 代替 delete 节点，二叉树的性质保持不变
    • 替代方法：首先删除 node 节点，然后改变 node 的左右子树
class Solution {
    public TreeNode deleteNode(TreeNode root, int key) {
        if(root == null) return root;
        if(key < root.val){
            root.left = deleteNode(root.left, key);
            return root;
        }else if(key > root.val){
            root.right = deleteNode(root.right, key);
            return root;
        }else if(key == root.val){
            if(root.left == null && root.right == null){
                return null;
            }else if(root.left == null){
                return root.right;
            }else if(root.right == null){
                return root.left;
            }else{
                TreeNode node = root.right;
                while(node.left != null){
                    node = node.left;
                }
                // 这里要写 root.right = deleteNode(xx)，因为有可能删除的是root.right，就会返回 null
                root.right = deleteNode(root.right, node.val);
                node.left = root.left;
                node.right = root.right;
                return node;
            }
        }
        return root;
    }
}

696.修剪二叉搜索树

将不在区间 [low, high] 内的节点剪掉

  • 如果节点的值 小于 low ，则只用修剪右子树

  • 如果节点的值 大于 high，则只用修建左子树

  • 如果位于区间，则其左右子树可能不正常，所以递归修建左右子树

  • 修剪到最后，如果没有正常的节点则会一直递归到节点为 null，返回 null

  • 如果遇到正常节点，则返回该节点本身

class Solution {
    public TreeNode trimBST(TreeNode root, int low, int high) {
        if(root == null) return root;
        if(root.val < low){
            return trimBST(root.right, low, high);
        }else if(root.val > high){
            return trimBST(root.left, low, high);
        }else{
            root.left = trimBST(root.left, low, high);
            root.right = trimBST(root.right, low, high);
            return root;
        }
    }
}

108.将有序数组转换为二叉搜索树

每次选择区间内的中间值作为根节点，递归构造左右子树，

直到区间唯一个点返回该点构造的节点，或者 l > r 说明不存在左边或者右边的值，返回 null

返回左右子树构造好的根节点给上层的根节点构造左右子树

class Solution {
    public TreeNode sortedArrayToBST(int[] nums) {
        return build(nums, 0, nums.length - 1);
    }

    public TreeNode build(int[] nums, int l, int r){
        if(l == r){
            return new TreeNode(nums[l]);
        }else if(l > r){
            return null;
        }
        int mid = l + (r - l)/2;
        TreeNode root = new TreeNode(nums[mid]);
        root.left = build(nums, l, mid - 1);
        root.right = build(nums, mid + 1, r);
        return root;
    }
}

538.把二叉搜索树转换为累加树

反向中序遍历，累加之前的值：

class Solution {
    int sum = 0;
    public TreeNode convertBST(TreeNode root) {
        if(root == null) return root;
        convertBST(root.right);
        sum += root.val;
        root.val = sum;
        convertBST(root.left);
        return root;
    }
}

我的代码：

先dfs一遍算出总和

中序遍历一遍，减去之前的值

class Solution {
    int sum = 0;
    public TreeNode convertBST(TreeNode root) {
        dfs(root);
        if(root != null) sum += root.val;
        build(root);
        return root;
    }

    public int dfs(TreeNode root){
        if(root == null) return 0;
        int l = dfs(root.left);
        int r = dfs(root.right);
        sum = sum + l + r;
        return root.val;
    }

    public void build(TreeNode root){
        if(root == null) return;
        build(root.left);
        int sub = root.val;
        root.val = sum;
        sum -= sub;
        build(root.right);
    }
}

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：/a/561680.html

如若内容造成侵权/违法违规/事实不符，请联系我们进行投诉反馈qq邮箱809451989@qq.com，一经查实，立即删除！

相关文章

请编写函数fun，它的功能是：求出1到1000之内能被7或11整除、但不能同时被7和11整除的所有整数并将它们放在a所指的数组中，通过n返回这些数的个数。

请编写函数fun，它的功能是：求出1到1000之内能被7或11整除、但不能同时被7和11整除的所有整数并将它们放在a所指的数组中，通过n返回这些数的个数。

本文收录于专栏：算法之翼 https://blog.csdn.net/weixin_52908342/category_10943144.html 订阅后本专栏全部文章可见。 本文含有题目的题干、解题思路、解题思路、解题代码、代码解析。本文分别包含C语言、C++、Java、Python四种语言的解法和详细的解析。 题干 请编写函数fu…
阅读更多...
K8S哲学 - Pod、RC、RS、deployment

K8S哲学 - Pod、RC、RS、deployment

pod&#xff08;最小的可部署单元&#xff09; 容器组&#xff08;运行一个或多个容器&#xff09; Pod(容器组&#xff09;是Kubernetes 中最小的可部署单元。 一个Pod(容器组&#xff09;包含了一个应用程序容器&#xff08;某些情况下是多个容器&#xff09;、存储资源、 一…
阅读更多...
哈尔滨等保测评综述

哈尔滨等保测评综述

​ 定级是网络安全等级保护的首要环节和关键环节&#xff0c;可以梳理各行业、各部门、各单位的等级保护对象类型、重要程度和数量等基本信息&#xff0c;确定分级保护的重点。定级不准&#xff0c;系统备案、建设、整改、等级测评等后续工作都会失去意义&#xff0c;等级…
阅读更多...
Elastic 网络爬虫：为你的网站添加搜索功能

Elastic 网络爬虫：为你的网站添加搜索功能

作者&#xff1a;来自 Elastic Lionel Palacin 为了演示如何使用 Elastic 网络爬虫&#xff0c;我们将以一个具体的网站为例&#xff0c;讲解如何在该网站上添加搜索功能。我们将探讨发现网站的方法&#xff0c;并利用 Elastic 网络爬虫提供的功能&#xff0c;以最佳方式准备待…
阅读更多...
LeetCode238 除自身以外数组的乘积

LeetCode238 除自身以外数组的乘积

LeetCode238 除自身以外数组的乘积 给你一个整数数组 nums&#xff0c;返回 数组 answer &#xff0c;其中 answer[i] 等于 nums 中除 nums[i] 之外其余各元素的乘积 。 题目数据 保证 数组 nums之中任意元素的全部前缀元素和后缀的乘积都在 32 位 整数范围内。 请 **不要使…
阅读更多...
ubuntu下boa服务器编译运行

ubuntu下boa服务器编译运行

一.下载boa源码并解压 官网网站&#xff1a;BOA源码 点击箭头所指的位置即可下载 解压&#xff1a; tar -xvf boa-0.94.13.tar.gz 解压完成得到目录&#xff1a; 二.安装环境所缺依赖&#xff0c;否则编译会报错 sudo apt install bison sudo apt install flex 三.编译 1…
阅读更多...
基于SSM的养老院管理系统（含源码+sql+视频导入教程）

基于SSM的养老院管理系统（含源码+sql+视频导入教程）

&#x1f449;文末查看项目功能视频演示获取源码sql脚本视频导入教程视频 1 、功能描述 基于SSM的养老院管理系统4拥有三种角色 管理员&#xff1a;护工\工资\家属\楼房\床位\老人\档案\药品\外出\出入库\缴费\财务\退房管理 家属&#xff1a;填写评价、缴费、查看各种信息 …
阅读更多...
单细胞RNA测序（scRNA-seq）Seurat分析流程入门

单细胞RNA测序（scRNA-seq）Seurat分析流程入门

单细胞RNA测序（scRNA-seq）基础可查内容看以下文章： 单细胞RNA测序（scRNA-seq）工作流程入门 单细胞RNA测序（scRNA-seq）细胞分离与扩增 单细胞RNA测序（scRNA-seq）SRA数据下载及fastq-dumq数据拆分 单细胞RNA测序（scRNA-seq）Cellranger流程入门和数据质控 单细胞R…
阅读更多...
STL-vector类的使用及其模拟实现

STL-vector类的使用及其模拟实现

在C中&#xff0c;vector是标准模板库&#xff08;STL&#xff09;中的一种动态数组容器&#xff0c;它可以存储任意类型的元素&#xff0c;并且能够自动调整大小。vector提供了许多方便的成员函数&#xff0c;使得对数组的操作更加简单和高效。 vector的使用 vector的构造函数…
阅读更多...
Redis中的慢查询日志(一)

Redis中的慢查询日志(一)

慢查询日志 概述 Redis的慢查询日志功能用于记录执行时间超过给定时长的命令请求&#xff0c;用户可以通过这个功能产生的日志来 监视和优化查询速度。服务器配置有两个和慢查询日志相关的选项: 1.slowlog-log-slower-than选项指定执行时间超过多少微妙(1秒1000 000微妙)的命…
阅读更多...
ZeRO论文阅读

ZeRO论文阅读

一.前情提要 1.本文理论为主&#xff0c;并且仅为个人理解&#xff0c;能力一般&#xff0c;不喜勿喷 2.本文理论知识较为成体系 3.如有需要&#xff0c;以下是原文&#xff0c;更为完备 Zero 论文精读【论文精读】_哔哩哔哩_bilibili 二.正文 1.前言 ①为什么用该技术&…
阅读更多...
ctf.show_web14

ctf.show_web14

在switch中&#xff0c;case 里如果没有 break&#xff0c;则会继续向下执行 case。 过滤了information_schema.tables、information_schema.column、空格 information_schema.tables 或 .columns 用反引号 information_schema.tables 同时查3个字段 ?query-1/**/union/**/…
阅读更多...
【Python- 包，自定义模块，import】

【Python- 包，自定义模块，import】

Python- 包&#xff0c;自定义模块&#xff0c;import ■ 包■ 包创建■ 导入包&#xff0c;模块&#xff0c;函数方法■ __init__.py■ __all__ [my_module1] ■ 自定义模块■ 新建模块■ 导入自定义模块使用■ 导入不同模块的同名功能■ __all__变量 ■ import■ import 模块…
阅读更多...
Java 网络编程之TCP(二)：基于BIO的聊天室

Java 网络编程之TCP(二)：基于BIO的聊天室

在上一篇【Java 网络编程之TCP(一)&#xff1a;基于BIO】中&#xff0c;介绍Java中I/O和TCP的基本概念&#xff0c;本文在上文的基础上&#xff0c;实现一个基本的聊天室的功能。 聊天室需求描述&#xff1a; 聊天客户端&#xff1a;发送消息给所有其他客户端&#xff0c;接收…
阅读更多...
《剑指 Offer》专项突破版 - 面试题 113、114 和 115 : 详解拓扑排序（C++ 实现）

《剑指 Offer》专项突破版 - 面试题 113、114 和 115 : 详解拓扑排序（C++ 实现）

目录 前言 面试题 113 : 课程顺序 面试题 114 : 外星文字典 面试题 115 : 重建序列 前言 拓扑排序是指对一个有向无环图的节点进行排序之后得到的序列。如果存在一条从节点 A 指向节点 B 的边&#xff0c;那么在拓扑排序的序列中节点 A 出现在节点 B 的前面。一个有向无环…
阅读更多...
关于某次授权的大型内网渗透测试（1）

关于某次授权的大型内网渗透测试（1）

前期渗透&#xff1a; 打点&#xff1a;&#xff08;任意文件上传&#xff09; 直接发现头像处任意文件上传&#xff0c;这里直接上传冰蝎即可。 tasklist查看杀软 System Idle Process 0 N/A System …
阅读更多...
细说postgresql之pg_rman备份恢复 —— 筑梦之路

细说postgresql之pg_rman备份恢复 —— 筑梦之路

pg_rman是一款开源的备份恢复软件&#xff0c;支持在线和基于PITR的备份恢复方式。 pg_rman类似于oracle的rman&#xff0c;可以进行全量、增量、归档日志的备份。 运行模式&#xff1a; 安装部署 Releases ossc-db/pg_rman GitHub 1、需要根据PG Server的版本&#xff0c;下…
阅读更多...
05 MySQL--字段约束、事务、视图

05 MySQL--字段约束、事务、视图

1. CONSTRAINT 约束 创建表时&#xff0c;可以给表的字段添加约束&#xff0c;可以保证数据的完整性、有效性。比如大家上网注册用户时常见的&#xff1a;用户名不能为空。对不起&#xff0c;用户名已存在。等提示信息。 约束包括&#xff1a; 非空约束&#xff1a;not null检…
阅读更多...
6.6 完数（project） educoder项目实训

6.6 完数（project） educoder项目实训

前言 在最近的Python课上&#xff0c;做到这样一个“有趣”的作业&#xff0c;求得前n个完数&#xff0c;并输出其与其真约数的约数的加和式子&#xff0c;刚开始没怎么在意这个题&#xff0c;想着不就是做过好几遍了的语言基础练习题嘛&#xff0c;但是接下来的几小时没想到都…
阅读更多...
探索设计模式的魅力：开启智慧之旅，AI与机器学习驱动的微服务设计模式探索

探索设计模式的魅力：开启智慧之旅，AI与机器学习驱动的微服务设计模式探索

​&#x1f308; 个人主页&#xff1a;danci_ &#x1f525; 系列专栏&#xff1a;《设计模式》 &#x1f4aa;&#x1f3fb; 制定明确可量化的目标&#xff0c;坚持默默的做事。 ✨欢迎加入探索AI与机器学习驱动的微服务设计模式之旅✨ 亲爱的科技爱好者们&#xff0c;有没…
阅读更多...
最新文章

Copyright @ 2022~2023