前言
emmmmmm，dp杯居然不考dp了，蓝桥一直没怎么出过的高精度居然也考了（当时居然因为没太复习那块知识直接模拟混分了），题量也改了，总的来说反而简单了？。。。还好天津竞赛弱省，但愿能够省一吧。。。（图灵保佑）
好那么进入正题
第一题
好那么好，小水题一道(50 * 49 / 2)- (7 * 6 / 2)=1204,cout 出去,过啦！！（此处配有英雄哥圣音）
第二题
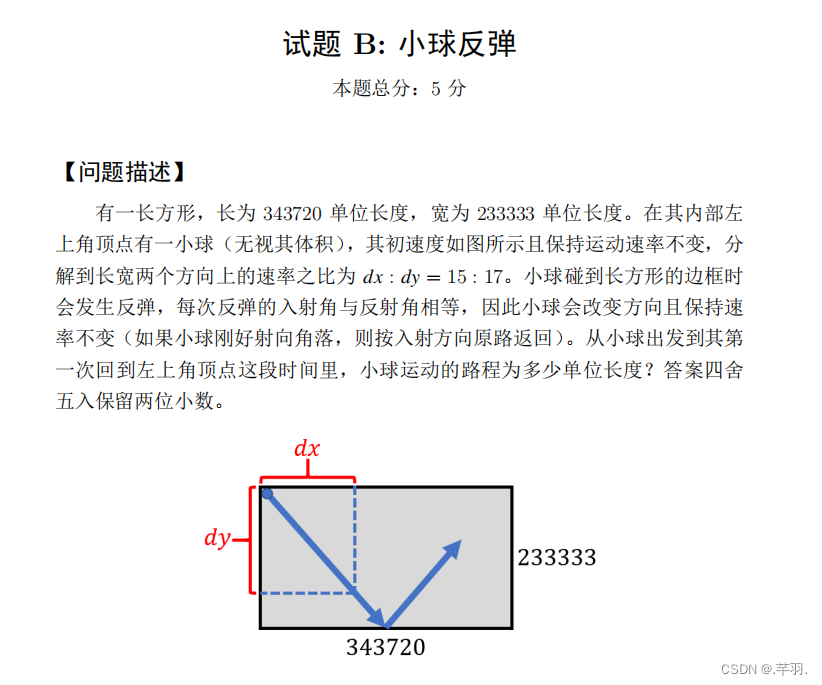
好，过过过过过过(卡壳)，过不了一点啊啊啊啊啊啊啊，很数学的一道题，原谅我这烂数学，此处只能引用某乎大佬的推理过程
大佬的思路真是清清又晰晰啊。。。。我的烂代码写的判断四个方向，转来转去转来转去，也没能得出答案
第三题
好那么好，蓝桥总是能在我快要崩溃的时候让我找回自信，很简单的一个模拟题，1e7的数据压根爆不了一点，吐槽一嘴：蓝桥正赛居然能遇见这么好写的题目了吗。。。
#include<iostream>
using namespace std;
bool is_good(int n){
int flag = 1;
while(n){
if((n%10)%2 != flag++ %2){
return false;
}
n /= 10;
}
return true;
}
int n,res;
int main()
{
cin >> n;
for(int i=1;i<=n;i++){
if(is_good(i))
res++;
}
cout << res;
return 0;
}第四题
嘿，不看数据量还真以为蓝桥要沦落为水赛了嘿，这刚一看不来个小白都会写吗，转头就是个暴击
高精度还忘了，但愿前50%错不了
第五题
很好的数论，使我的大脑旋转，爱来自蓝桥。很想知道大佬们怎么一眼就看出这个式子等于gcd(a,b,c)的，我赛场上直接暴力了，不过就这个数据量来看，数据量再大一点就会爆炸，（再近一点靠近点快被融化）(bushi)愿kunkun保佑我骗分
第六题
好，我真是太喜欢这种不在题目里玩一点心眼子，直接告诉你该干嘛的搜索题目了，数据量N小于等于10，那么我们直接上dfs ，走起
#include <iostream>
#include<set>
#include<vector>
using namespace std;
int f[8][2] = {
{-1, 0} ,
{-1, 1} ,
{0 , 1} ,
{1 , 1} ,
{1 , 0} ,
{1 ,-1} ,
{0 ,-1} ,
{-1,-1}
};
vector<int> ans;
set<pair<int, int>> se;
int a[12][12], flag = 1;
bool vis[12][12];
int n, k;
void dfs(int x, int y) {
if (flag == 0) {
return;
}
if (x == n && y == n && ans.size() == n * n - 1) {
flag = 0;
for (int i = 0; i < ans.size(); i++) {
cout << ans[i];
}
return;
}
if (x == 0 || y == 0 || x > n || y > n) {
return;
}
for (int i = 0; i < 8; i++)
{
int gox = x + f[i][0];
int goy = y + f[i][1];
int t = (x - 1) * n + y, to = (gox - 1) * n + goy;
pair<int, int> road({ t,to });
if (vis[gox][goy] == false && (a[x][y] + 1) % k == a[gox][goy] && se.find(road) == se.end()) {
vis[gox][goy] = true;
ans.push_back(i);
if (i == 1) {
se.insert({ t + 1,t - n });
se.insert({ t - n,t + 1 });
}
else if (i == 3) {
se.insert({ t + 1,t + n });
se.insert({ t + n,t + 1 });
}
else if (i == 5) {
se.insert({ t - 1 ,t + n });
se.insert({ t + n,t - 1 });
}
else if (i == 7) {
se.insert({ t - 1,t - n });
se.insert({ t - n,t - 1 });
}
dfs(gox, goy);
if (i == 1) {
se.erase({ t + 1,t - n });
se.erase({ t - n,t + 1 });
}
else if (i == 3) {
se.erase({ t + 1,t + n });
se.erase({ t + n,t + 1 });
}
else if (i == 5) {
se.erase({ t - 1 ,t + n });
se.erase({ t + n,t - 1 });
}
else if (i == 7) {
se.erase({ t - 1,t - n });
se.erase({ t - n,t - 1 });
}
vis[gox][goy] = false;
ans.pop_back();
}
}
return;
}
int main()
{
cin >> n >> k;
vis[1][1] = true;
for (int i = 1; i <= n; i++) {
for (int j = 1; j <= n; j++) {
cin >> a[i][j];
}
}
dfs(1, 1);
if (flag) {
cout << -1;
}
return 0;
}
我的acmer学长告诉我这样写常数太大了，容易炸，但愿还能混过前80%。。但是dotcpp上的民间数据倒是确确实实ac过去了，又是听天由命的一集，提一嘴，路径不让交叉的存储方法我看有大佬写的四维数组来标记，也就是记录起点(x0,y0)以及终点(x1,y1) 我更倾向于用(x-1) * n + y把他们弄成编号 ,虽然当时没想到数组去记录就好(会比set的插入删除操作更快，可能当时脑抽了吧，临场的时候就是想到啥直接用了，没有啥时间去做更多的考虑)
第七题
对于任意一个大于等于2的数来说，开根号并向下取整肯定是优于（或等于）减半的，所以我们利用优先队列(堆)每次对最大数进行开根号操作（P次以后变成减半操作），然后弹出并把操作后得到的新数放回去就行了，也就是堆+贪心，但有大佬把出题人hack了
恕我太菜没想到这种数据的解决办法，而且赛场上这个题我压根就没写。。。(调暴搜花了1.5h给自己整累了，觉得反正后面更难懒得写了)
附代码（非本人手写）
#include<iostream>
#include<queue>
#include<algorithm>
#include<cmath>
using namespace std;
typedef long long ll;
int n,P,Q;
int a[100010];
int main()
{
priority_queue<int> heap;
scanf("%d%d%d",&n,&P,&Q);
for(int i=1;i<=n;i++) scanf("%d",&a[i]),heap.push(a[i]);
while(P||Q)
{
auto x=heap.top();
heap.pop();
if(P&&Q)
{
int yl=sqrt(x);
int y2=x/2;
if(y2<=yl)
{
Q--;
heap.push(y2);
}
else
{
P--;
heap.push(yl);
}
}
else if(P)
{
int yl=sqrt(x);
P--;
heap.push(yl);
}
else
{
int y2=x/2;
Q--;
heap.push(y2);
}
}
ll res=0;
while(!heap.empty())
{
int x=heap.top();
heap.pop();
res+=x;
}
printf("%lld\n",res);
return 0;
}第八题
没什么好解释的，纯粹的不会，当时感觉这个题前缀和应该是有用的，但是就是一点都不想写了，就是累了摆了菜了qwq呜呜呜呜。。。。
吐槽
感觉总体上比去年是更简单了，去年最后那俩lca我当时大一是真一点都看不懂，emmmm，今年好像真的没有dp，难道dp杯知道自己黑称要改过自新？
求求今年让孩子混个省一吧（哭哭哭哭qaq qaq）
