【算法分析与设计】全排列

📝个人主页：五敷有你

🔥系列专栏：算法分析与设计

⛺️稳中求进，晒太阳

题目

给定一个不含重复数字的整数数组 nums ，返回其 所有可能的全排列 。可以 按任意顺序 返回答案。

示例

示例 1：

输入：nums = [1,2,3]
输出：[[1,2,3],[1,3,2],[2,1,3],[2,3,1],[3,1,2],[3,2,1]]

示例 2：

输入：nums = [0,1]
输出：[[0,1],[1,0]]

示例 3：

输入：nums = [1]
输出：[[1]]

思路

回溯

这个问题可以看作是n个排列成一行的空格，我们需要从左向右填入给定的n个数，每个只能使用一次，那么很直接的可以想到穷举法，从左向右依次填入，看能不能填完这个n个空格，在这个过程可以使用回溯来模拟。

我们定义一个backtrack(first,output)表示从左向右填到first个位置，当前排列为output,那么整个递归函数分为两个情况：

一、first==n

这个情况就表示已经填完了n个位置，可以进行一次排列的收集

二、first<n

这个情况是从第一个格到first是固定了，之后就

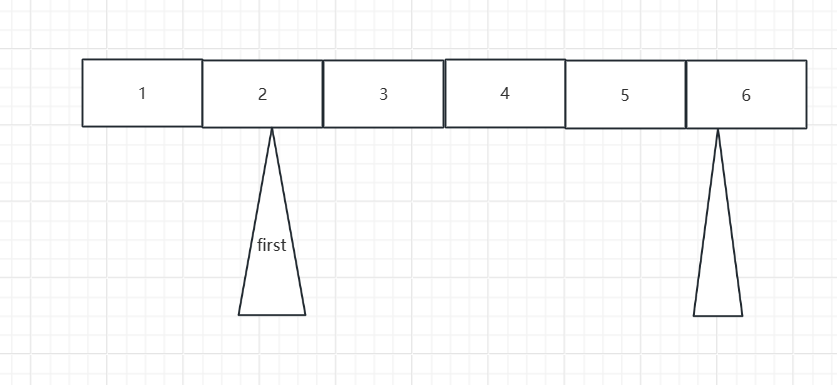
之后first左边的固定了，右边的还在回溯。

first现在指向2，之后2和2自己进行交换，有人可能回想为什么2还要与自己进行交换，因为1 2 3 4 5 6 本身也是一种排列，难道不是吗？呼

然后first指向3，同理，自身与自身交换一次，然后一直回溯，。。。。

之后再回来回溯交换

     for (int i = first; i < n; i++) {
            // 动态维护数组
            Collections.swap(output, first, i);
            // 继续递归填下一个数
            backtrack(n, output, res, first + 1);
            // 撤销操作
            Collections.swap(output, first, i);
        }

代码实现：

class Solution {
    public List<List<Integer>> permute(int[] nums) {
        List<List<Integer>> res = new ArrayList<List<Integer>>();

        List<Integer> output = new ArrayList<Integer>();
        for (int num : nums) {
            output.add(num);
        }

        int n = nums.length;
        backtrack(n, output, res, 0);
        return res;
    }

    public void backtrack(int n, List<Integer> output, List<List<Integer>> res, int first) {
        // 所有数都填完了
        if (first == n) {
            res.add(new ArrayList<Integer>(output));
        }
        for (int i = first; i < n; i++) {
            // 动态维护数组
            Collections.swap(output, first, i);
            // 继续递归填下一个数
            backtrack(n, output, res, first + 1);
            // 撤销操作
            Collections.swap(output, first, i);
        }
    }
}