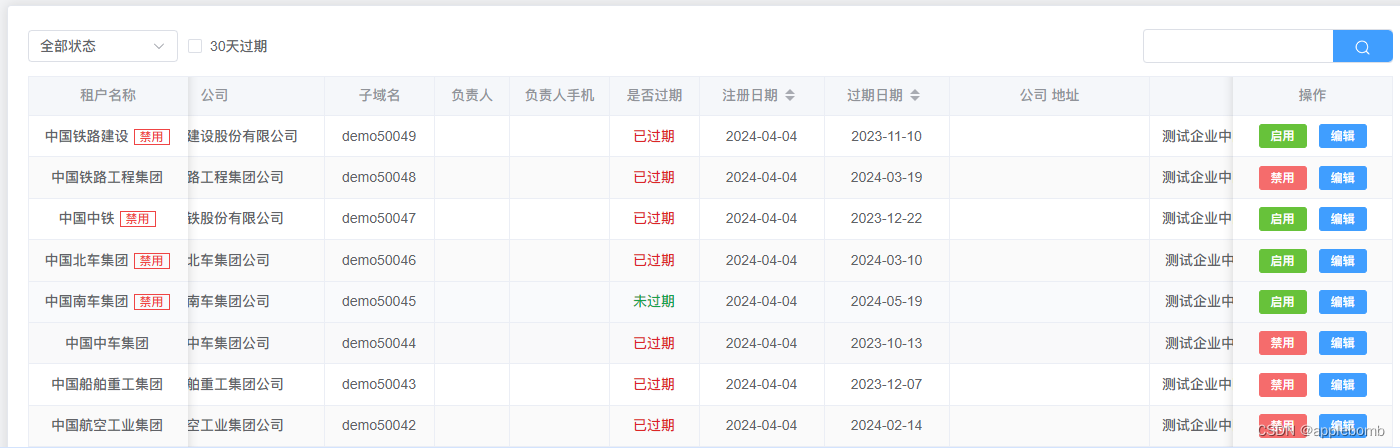
蓝桥集训之斐波那契数列
-
核心思想:矩阵乘法
-
将原本O(n)的递推算法优化为O(log2n)
-

-
构造1x2矩阵f和2x2矩阵a
-
发现f(n+1) = f(n) * a
- 则f(n+1) = f(1) * an
- 可以用快速幂优化
-
-
#include <iostream> #include <cstring> #include <algorithm> using namespace std; const int MOD = 10000; int f[2]; int a[2][2]; int n; void mul1() { int res[2]; //res = res*a 求1x2矩阵 memset(res,0,sizeof res); for(int i=0;i<2;i++) for(int j=0;j<2;j++) res[i] = (res[i] + f[j] * a[j][i]) %MOD; //计算f*a memcpy(f,res,sizeof f); } void mul2() { int res[2][2]; //a = a*a 求2x2矩阵 memset(res,0,sizeof res); for(int i=0;i<2;i++) for(int j=0;j<2;j++) for(int k=0;k<2;k++) res[i][j] = (res[i][j] + a[i][k] * a[k][j])%MOD; //计算a*a memcpy(a,res,sizeof a); } void qmi(int n) { while (n) //快速幂优化 { if(n&1) mul1(); //res = res*a%MOD mul2(); //a = a*a%MOD n>>=1; } } int main() { while(cin>>n , n!=-1) { f[0] = 0,f[1] = 1; //初始化第0 1项 a[0][0] = 0,a[0][1] = 1,a[1][0] = 1,a[1][1] = 1; //初始化a矩阵 qmi(n); cout<<f[0]<<endl; } return 0; }








![[机器学习]人工智能为小米智架保驾护航](https://img-blog.csdnimg.cn/9ce5039ffe5246f78843a13ab28ffc79.jpg)