1. 两数之和
给定一个整数数组 nums 和一个整数目标值 target,请你在该数组中找出 和为目标值 target 的那 两个 整数,并返回它们的数组下标。
你可以假设每种输入只会对应一个答案。但是,数组中同一个元素在答案里不能重复出现。
你可以按任意顺序返回答案。
示例 1:
输入:nums = [2,7,11,15], target = 9 输出:[0,1] 解释:因为 nums[0] + nums[1] == 9 ,返回 [0, 1] 。
示例 2:
输入:nums = [3,2,4], target = 6 输出:[1,2]
示例 3:
输入:nums = [3,3], target = 6 输出:[0,1]
提示:
2 <= nums.length <= 10^4-10^9 <= nums[i] <= 10^9-10^9 <= target <= 10^9- 只会存在一个有效答案
#include <iostream>
#include <vector>
#include <map>
using namespace std;
vector<int> twoSum(vector<int>& nums, int target) {
map<int, int> map;
vector<int> result;
for (int i = 0; i < nums.size(); i++) {
int complement = target - nums[i]; // 差值
if (map.find(complement) != map.end()) {
result.push_back(map[complement]);
result.push_back(i);
return result;
}
map[nums[i]] = i;
}
return result;
}
int main() {
vector<int> nums;
int target;
int temp;
while(cin>>temp){
nums.push_back(temp);
if(cin.get() == '\n')
break;
}
cin>>target;
vector<int> result = twoSum(nums, target);
for (int i = 0; i < result.size(); i++) {
cout << result[i] << " ";
}
return 0;
}
2. 两数相加
给你两个 非空 的链表,表示两个非负的整数。它们每位数字都是按照 逆序 的方式存储的,并且每个节点只能存储 一位 数字。
请你将两个数相加,并以相同形式返回一个表示和的链表。
你可以假设除了数字 0 之外,这两个数都不会以 0 开头。
示例 1:

输入:l1 = [2,4,3], l2 = [5,6,4] 输出:[7,0,8] 解释:342 + 465 = 807.
示例 2:
输入:l1 = [0], l2 = [0] 输出:[0]
示例 3:
输入:l1 = [9,9,9,9,9,9,9], l2 = [9,9,9,9] 输出:[8,9,9,9,0,0,0,1]
提示:
- 每个链表中的节点数在范围
[1, 100]内 0 <= Node.val <= 9- 题目数据保证列表表示的数字不含前导零
#include<iostream>
using namespace std;
struct ListNode {
int val;
ListNode *next;
ListNode() : val(0), next(NULL) {}
ListNode(int x) : val(x), next(NULL) {}
ListNode(int x, ListNode *next) : val(x), next(next) {}
};
class Solution {
public:
ListNode* addTwoNumbers(ListNode* l1, ListNode* l2) {
ListNode* dummy = new ListNode(0);
ListNode* curr = dummy;
int carry = 0; // 进位
while(l1 != NULL || l2 != NULL) {
int x = (l1 != NULL) ? l1->val : 0;
int y = (l2 != NULL) ? l2->val : 0;
int sum = x + y + carry;
carry = sum / 10;
curr->next = new ListNode(sum % 10);
curr = curr->next;
if(l1 != NULL) l1 = l1->next;
if(l2 != NULL) l2 = l2->next;
}
// 增添1位
if(carry > 0) {
curr->next = new ListNode(carry);
}
return dummy->next;
}
};
ListNode* createList() {
ListNode* head = NULL;
ListNode* current = NULL;
int val;
while(cin >> val) {
ListNode* newNode = new ListNode(val);
if(head == NULL) {
head = newNode;
current = newNode;
} else {
current->next = newNode;
current = current->next;
}
if(cin.get() == '\n')
break;
}
return head;
}
void printList(ListNode* head) {
ListNode* current = head;
while(current != NULL) {
cout << current->val << " ";
current = current->next;
}
}
int main() {
Solution solution;
ListNode* list1 = createList();
ListNode* list2 = createList();
ListNode* result = solution.addTwoNumbers(list1, list2);
printList(result);
return 0;
}3. 无重复字符的最长子串
给定一个字符串 s ,请你找出其中不含有重复字符的 最长子串 的长度。
示例 1:
输入: s = "abcabcbb" 输出: 3 解释: 因为无重复字符的最长子串是 "abc",所以其长度为 3。
示例 2:
输入: s = "bbbbb" 输出: 1 解释: 因为无重复字符的最长子串是 "b",所以其长度为 1。
示例 3:
输入: s = "pwwkew" 输出: 3 解释: 因为无重复字符的最长子串是 "wke",所以其长度为 3。请注意,你的答案必须是子串的长度,"pwke"是一个子序列,不是子串。
提示:
0 <= s.length <= 5 * 10^4s由英文字母、数字、符号和空格组成
#include<iostream>
#include<vector>
using namespace std;
class Solution {
public:
int lengthOfLongestSubstring(string s) {
// 使用滑动窗口来找出最长的不含重复字符的子串
int n = s.length();
int res = 0; // 记录最长子串的长度
vector<int> index(128, -1); // 记录每个字符在字符串s中最近出现的位置
for(int i = 0, j = 0; j < n; j++) {
i = max(i, index[s[j]] + 1); // 当遇到重复的字符时,将左边界i移动到该重复字符的下一个位置,确保窗口内没有重复字符
res = max(res, j - i + 1); // 每次更新窗口长度并与res比较,取较大者
index[s[j]] = j; // 更新字符在index数组中的位置为当前位置
}
return res;
}
};
int main() {
Solution solution;
string s;
cin >> s;
cout << solution.lengthOfLongestSubstring(s);
return 0;
}4. 寻找两个正序数组的中位数
给定两个大小分别为 m 和 n 的正序(从小到大)数组 nums1 和 nums2。请你找出并返回这两个正序数组的 中位数 。
算法的时间复杂度应该为 O(log (m+n)) 。
示例 1:
输入:nums1 = [1,3], nums2 = [2] 输出:2.00000 解释:合并数组 = [1,2,3] ,中位数 2
示例 2:
输入:nums1 = [1,2], nums2 = [3,4] 输出:2.50000 解释:合并数组 = [1,2,3,4] ,中位数 (2 + 3) / 2 = 2.5 ^
提示:
nums1.length == mnums2.length == n0 <= m <= 10000 <= n <= 10001 <= m + n <= 2000-10^6 <= nums1[i], nums2[i] <= 10^6
解法一:
#include<iostream>
#include<algorithm>
#include<vector>
using namespace std;
class Solution {
public:
double findMedianSortedArrays(vector<int>& nums1, vector<int>& nums2) {
vector<int> curr;
int m = nums1.size();
int n = nums2.size();
for(int i = 0; i < m; i++) {
curr.push_back(nums1[i]);
}
for(int i = 0; i < n; i++) {
curr.push_back(nums2[i]);
}
sort(curr.begin(), curr.end());
if((m + n) % 2 == 0) {
return (curr[(m + n) / 2] + curr[(m + n) / 2 - 1] ) / 2.0;
} else {
return curr[(m + n) / 2];
}
}
};
int main() {
Solution solution;
vector<int> nums1, nums2;
int tmp;
cout << "请输入nums1数组的元素:" << endl;
while(cin >> tmp) {
nums1.push_back(tmp);
if(cin.get() == '\n')
break;
}
cout << "请输入nums2数组的元素:" << endl;
while(cin >> tmp) {
nums2.push_back(tmp);
if(cin.get() == '\n')
break;
}
cout << solution.findMedianSortedArrays(nums1, nums2);
return 0;
}解法二:
class Solution {
public:
double findMedianSortedArrays(vector<int>& nums1, vector<int>& nums2) {
vector<int> curr;
int m = nums1.size();
int n = nums2.size();
int i = 0, j = 0;
while(i < m && j < n) {
if(nums1[i] < nums2[j]) {
curr.push_back(nums1[i]);
i++;
}
else {
curr.push_back(nums2[j]);
j++;
}
}
while(i < m) {
curr.push_back(nums1[i]);
i++;
}
while(j < n) {
curr.push_back(nums2[j]);
j++;
}
if((m + n) % 2 == 0) {
return (curr[(m + n) / 2] + curr[(m + n) / 2 - 1] ) / 2.0;
} else {
return curr[(m + n) / 2];
}
}
};5. 最长回文子串
给你一个字符串 s,找到 s 中最长的回文子串。
如果字符串的反序与原始字符串相同,则该字符串称为回文字符串。
示例 1:
输入:s = "babad" 输出:"bab" 解释:"aba" 同样是符合题意的答案。
示例 2:
输入:s = "cbbd" 输出:"bb"
提示:
1 <= s.length <= 1000s仅由数字和英文字母组成
// C++
#include<iostream>
#include<vector>
#include<string>
using namespace std;
class Solution {
public:
string longestPalindrome(string s) {
int n = s.size();
if(n < 2) {
return s;
}
int maxLen = 1;
int begin = 0;
//dp[i][j]表示s[i··j]是否为回文串
vector<vector <int> > dp(n,vector<int>(n, true));
//递归开始
//先枚举子串长度
for(int L = 2; L <= n; L++) {
//枚举左边界,左边界的上限设置可以宽松一些
for(int i = 0; i < n; i++) {
int j = L + i - 1;
//如果右边界越界,就可以退出当前循环
if(j >= n) {
break;
}
if(s[i] != s[j]) {
dp[i][j] = false;
} else {
if(j-i < 3) {
dp[i][j] = true;
} else {
dp[i][j] = dp[i + 1][j - 1];
}
}
// 只要dp[i][j] == true成立,就表示子串s[i···L]是回文,此时记录回文长度和起始位置。
if(dp[i][j] && j - i + 1 > maxLen) {
maxLen = j - i + 1;
begin = i;
}
}
}
return s.substr(begin,maxLen);
}
};
int main() {
string s = "";
cin>>s;
Solution solution;
cout<<solution.longestPalindrome(s)<<endl;
return 0;
}解法二:
// C++
#include<iostream>
#include<vector>
#include<string>
using namespace std;
class Solution {
public:
string longestPalindrome(string s) {
if(s.empty()) {
return "";
}
int start = 0, end = 0;
for(int i = 0; i < s.size(); i++) {
int len1 = expandAroundCenter(s, i, i);
int len2 = expandAroundCenter(s, i, i + 1);
int len = max(len1, len2);
if(len > end - start) {
start = i - (len - 1) / 2;
end = i + len / 2;
}
}
return s.substr(start, end - start + 1);
}
int expandAroundCenter(string s, int left, int right) {
while(left >= 0 && right < s.size() && s[left] == s[right]) {
left--;
right++;
}
return right - left - 1;
}
};
int main() {
string s = "";
cin>>s;
Solution solution;
cout<<solution.longestPalindrome(s)<<endl;
return 0;
}6. Z字型变换
将一个给定字符串 s 根据给定的行数 numRows ,以从上往下、从左到右进行 Z 字形排列。
比如输入字符串为 "PAYPALISHIRING" 行数为 3 时,排列如下:
P A H N A P L S I I G Y I R
之后,你的输出需要从左往右逐行读取,产生出一个新的字符串,比如:"PAHNAPLSIIGYIR"。
请你实现这个将字符串进行指定行数变换的函数:
string convert(string s, int numRows);
示例 1:
输入:s = "PAYPALISHIRING", numRows = 3 输出:"PAHNAPLSIIGYIR"
示例 2:
输入:s = "PAYPALISHIRING", numRows = 4 输出:"PINALSIGYAHRPI" 解释: P I N A L S I G Y A H R P I
示例 3:
输入:s = "A", numRows = 1 输出:"A"
提示:
1 <= s.length <= 1000s由英文字母(小写和大写)、','和'.'组成1 <= numRows <= 1000
#include<iostream>
#include<vector>
#include<algorithm>
using namespace std;
class Solution {
public:
string covert(string s, int numRows) {
if(numRows == 1) return s;
int n = s.size();
int k = 2 * numRows - 2; // 计算 Z 字形的周期长度,即每个完整的 Z 字形有多少个字符
vector<string> a(numRows);
// 创建一个长度为 numRows 的字符串数组 a,用于存储 Z 字形排列后的每一行字符串
for(int i = 0; i < n; i++) {
a[min(k - i % k, i % k)] += s[i];
// k - i % k 和 i % k 分别表示当前字符在 Z 字形周期中的上半部分和下半部分的位置
}
return accumulate(a.begin(), a.end(), string("")); // 拼接
}
};
int main() {
string s;
cin >> s;
int numRows;
cin >> numRows;
Solution solution;
cout << solution.covert(s, numRows);
return 0;
}
7. 整数反转
给你一个 32 位的有符号整数 x ,返回将 x 中的数字部分反转后的结果。
如果反转后整数超过 32 位的有符号整数的范围 [−2^31, 2^31 − 1] ,就返回 0。
假设环境不允许存储 64 位整数(有符号或无符号)。
示例 1:
输入:x = 123 输出:321
示例 2:
输入:x = -123 输出:-321
示例 3:
输入:x = 120 输出:21
示例 4:
输入:x = 0 输出:0
提示:
-2^31 <= x <= 2^31 - 1
#include<iostream>
#include<vector>
using namespace std;
class Solution {
public:
int reverse(int x) {
// if(x == 0) return 0;
int res = 0;
while(x != 0) {
if(res < INT_MIN / 10 || res > INT_MAX / 10) {
return 0;
}
res = res * 10 + x % 10;
x /= 10;
}
return res;
}
};
int main() {
int x;
cin >> x;
Solution solution;
cout << solution.reverse(x) << endl;
return 0;
}8. 字符串转换正数(atoi)
请你来实现一个 myAtoi(string s) 函数,使其能将字符串转换成一个 32 位有符号整数(类似 C/C++ 中的 atoi 函数)。
函数 myAtoi(string s) 的算法如下:
- 读入字符串并丢弃无用的前导空格
- 检查下一个字符(假设还未到字符末尾)为正还是负号,读取该字符(如果有)。 确定最终结果是负数还是正数。 如果两者都不存在,则假定结果为正。
- 读入下一个字符,直到到达下一个非数字字符或到达输入的结尾。字符串的其余部分将被忽略。
- 将前面步骤读入的这些数字转换为整数(即,"123" -> 123, "0032" -> 32)。如果没有读入数字,则整数为
0。必要时更改符号(从步骤 2 开始)。 - 如果整数数超过 32 位有符号整数范围
[−231, 231 − 1],需要截断这个整数,使其保持在这个范围内。具体来说,小于−231的整数应该被固定为−231,大于231 − 1的整数应该被固定为231 − 1。 - 返回整数作为最终结果。
注意:
- 本题中的空白字符只包括空格字符
' '。 - 除前导空格或数字后的其余字符串外,请勿忽略 任何其他字符。
示例 1:
输入:s = "42"
输出:42
解释:加粗的字符串为已经读入的字符,插入符号是当前读取的字符。
第 1 步:"42"(当前没有读入字符,因为没有前导空格)
^
第 2 步:"42"(当前没有读入字符,因为这里不存在 '-' 或者 '+')
^
第 3 步:"42"(读入 "42")
^
解析得到整数 42 。
由于 "42" 在范围 [-231, 231 - 1] 内,最终结果为 42 。
示例 2:
输入:s = " -42"
输出:-42
解释:
第 1 步:" -42"(读入前导空格,但忽视掉)
^
第 2 步:" -42"(读入 '-' 字符,所以结果应该是负数)
^
第 3 步:" -42"(读入 "42")
^
解析得到整数 -42 。
由于 "-42" 在范围 [-231, 231 - 1] 内,最终结果为 -42 。
示例 3:
输入:s = "4193 with words"
输出:4193
解释:
第 1 步:"4193 with words"(当前没有读入字符,因为没有前导空格)
^
第 2 步:"4193 with words"(当前没有读入字符,因为这里不存在 '-' 或者 '+')
^
第 3 步:"4193 with words"(读入 "4193";由于下一个字符不是一个数字,所以读入停止)
^
解析得到整数 4193 。
由于 "4193" 在范围 [-231, 231 - 1] 内,最终结果为 4193 。
提示:
0 <= s.length <= 200s由英文字母(大写和小写)、数字(0-9)、' '、'+'、'-'和'.'组成
#include<iostream>
#include<ctype.h>
using namespace std;
class Solution {
public:
int myAtoi(string s) {
int i = 0; // 索引
int sign = 1; // 符号位
long long result = 0;
// 跳过前面的空格字符
while(i < s.size() && s[i] == ' ') {
i++;
}
// 检查最终结果为正数还是负数
if(i < s.size() && (s[i] == '+' || s[i] == '-')) {
sign = (s[i++] == '-' ? -1 : 1);
}
// 把字符串转换成数字
while(i < s.size() && isdigit(s[i])) {
result = result * 10 + (s[i++] - '0');
if(result * sign > INT_MAX) {
return INT_MAX;
}
if(result * sign < INT_MIN) {
return INT_MIN;
}
}
return result * sign;
}
};
int main() {
string s;
cin >> s;
Solution solution;
cout << solution.myAtoi(s);
return 0;
}
9. 回文数
给你一个整数 x ,如果 x 是一个回文整数,返回 true ;否则,返回 false 。
回文数是指正序(从左向右)和倒序(从右向左)读都是一样的整数。
- 例如,
121是回文,而123不是。
示例 1:
输入:x = 121 输出:true
示例 2:
输入:x = -121 输出:false 解释:从左向右读, 为 -121 。 从右向左读, 为 121- 。因此它不是一个回文数。
示例 3:
输入:x = 10 输出:false 解释:从右向左读, 为 01 。因此它不是一个回文数。
提示:
-2^31 <= x <= 2^31 - 1
#include<iostream>
using namespace std;
bool isPalindrome(int num){
if(num < 0){
return false;
}
int original = num;
long long reversed = 0;
while(num > 0) {
reversed = reversed * 10 + num % 10;
num /= 10;
}
return original == reversed;
}
int main()
{
int num;
cin>>num;
cout<<boolalpha<<isPalindrome(num)<<endl;
return 0;
}10. 正则表达式匹配
给你一个字符串 s 和一个字符规律 p,请你来实现一个支持 '.' 和 '*' 的正则表达式匹配。
'.'匹配任意单个字符'*'匹配零个或多个前面的那一个元素
所谓匹配,是要涵盖 整个 字符串 s的,而不是部分字符串。
示例 1:
输入:s = "aa", p = "a" 输出:false 解释:"a" 无法匹配 "aa" 整个字符串。
示例 2:
输入:s = "aa", p = "a*" 输出:true 解释:因为 '*' 代表可以匹配零个或多个前面的那一个元素, 在这里前面的元素就是 'a'。因此,字符串 "aa" 可被视为 'a' 重复了一次。
示例 3:
输入:s = "ab", p = ".*" 输出:true 解释:".*" 表示可匹配零个或多个('*')任意字符('.')。
提示:
1 <= s.length <= 201 <= p.length <= 20s只包含从a-z的小写字母。p只包含从a-z的小写字母,以及字符.和*。- 保证每次出现字符
*时,前面都匹配到有效的字符
#include<iostream>
#include<vector>
using namespace std;
class Solution {
public:
bool isMatch(string s, string p) {
int m = s.size();
int n = p.size();
vector<vector<bool>> dp(m + 1, vector<bool>(n + 1, false));
dp[0][0] = true;
/*
使用两层循环遍历dp数组,其中i表示s的长度,j表示p的长度。
在内层循环中,分情况讨论:
如果p[j-1]为'',则dp[i][j]为dp[i][j-2](''匹配0次)或者(s的第i个字符和p的第j-1个字符匹配,
并且dp[i-1][j]为true)。
如果p[j-1]不为'*',则dp[i][j]为dp[i-1][j-1](s的第i个字符和p的第j个字符匹配)。
*/
for (int i = 0; i <= m; i++) {
for (int j = 1; j <= n; j++) {
if (p[j - 1] == '*') {
dp[i][j] = dp[i][j - 2] || (i > 0 && (s[i - 1] == p[j - 2] || p[j - 2] == '.') && dp[i - 1][j]);
} else {
dp[i][j] = i > 0 && dp[i - 1][j - 1] && (s[i - 1] == p[j - 1] || p[j - 1] == '.');
}
}
}
return dp[m][n];
}
};
int main() {
string s, p;
cin >> s >> p;
Solution solution;
cout << boolalpha << solution.isMatch(s, p);
return 0;
}11. 盛最多的水
给定一个长度为 n 的整数数组 height 。有 n 条垂线,第 i 条线的两个端点是 (i, 0) 和 (i, height[i]) 。
找出其中的两条线,使得它们与 x 轴共同构成的容器可以容纳最多的水。
返回容器可以储存的最大水量。
说明:你不能倾斜容器。
示例 1:
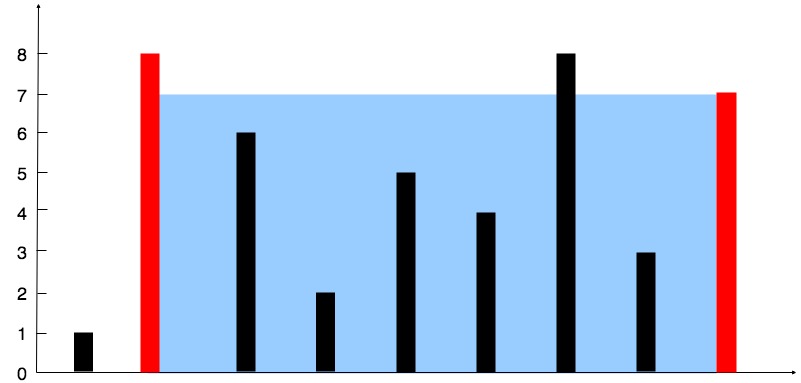
输入:[1,8,6,2,5,4,8,3,7] 输出:49 解释:图中垂直线代表输入数组 [1,8,6,2,5,4,8,3,7]。在此情况下,容器能够容纳水(表示为蓝色部分)的最大值为 49。
示例 2:
输入:height = [1,1] 输出:1
提示:
n == height.length2 <= n <= 10^50 <= height[i] <= 10^4
#include<iostream>
#include<vector>
using namespace std;
class Solution {
public:
int maxArea(vector<int>& height) {
int n = height.size();
int left = 0;
int right = n - 1;
int result = 0;
while(left < right) {
result = max(result, (right - left) * min(height[left], height[right]));
if(height[left] > height[right]) {
--right;
} else {
++left;
}
}
return result;
}
};
int main() {
Solution solution;
vector<int> height;
int tmp;
while(cin >> tmp) {
height.push_back(tmp);
if(cin.get() == '\n')
break;
}
cout << solution.maxArea(height);
return 0;
}12. 整数转罗马数字
罗马数字包含以下七种字符: I, V, X, L,C,D 和 M。
字符 数值 I 1 V 5 X 10 L 50 C 100 D 500 M 1000
例如, 罗马数字 2 写做 II ,即为两个并列的 1。12 写做 XII ,即为 X + II 。 27 写做 XXVII, 即为 XX + V + II 。
通常情况下,罗马数字中小的数字在大的数字的右边。但也存在特例,例如 4 不写做 IIII,而是 IV。数字 1 在数字 5 的左边,所表示的数等于大数 5 减小数 1 得到的数值 4 。同样地,数字 9 表示为 IX。这个特殊的规则只适用于以下六种情况:
I可以放在V(5) 和X(10) 的左边,来表示 4 和 9。X可以放在L(50) 和C(100) 的左边,来表示 40 和 90。C可以放在D(500) 和M(1000) 的左边,来表示 400 和 900。
给你一个整数,将其转为罗马数字。
示例 1:
输入: num = 3 输出: "III"
示例 2:
输入: num = 4 输出: "IV"
示例 3:
输入: num = 9 输出: "IX"
示例 4:
输入: num = 58 输出: "LVIII" 解释: L = 50, V = 5, III = 3.
示例 5:
输入: num = 1994 输出: "MCMXCIV" 解释: M = 1000, CM = 900, XC = 90, IV = 4.
提示:
1 <= num <= 3999
解法一:
#include<iostream>
#include<map>
#include<vector>
using namespace std;
class Solution {
public:
string intToRoman(int num) {
vector<int> values = {1000, 900, 500, 400, 100, 90, 50, 40, 10, 9, 5, 4, 1};
vector<string> symbols = {"M", "CM", "D", "CD", "C", "XC", "L", "XL", "X", "IX", "V", "IV", "I"};
string result = "";
for(int i = 0; i < values.size(); i++) {
while(num >= values[i]) {
num -= values[i];
result += symbols[i];
}
}
return result;
}
};
int main() {
Solution solution;
int num;
cin >> num;
cout << solution.intToRoman(num);
return 0;
}解法二:
#include<iostream>
#include<map>
#include<vector>
using namespace std;
class Solution {
public:
string intToRoman(int num) {
map<int, string> romanMap;
romanMap[1] = "I";
romanMap[4] = "IV";
romanMap[5] = "V";
romanMap[9] = "IX";
romanMap[10] = "X";
romanMap[40] = "XL";
romanMap[50] = "L";
romanMap[90] = "XC";
romanMap[100] = "C";
romanMap[400] = "CD";
romanMap[500] = "D";
romanMap[900] = "CM";
romanMap[1000] = "M";
string result = "";
for(auto it = romanMap.rbegin(); it != romanMap.rend(); ++it) {
while(num >= it->first) {
result += it->second;
num -= it->first;
}
}
return result;
}
};
int main() {
Solution solution;
int num;
cin >> num;
cout << solution.intToRoman(num);
return 0;
}13. 罗马数字转整数
罗马数字包含以下七种字符: I, V, X, L,C,D 和 M。
字符 数值 I 1 V 5 X 10 L 50 C 100 D 500 M 1000
例如, 罗马数字 2 写做 II ,即为两个并列的 1 。12 写做 XII ,即为 X + II 。 27 写做 XXVII, 即为 XX + V + II 。
通常情况下,罗马数字中小的数字在大的数字的右边。但也存在特例,例如 4 不写做 IIII,而是 IV。数字 1 在数字 5 的左边,所表示的数等于大数 5 减小数 1 得到的数值 4 。同样地,数字 9 表示为 IX。这个特殊的规则只适用于以下六种情况:
I可以放在V(5) 和X(10) 的左边,来表示 4 和 9。X可以放在L(50) 和C(100) 的左边,来表示 40 和 90。C可以放在D(500) 和M(1000) 的左边,来表示 400 和 900。
给定一个罗马数字,将其转换成整数。
示例 1:
输入: s = "III" 输出: 3
示例 2:
输入: s = "IV" 输出: 4
示例 3:
输入: s = "IX" 输出: 9
示例 4:
输入: s = "LVIII" 输出: 58 解释: L = 50, V= 5, III = 3.
示例 5:
输入: s = "MCMXCIV" 输出: 1994 解释: M = 1000, CM = 900, XC = 90, IV = 4.
提示:
1 <= s.length <= 15s仅含字符('I', 'V', 'X', 'L', 'C', 'D', 'M')- 题目数据保证
s是一个有效的罗马数字,且表示整数在范围[1, 3999]内 - 题目所给测试用例皆符合罗马数字书写规则,不会出现跨位等情况。
- IL 和 IM 这样的例子并不符合题目要求,49 应该写作 XLIX,999 应该写作 CMXCIX 。
- 关于罗马数字的详尽书写规则,可以参考 罗马数字 - Mathematics 。
#include<iostream>
#include<map>
using namespace std;
int romanToInt(string s){
map<char, int> romanMap;
romanMap['I'] = 1;
romanMap['V'] = 5;
romanMap['X'] = 10;
romanMap['L'] = 50;
romanMap['C'] = 100;
romanMap['D'] = 500;
romanMap['M'] = 1000;
int result = 0;
for(int i = 0; i < s.length(); i++) {
if(i < s.length() - 1 && romanMap[s[i]] < romanMap[s[i + 1]]) {
result -= romanMap[s[i]];
} else {
result += romanMap[s[i]];
}
}
return result;
}
int main(){
string romanNum;
cin>>romanNum;
cout<<romanToInt(romanNum);
return 0;
} 14. 最长公共前缀
编写一个函数来查找字符串数组中的最长公共前缀。
如果不存在公共前缀,返回空字符串 ""。
示例 1:
输入:strs = ["flower","flow","flight"] 输出:"fl"
示例 2:
输入:strs = ["dog","racecar","car"] 输出:"" 解释:输入不存在公共前缀。
提示:
1 <= strs.length <= 2000 <= strs[i].length <= 200strs[i]仅由小写英文字母组成
#include<iostream>
#include<vector>
#include<algorithm>
using namespace std;
string longestCommonPrefix(vector<string>& strs) {
if(strs.empty()) {
return "";
}
string ans = strs[0];
for(int i = 1; i < strs.size(); i++) {
int j = 0;
for(; j < ans.length() && j < strs[i].length(); j++) {
if(ans[j] != strs[i][j])
break;
}
ans = ans.substr(0, j);
if(ans == "")
return ans;
}
return ans;
}
int main() {
vector<string> strs;
string tmp;
while(cin>>tmp) {
strs.push_back(tmp);
if(cin.get() == '\n')
break;
}
cout<<longestCommonPrefix(strs)<<endl;
return 0;
} 15. 三数之和
给你一个整数数组 nums ,判断是否存在三元组 [nums[i], nums[j], nums[k]] 满足 i != j、i != k 且 j != k ,同时还满足 nums[i] + nums[j] + nums[k] == 0 。请
你返回所有和为 0 且不重复的三元组。
注意:答案中不可以包含重复的三元组。
示例 1:
输入:nums = [-1,0,1,2,-1,-4] 输出:[[-1,-1,2],[-1,0,1]] 解释: nums[0] + nums[1] + nums[2] = (-1) + 0 + 1 = 0 。 nums[1] + nums[2] + nums[4] = 0 + 1 + (-1) = 0 。 nums[0] + nums[3] + nums[4] = (-1) + 2 + (-1) = 0 。 不同的三元组是 [-1,0,1] 和 [-1,-1,2] 。 注意,输出的顺序和三元组的顺序并不重要。
示例 2:
输入:nums = [0,1,1] 输出:[] 解释:唯一可能的三元组和不为 0 。
示例 3:
输入:nums = [0,0,0] 输出:[[0,0,0]] 解释:唯一可能的三元组和为 0 。
提示:
3 <= nums.length <= 3000-10^5 <= nums[i] <= 10^5
#include<iostream>
#include<vector>
#include<algorithm>
using namespace std;
class Solution {
public:
vector<vector<int> > threeSum(vector<int>& nums) {
vector<vector<int> > result;
sort(nums.begin(), nums.end());
for(int i = 0; i < nums.size(); i++) {
if(i > 0 && nums[i] == nums[i - 1]) {
continue; // 重复
}
int target = -nums[i];
int left = i + 1;
int right = nums.size() - 1;
while(left < right) {
int sum = nums[left] + nums[right];
if(sum < target) { // 小于0
left++;
} else if(sum > target) { // 大于0
right--;
} else { // 等于0
result.push_back({nums[i], nums[left], nums[right]});
while(left < right && nums[left] == nums[left + 2]) {
left++;
}
while(left < right &&nums[right] == nums[right - 1]) {
right--;
}
left++;
right--;
}
}
}
return result;
}
};
int main() {
int tmp;
vector<int> nums;
while(cin >> tmp) {
nums.push_back(tmp);
if(cin.get() == '\n')
break;
}
Solution solution;
vector<vector<int>> result = solution.threeSum(nums);
// for(const auto& triplet : result) {
// cout << "[";
// for(int i = 0; i < 3; i++) {
// cout << triplet[i];
// if(i < 2) {
// cout << ", ";
// }
// }
// cout << "] ";
// }
cout << "[";
for(vector<vector<int> >::iterator it = result.begin(); it != result.end(); ++it) {
cout << "[";
for(int i = 0; i < 3; ++i) {
cout << (*it)[i];
if(i < 2) {
cout << ",";
}
}
if(it == result.end() - 1) {
cout << "]";
} else {
cout << "], ";
}
}
cout << "]";
return 0;
} 16. 最接近的三数之和
给你一个长度为 n 的整数数组 nums 和 一个目标值 target。请你从 nums 中选出三个整数,使它们的和与 target 最接近。
返回这三个数的和。
假定每组输入只存在恰好一个解。
示例 1:
输入:nums = [-1,2,1,-4], target = 1 输出:2 解释:与 target 最接近的和是 2 (-1 + 2 + 1 = 2) 。
示例 2:
输入:nums = [0,0,0], target = 1 输出:0
提示:
3 <= nums.length <= 1000-1000 <= nums[i] <= 1000-10^4 <= target <= 10^4
#include<iostream>
#include<vector>
#include<algorithm>
#include<climits>
using namespace std;
class Solution {
public:
int threeSumClosest(vector<int>& nums, int target) {
sort(nums.begin(), nums.end());
int closestSum = INT_MAX;
int minDiff = INT_MAX;
for(int i = 0; i < nums.size() - 2; i++) {
int left = i + 1;
int right = nums.size() - 1;
while(left < right) {
int sum = nums[i] + nums[left] + nums[right];
int diff = abs(sum - target);
if(diff < minDiff) { // 更新最小差值
minDiff = diff;
closestSum = sum;
}
if(sum < target) {
++left; // 偏小
} else if(sum > target) {
--right; // 偏大
} else {
return sum; // 相等
}
}
}
return closestSum;
}
};
int main() {
int target;
vector<int> nums;
while(cin >> target) {
nums.push_back(target);
if(cin.get() == '\n')
break;
}
cin >> target;
Solution solution;
cout << solution.threeSumClosest(nums, target);
return 0;
}17. 电话号码的字母组合
给定一个仅包含数字 2-9 的字符串,返回所有它能表示的字母组合。答案可以按 任意顺序 返回。
给出数字到字母的映射如下(与电话按键相同)。注意 1 不对应任何字母。

示例 1:
输入:digits = "23" 输出:["ad","ae","af","bd","be","bf","cd","ce","cf"]
示例 2:
输入:digits = "" 输出:[]
示例 3:
输入:digits = "2" 输出:["a","b","c"]
提示:
0 <= digits.length <= 4digits[i]是范围['2', '9']的一个数字。
#include <iostream>
#include <vector>
#include <string>
#include <unordered_map>
using namespace std;
class Solution {
public:
vector<string> letterCombinations(string digits) {
vector<string> result;
if (digits.empty()) {
return result;
}
unordered_map<char, string> digitToLetters = {
{'2', "abc"},
{'3', "def"},
{'4', "ghi"},
{'5', "jkl"},
{'6', "mno"},
{'7', "pqrs"},
{'8', "tuv"},
{'9', "wxyz"}
};
string current;
backtrack(digits, 0, current, result, digitToLetters);
return result;
}
void backtrack(string& digits, int index, string& current, vector<string>& result, unordered_map<char, string>& digitToLetters) {
if (index == digits.length()) {
result.push_back(current);
return;
}
char digit = digits[index];
string letters = digitToLetters[digit];
for (char letter : letters) {
current.push_back(letter);
backtrack(digits, index + 1, current, result, digitToLetters);
current.pop_back();
}
}
};
int main() {
string digits;
cin >> digits;
Solution solution;
vector<string> result = solution.letterCombinations(digits);
for (string& combination : result) {
cout << combination << " ";
}
return 0;
}
18. 四数之和
给你一个由 n 个整数组成的数组 nums ,和一个目标值 target 。请你找出并返回满足下述全部条件且不重复的四元组 [nums[a], nums[b], nums[c], nums[d]] (若两个四元组元素一一对应,则认为两个四元组重复):
0 <= a, b, c, d < na、b、c和d互不相同nums[a] + nums[b] + nums[c] + nums[d] == target
你可以按 任意顺序 返回答案 。
示例 1:
输入:nums = [1,0,-1,0,-2,2], target = 0 输出:[[-2,-1,1,2],[-2,0,0,2],[-1,0,0,1]]
示例 2:
输入:nums = [2,2,2,2,2], target = 8 输出:[[2,2,2,2]]
提示:
1 <= nums.length <= 200-10^9 <= nums[i] <= 10^9-10^9 <= target <= 10^9
#include<iostream>
#include<vector>
#include<algorithm>
using namespace std;
class Solution {
public:
vector<vector<int>> fourSum(vector<int>& nums, int target) {
vector<vector<int>> result;
int n = nums.size();
if(n < 4) {
return result;
}
sort(nums.begin(), nums.end());
for(int i = 0; i < n - 3; i++) {
if(i > 0 && nums[i] == nums[i - 1]) {
continue;
}
for(int j = i + 1; j < n - 2; j++) {
if(j > i + 1 && nums[j] == nums[j - 1]) {
continue;
}
int left = j + 1;
int right = n - 1;
while(left < right) {
long sum = static_cast<long>(nums[i]) + nums[j] + nums[left] + nums[right];
if(sum < target) {
++left;
} else if(sum > target) {
--right;
} else {
result.push_back({nums[i], nums[j], nums[left], nums[right]});
while(left < right && nums[left] == nums[left + 1]) {
++left;
}
while(left < right && nums[right] == nums[right - 1]) {
--right;
}
++left;
--right;
}
}
}
}
return result;
}
};
int main() {
int target;
vector<int> nums;
while(cin >> target) {
nums.push_back(target);
if(cin.get() == '\n')
break;
}
cin >> target;
Solution solution;
vector<vector<int>> result = solution.fourSum(nums, target);
for(vector<int>& quadruplet : result) {
cout << "[";
for(int num : quadruplet) {
cout << num << " ";
}
cout << "] ";
}
return 0;
}19. 删除链表的倒数第N个结点
给你一个链表,删除链表的倒数第 n 个结点,并且返回链表的头结点。
示例 1:
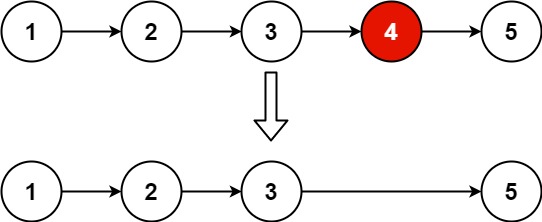
输入:head = [1,2,3,4,5], n = 2 输出:[1,2,3,5]
示例 2:
输入:head = [1], n = 1 输出:[]
示例 3:
输入:head = [1,2], n = 1 输出:[1]
提示:
- 链表中结点的数目为
sz 1 <= sz <= 300 <= Node.val <= 1001 <= n <= sz
进阶:你能尝试使用一趟扫描实现吗?
解题思路:要删除链表的倒数第n个结点,可以使用双指针技巧。首先定义两个指针,分别为快指针和慢指针,初始时均指向头节点。然后让快指针先向后移动n + 1步,这样快指针和慢指针之间就会相隔n个节点。接着同时移动快指针和慢指针,直到快指针指向链表末尾。此时慢指针指向的节点就是要删除的节点的前一个结点。
#include<iostream>
using namespace std;
// Definition for single-linked list
struct ListNode {
int val;
ListNode *next;
ListNode() : val(0), next(NULL) {}
ListNode(int x) : val(x), next(NULL) {}
ListNode(int x, ListNode *next) : val(x), next(next) {}
};
class Solution {
public:
ListNode* removeNthFromEnd(ListNode* head, int n) {
ListNode* dummy = new ListNode(0);
dummy->next = head;
ListNode* fast = dummy;
ListNode* slow = dummy;
// 向后移动n + 1步
for(int i = 0; i < n + 1; i++) {
fast = fast->next;
}
while(fast != NULL) {
fast = fast->next;
slow = slow->next;
}
// 删除结点
ListNode * q = slow->next;
slow->next = slow->next->next;
delete q;
return dummy->next;
}
};
ListNode* createList() {
ListNode* head = NULL;
ListNode* current = NULL;
int val;
while(cin >> val) {
ListNode* newNode = new ListNode(val);
if(head == NULL) {
head = newNode;
current = newNode;
} else {
current->next = newNode;
current = newNode;
}
if(cin.get() == '\n')
break;
}
return head;
}
void printList(ListNode* head) {
ListNode* current = head;
while(current != NULL) {
cout << current->val << " ";
current = current->next;
}
}
int main() {
ListNode* head = createList();
int n;
cin >> n;
Solution solution;
ListNode* result = solution.removeNthFromEnd(head, n);
printList(result);
return 0;
}20. 有效的括号
给定一个只包括 '(',')','{','}','[',']' 的字符串 s ,判断字符串是否有效。
有效字符串需满足:
- 左括号必须用相同类型的右括号闭合。
- 左括号必须以正确的顺序闭合。
- 每个右括号都有一个对应的相同类型的左括号。
示例 1:
输入:s = "()" 输出:true
示例 2:
输入:s = "()[]{}"
输出:true
示例 3:
输入:s = "(]" 输出:false
提示:
1 <= s.length <= 104s仅由括号'()[]{}'组成
#include<iostream>
#include<stack>
#include<map>
using namespace std;
bool isValid(string s) {
stack<char> stk;
map<char, char> brackets;
brackets.insert(make_pair(')', '('));
brackets.insert(make_pair('}', '{'));
brackets.insert(make_pair(']', '['));
for(string::iterator it = s.begin(); it != s.end(); ++it) {
char c = *it;
// 如果是右括号
if(brackets.find(c) != brackets.end()) {
// 栈为空或者栈顶元素与当前右括号不匹配,返回false
if(stk.empty() || stk.top() != brackets[c]) {
return false;
}
stk.pop();
} else {
stk.push(c); // 左括号
}
}
return stk.empty();
}
int main() {
string s;
cin>>s;
cout<<boolalpha<<isValid(s)<<endl;
return 0;
}21. 合并两个有序链表
将两个升序链表合并为一个新的 升序 链表并返回。新链表是通过拼接给定的两个链表的所有节点组成的。
示例 1:
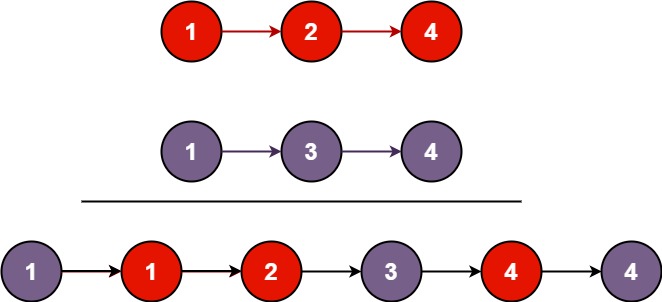
输入:l1 = [1,2,4], l2 = [1,3,4] 输出:[1,1,2,3,4,4]
示例 2:
输入:l1 = [], l2 = [] 输出:[]
示例 3:
输入:l1 = [], l2 = [0] 输出:[0]
提示:
- 两个链表的节点数目范围是
[0, 50] -100 <= Node.val <= 100l1和l2均按 非递减顺序 排列
#include<iostream>
using namespace std;
struct ListNode {
int val;
ListNode *next;
ListNode() : val(0), next(NULL) {}
ListNode(int x) : val(x), next(NULL) {}
ListNode(int x, ListNode *next) : val(x), next(next) {}
};
class Solution {
public:
ListNode* mergeTwoLists(ListNode* list1, ListNode* list2) { // 递归
if(list1 == NULL)
return list2;
if(list2 == NULL)
return list1;
if(list1->val < list2->val) {
list1->next = mergeTwoLists(list1->next, list2);
return list1;
}else {
list2->next = mergeTwoLists(list1, list2->next);
return list2;
}
}
};
int main() {
ListNode* list1 = NULL;
ListNode* list2 = NULL;
cout << "Enter elements for list1 (enter -1 to stop): ";
int val;
while (cin >> val && val != -1) {
ListNode* node = new ListNode(val);
if (list1 == NULL) {
list1 = node;
} else {
ListNode* temp = list1;
while (temp->next != NULL) {
temp = temp->next;
}
temp->next = node;
}
}
cout << "Enter elements for list2 (enter -1 to stop): ";
while (cin >> val && val != -1) {
ListNode* node = new ListNode(val);
if (list2 == NULL) {
list2 = node;
} else {
ListNode* temp = list2;
while (temp->next != NULL) {
temp = temp->next;
}
temp->next = node;
}
}
Solution solution;
ListNode* mergedList = solution.mergeTwoLists(list1, list2);
// Print the merged list
ListNode* temp = mergedList;
cout << "Merged list: ";
while (temp != NULL) {
cout << temp->val << " ";
temp = temp->next;
}
cout << endl;
return 0;
}
22. 括号生成
数字 n 代表生成括号的对数,请你设计一个函数,用于能够生成所有可能的并且 有效的 括号组合。
示例 1:
输入:n = 3 输出:["((()))","(()())","(())()","()(())","()()()"]
示例 2:
输入:n = 1 输出:["()"]
提示:
1 <= n <= 8
#include<iostream>
#include<vector>
using namespace std;
class Solution {
public:
void helper(vector<string>& result, string current, int open, int close, int n) {
if(current.size() == 2 * n) {
result.push_back(current);
return; // 递归结束
}
if(open < n) {
helper(result, current + "(", open + 1, close, n);
}
if(close < open) {
helper(result, current + ")", open, close + 1, n);
}
}
vector<string> generateParenthesis(int n) {
vector<string> result;
helper(result, "", 0, 0, n);
return result;
}
};
int main() {
int n;
cin >> n;
Solution solution;
vector<string> result = solution.generateParenthesis(n);
cout << "[";
for(auto it = result.begin(); it != result.end(); ++it) {
if(it == result.end() - 1) {
cout << *it;
} else {
cout << *it <<", ";
}
}
cout << "]";
return 0;
}23. 合并K个升序链表
给你一个链表数组,每个链表都已经按升序排列。
请你将所有链表合并到一个升序链表中,返回合并后的链表。
示例 1:
输入:lists = [[1,4,5],[1,3,4],[2,6]] 输出:[1,1,2,3,4,4,5,6] 解释:链表数组如下: [ 1->4->5, 1->3->4, 2->6 ] 将它们合并到一个有序链表中得到。 1->1->2->3->4->4->5->6
示例 2:
输入:lists = [] 输出:[]
示例 3:
输入:lists = [[]] 输出:[]
提示:
k == lists.length0 <= k <= 10^40 <= lists[i].length <= 500-10^4 <= lists[i][j] <= 10^4lists[i]按 升序 排列lists[i].length的总和不超过10^4
解题思路:要合并K个升序链表,可以使用优先队列(最小堆)来实现。首先将每个链表的头节点加入到优先队列中,然后不断从优先队列中取出最小的节点,将其加入到结果链表中,并将该节点的下一个节点加入到优先队列中。重复这个过程直到优先队列为空。
#include<iostream>
#include<vector>
#include<queue>
using namespace std;
// Definition for singly-linked list
struct ListNode {
int val;
ListNode *next;
ListNode() : val(0), next(NULL) {}
ListNode(int x) : val(x), next(NULL) {}
ListNode(int x, ListNode *next) : val(x), next(next) {}
};
struct compare {
bool operator()(ListNode* a, ListNode* b) {
return a->val > b->val;
}
};
class Solution {
public:
ListNode* mergeKLists(vector<ListNode*>& lists) {
priority_queue<ListNode*, vector<ListNode*>, compare> pq;
for(ListNode* list : lists) { // 添加各个链表的头节点
if(list) {
pq.push(list);
}
}
ListNode* dummy = new ListNode(0);
ListNode* tail = dummy;
while(!pq.empty()) {
ListNode* node = pq.top(); // 获取堆顶元素
pq.pop();
tail->next = node;
tail = tail->next;
if(node->next) {
// 把堆顶元素所在链表的下一个节点入队
pq.push(node->next);
}
}
return dummy->next;
}
};
ListNode* createList() {
ListNode* head = NULL;
ListNode* current = NULL;
int val;
while(cin >> val) {
ListNode* newNode = new ListNode(val);
if(head == NULL) {
head = newNode;
current = newNode;
} else {
current->next = newNode;
current = newNode;
}
if(cin.get() == '\n')
break;
}
return head;
}
void printList(ListNode* head) {
ListNode* current = head;
while(current != NULL) {
cout << current->val << " ";
current = current->next;
}
}
int main() {
vector<ListNode*> lists;
int flag = 0;
while(true) {
cout << "请输入链表元素:";
ListNode* head = createList();
lists.push_back(head);
cout << "是否需要继续创建链表数组:0(继续), 1(结束):";
cin >> flag;
if(flag == 1) { // flag = 1时,结束创建链表数组
break;
}
}
cout << "Merged List: ";
Solution solution;
ListNode* mergedList = solution.mergeKLists(lists);
printList(mergedList);
return 0;
}24. 两两交换链表中的节点
给你一个链表,两两交换其中相邻的节点,并返回交换后链表的头节点。你必须在不修改节点内部的值的情况下完成本题(即,只能进行节点交换)。
示例 1:
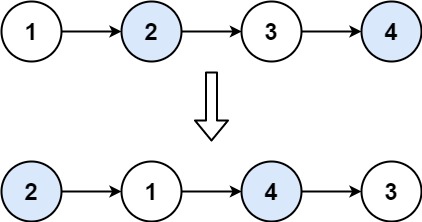
输入:head = [1,2,3,4] 输出:[2,1,4,3]
示例 2:
输入:head = [] 输出:[]
示例 3:
输入:head = [1] 输出:[1]
提示:
- 链表中节点的数目在范围
[0, 100]内 0 <= Node.val <= 100
#include<iostream>
using namespace std;
// Definition for singly-linked list
struct ListNode {
int val;
ListNode *next;
ListNode() : val(0), next(NULL) {}
ListNode(int x) : val(x), next(NULL) {}
ListNode(int x, ListNode *next) : val(x), next(next) {}
};
class Solution {
public:
ListNode* swapPairs(ListNode* head) {
if(!head || !head->next) {
return head;
}
ListNode *dummy = new ListNode(0);
dummy->next = head;
ListNode *prev = dummy;
while(prev->next && prev->next->next) {
ListNode *first = prev->next;
ListNode *second = prev->next->next;
prev->next = second;
first->next = second->next;
second->next = first;
prev = first;
}
return dummy->next;
}
};
ListNode* createList() {
ListNode *head = NULL;
ListNode *current = NULL;
int val;
while(cin >> val) {
ListNode *newNode = new ListNode(val);
if(head == NULL) {
head = newNode;
current = newNode;
} else {
current->next = newNode;
current = current->next;
}
if(cin.get() == '\n') {
break;
}
}
return head;
}
void printList(ListNode *head) {
ListNode *current = head;
while(current != NULL) {
cout << current->val << " ";
current = current->next;
}
}
int main() {
Solution solution;
ListNode *head = createList();
ListNode *result = solution.swapPairs(head);
printList(result);
return 0;
}25. K个一组翻转链表
给你链表的头节点 head ,每 k 个节点一组进行翻转,请你返回修改后的链表。
k 是一个正整数,它的值小于或等于链表的长度。如果节点总数不是 k 的整数倍,那么请将最后剩余的节点保持原有顺序。
你不能只是单纯的改变节点内部的值,而是需要实际进行节点交换。
示例 1:

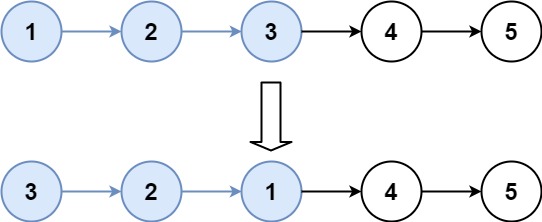
输入:head = [1,2,3,4,5], k = 2 输出:[2,1,4,3,5]
示例 2:
输入:head = [1,2,3,4,5], k = 3 输出:[3,2,1,4,5]
提示:
- 链表中的节点数目为
n 1 <= k <= n <= 50000 <= Node.val <= 1000
进阶:你可以设计一个只用 O(1) 额外内存空间的算法解决此问题吗?
#include<iostream>
using namespace std;
// Definition for singly-linked list
struct ListNode {
int val;
ListNode *next;
ListNode() : val(0), next(NULL) {}
ListNode(int x) : val(x), next(NULL) {}
ListNode(int x, ListNode *next) : val(x), next(next) {}
};
class Solution {
public:
ListNode* reverseKGroup(ListNode* head, int k) {
if(!head || k == 1) {
return head;
}
ListNode* dummy = new ListNode(0);
dummy->next = head;
ListNode* pre = dummy;
int count = 0;
ListNode* cur = head;
while(cur) {
count++;
ListNode* nextNode = cur->next;
if(count == k) {
pre = reverse(pre, nextNode); // 翻转
count = 0;
}
cur = nextNode;
}
return dummy->next;
}
ListNode* reverse(ListNode* pre, ListNode* end) {
if(!pre || !pre->next || !pre->next->next) {
return pre->next;
}
ListNode* head = pre->next;
ListNode* cur = head->next;
while(cur != end) {
head->next = cur->next;
cur->next = pre->next;
pre->next = cur;
cur = head->next;
}
return head;
}
};
ListNode* createList() {
ListNode *head = NULL;
ListNode *current = NULL;
int val;
while(cin >> val) {
ListNode *newNode = new ListNode(val);
if(head == NULL) {
head = newNode;
current = newNode;
} else {
current->next = newNode;
current = current->next;
}
if(cin.get() == '\n') {
break;
}
}
return head;
}
void printList(ListNode *head) {
ListNode *current = head;
while(current != NULL) {
cout << current->val << " ";
current = current->next;
}
}
int main() {
Solution solution;
ListNode *head = createList();
int k;
cin >> k;
ListNode *result = solution.reverseKGroup(head, k);
printList(result);
return 0;
}26. 删除有序数组中的重复项
给你一个 非严格递增排列 的数组 nums ,请你 原地 删除重复出现的元素,使每个元素 只出现一次 ,返回删除后数组的新长度。元素的 相对顺序 应该保持 一致 。然后返回 nums 中唯一元素的个数。
考虑 nums 的唯一元素的数量为 k ,你需要做以下事情确保你的题解可以被通过:
- 更改数组
nums,使nums的前k个元素包含唯一元素,并按照它们最初在nums中出现的顺序排列。nums的其余元素与nums的大小不重要。 - 返回
k。
判题标准:
系统会用下面的代码来测试你的题解:
int[] nums = [...]; // 输入数组
int[] expectedNums = [...]; // 长度正确的期望答案
int k = removeDuplicates(nums); // 调用
assert k == expectedNums.length;
for (int i = 0; i < k; i++) {
assert nums[i] == expectedNums[i];
}
如果所有断言都通过,那么您的题解将被 通过。
示例 1:
输入:nums = [1,1,2] 输出:2, nums = [1,2,_] 解释:函数应该返回新的长度 2,并且原数组 nums 的前两个元素被修改为 1, 2 。不需要考虑数组中超出新长度后面的元素。
示例 2:
输入:nums = [0,0,1,1,1,2,2,3,3,4] 输出:5, nums = [0,1,2,3,4] 解释:函数应该返回新的长度 5, 并且原数组 nums 的前五个元素被修改为 0, 1, 2, 3, 4。不需要考虑数组中超出新长度后面的元素。
提示:
1 <= nums.length <= 3 * 10^4-10^4 <= nums[i] <= 10^4nums已按 非严格递增 排列
#include<iostream>
#include<vector>
#include<algorithm>
using namespace std;
class Solution {
public:
int removeDuplicates(vector<int>& nums) {
int size = nums.size();
int left = 0, right = 1;
while(--size) {
if(nums[left] == nums[right]) {
right++;
} else {
nums[left + 1] = nums[right];
right++;
left++;
}
}
return left + 1;
}
};
int main() {
vector<int> nums;
int temp;
while(cin >> temp) {
nums.push_back(temp);
if(cin.get() == '\n')
break;
}
Solution solution;
cout<<solution.removeDuplicates(nums);
return 0;
}解法2:使用STL中的unique函数进行优化。unique函数可以将重复的元素移动到数组的末尾,并返回指向不重复部分的尾后迭代器。我们可以利用这个特性来简化代码。只需要调用unique函数,并返回结果与数组起始位置之间的距离,即为不重复元素的个数。
#include<iostream>
#include<vector>
#include<algorithm>
using namespace std;
class Solution {
public:
int removeDuplicates(vector<int>& nums) {
return distance(nums.begin(), unique(nums.begin(), nums.end()));
}
};
int main() {
vector<int> nums;
int temp;
while(cin >> temp) {
nums.push_back(temp);
if(cin.get() == '\n')
break;
}
Solution solution;
cout<<solution.removeDuplicates(nums);
return 0;
}解法3:在这个优化后的代码中,我们使用了两个指针left和right,其中left指针指向不重复部分的最后一个元素,right指针用于遍历数组。当遇到一个与left指针指向的元素不相同的元素时,将该元素移动到left指针的下一个位置,并将left指针后移一位。遍历完成后,left指针的位置加1即为不重复元素的个数。
#include<iostream>
#include<vector>
#include<algorithm>
using namespace std;
class Solution {
public:
int removeDuplicates(vector<int>& nums) {
int n = nums.size();
if(n < 2) {
return n;
}
int left = 0;
for(int right = 1; right < n; right++) {
if(nums[left] != nums[right]) {
left++;
nums[left] = nums[right];
}
}
return left + 1;
}
};
int main() {
vector<int> nums;
int temp;
while(cin >> temp) {
nums.push_back(temp);
if(cin.get() == '\n')
break;
}
Solution solution;
cout<<solution.removeDuplicates(nums);
return 0;
}27. 移除元素
给你一个数组 nums 和一个值 val,你需要 原地 移除所有数值等于 val 的元素,并返回移除后数组的新长度。
不要使用额外的数组空间,你必须仅使用 O(1) 额外空间并 原地 修改输入数组。
元素的顺序可以改变。你不需要考虑数组中超出新长度后面的元素。
说明:
为什么返回数值是整数,但输出的答案是数组呢?
请注意,输入数组是以「引用」方式传递的,这意味着在函数里修改输入数组对于调用者是可见的。
你可以想象内部操作如下:
// nums 是以“引用”方式传递的。也就是说,不对实参作任何拷贝
int len = removeElement(nums, val);
// 在函数里修改输入数组对于调用者是可见的。
// 根据你的函数返回的长度, 它会打印出数组中 该长度范围内 的所有元素。
for (int i = 0; i < len; i++) {
print(nums[i]);
}
示例 1:
输入:nums = [3,2,2,3], val = 3 输出:2, nums = [2,2] 解释:函数应该返回新的长度 2, 并且 nums 中的前两个元素均为 2。你不需要考虑数组中超出新长度后面的元素。例如,函数返回的新长度为 2 ,而 nums = [2,2,3,3] 或 nums = [2,2,0,0],也会被视作正确答案。
示例 2:
输入:nums = [0,1,2,2,3,0,4,2], val = 2 输出:5, nums = [0,1,3,0,4] 解释:函数应该返回新的长度 5, 并且 nums 中的前五个元素为 0, 1, 3, 0, 4。注意这五个元素可为任意顺序。你不需要考虑数组中超出新长度后面的元素。
提示:
0 <= nums.length <= 1000 <= nums[i] <= 500 <= val <= 100
#include<iostream>
#include<vector>
using namespace std;
class Solution {
public:
int removeElement(vector<int>& nums, int val) {
int j = 0;
for(int i = 0; i < nums.size(); i++) {
if(nums[i] != val) {
nums[j++] = nums[i]; // 不需要考虑数组中超出新长度后面的元素
}
}
return j;
}
};
int main() {
int val;
int temp;
vector<int> nums;
while(cin >> temp) {
nums.push_back(temp);
if(cin.get() == '\n') {
break;
}
}
cin>>val;
Solution solution;
int len = solution.removeElement(nums, val);
cout << len;
return 0;
} 28.找出字符串中第一个匹配项的下标
给你两个字符串 haystack 和 needle ,请你在 haystack 字符串中找出 needle 字符串的第一个匹配项的下标(下标从 0 开始)。如果 needle 不是 haystack 的一部分,则返回 -1 。
示例 1:
输入:haystack = "sadbutsad", needle = "sad" 输出:0 解释:"sad" 在下标 0 和 6 处匹配。 第一个匹配项的下标是 0 ,所以返回 0 。
示例 2:
输入:haystack = "leetcode", needle = "leeto" 输出:-1 解释:"leeto" 没有在 "leetcode" 中出现,所以返回 -1 。
提示:
1 <= haystack.length, needle.length <= 10^4haystack和needle仅由小写英文字符组成
#include<iostream>
#include<vector>
using namespace std;
class Solution {
public:
int strStr(string haystack, string needle) {
return haystack.find(needle);
}
};
int main() {
string haystack, needle;
cin >> haystack >> needle;
Solution solution;
cout<<solution.strStr(haystack, needle);
return 0;
}注:在C++中,string类的find()函数用于在一个字符串中查找子字符串,并返回第一次出现的位置。find()函数有多种重载形式,常用的形式包括:
size_t find(const string& str, size_t pos = 0) const: 在当前字符串中从指定位置pos开始查找子字符串str,返回第一次出现的位置。如果未找到则返回string::npos。size_t find(const char* s, size_t pos = 0) const: 在当前字符串中从指定位置pos开始查找C风格字符串s,返回第一次出现的位置。如果未找到则返回string::npos。size_t find(char c, size_t pos = 0) const: 在当前字符串中从指定位置pos开始查找字符c,返回第一次出现的位置。如果未找到则返回string::npos。
示例用法:
#include <iostream>
#include <string>
using namespace std;
int main() {
string str = "Hello, World!";
// 查找子字符串
size_t found = str.find("World");
if (found != string::npos) {
cout << "Found at position: " << found << endl;
} else {
cout << "Not found" << endl;
}
// 查找字符
size_t foundChar = str.find(',');
if (foundChar != string::npos) {
cout << "Found character at position: " << foundChar << endl;
} else {
cout << "Character not found" << endl;
}
return 0;
}
29. 两数相除
给你两个整数,被除数 dividend 和除数 divisor。将两数相除,要求 不使用 乘法、除法和取余运算。
整数除法应该向零截断,也就是截去(truncate)其小数部分。例如,8.345 将被截断为 8 ,-2.7335 将被截断至 -2 。
返回被除数 dividend 除以除数 divisor 得到的 商 。
注意:假设我们的环境只能存储 32 位 有符号整数,其数值范围是 [−231, 231 − 1] 。本题中,如果商 严格大于 231 − 1 ,则返回 231 − 1 ;如果商 严格小于 -231 ,则返回 -231 。
示例 1:
输入: dividend = 10, divisor = 3 输出: 3 解释: 10/3 = 3.33333.. ,向零截断后得到 3 。
示例 2:
输入: dividend = 7, divisor = -3 输出: -2 解释: 7/-3 = -2.33333.. ,向零截断后得到 -2 。
提示:
-2^31 <= dividend, divisor <= 2^31 - 1divisor != 0
#include<iostream>
#include<climits>
using namespace std;
class Solution {
public:
int divide(int dividend, int divisor) {
if(dividend == INT_MIN && divisor == -1) {
return INT_MAX;
}
long dvd = labs(dividend);
long dvs = labs(divisor);
int sign = (dividend > 0) ^ (divisor > 0) ? -1 : 1;
long res = 0;
while(dvd >= dvs) {
long temp = dvs, m = 1;
while(temp<<1 <= dvd) {
temp <<= 1;
m <<= 1; // 左移一位
}
dvd = -= temp;
res += m;
}
return sign * res;
}
};
int main() {
int dividend, divisor;
cin >> dividend >> divisor;
Solution solution;
cout << solution.divide(dividend, divisor);
return 0;
}30. 串联所有单词的子串
给定一个字符串 s 和一个字符串数组 words。 words 中所有字符串 长度相同。
s 中的 串联子串 是指一个包含 words 中所有字符串以任意顺序排列连接起来的子串。
- 例如,如果
words = ["ab","cd","ef"], 那么"abcdef","abefcd","cdabef","cdefab","efabcd", 和"efcdab"都是串联子串。"acdbef"不是串联子串,因为他不是任何words排列的连接。
返回所有串联子串在 s 中的开始索引。你可以以 任意顺序 返回答案。
示例 1:
输入:s = "barfoothefoobarman", words = ["foo","bar"] 输出:[0,9] 解释:因为 words.length == 2 同时 words[i].length == 3,连接的子字符串的长度必须为 6。 子串 "barfoo" 开始位置是 0。它是 words 中以 ["bar","foo"] 顺序排列的连接。 子串 "foobar" 开始位置是 9。它是 words 中以 ["foo","bar"] 顺序排列的连接。 输出顺序无关紧要。返回 [9,0] 也是可以的。
示例 2:
输入:s = "wordgoodgoodgoodbestword", words = ["word","good","best","word"] 输出:[] 解释:因为 words.length == 4 并且 words[i].length == 4,所以串联子串的长度必须为 16。 s 中没有子串长度为 16 并且等于 words 的任何顺序排列的连接。 所以我们返回一个空数组。
示例 3:
输入:s = "barfoofoobarthefoobarman", words = ["bar","foo","the"] 输出:[6,9,12] 解释:因为 words.length == 3 并且 words[i].length == 3,所以串联子串的长度必须为 9。 子串 "foobarthe" 开始位置是 6。它是 words 中以 ["foo","bar","the"] 顺序排列的连接。 子串 "barthefoo" 开始位置是 9。它是 words 中以 ["bar","the","foo"] 顺序排列的连接。 子串 "thefoobar" 开始位置是 12。它是 words 中以 ["the","foo","bar"] 顺序排列的连接。
提示:
1 <= s.length <= 1041 <= words.length <= 50001 <= words[i].length <= 30words[i]和s由小写英文字母组成
解法一:超时版
#include<iostream>
#include<vector>
#include<string>
#include<unordered_map>
using namespace std;
class Solution {
public:
vector<int> findSubstring(string s, vector<string>& words) {
vector<int> result;
if(s.empty() || words.empty()) {
return result;
}
int wordLen = words[0].length();
int wordCount = words.size();
int totalLen = wordLen * wordCount;
unordered_map<string, int> wordFreq;
for(const string& word : words) {
wordFreq[word]++;
}
for(int i = 0; i <= (int)s.length() - totalLen; ++i) {
unordered_map<string, int> seen;
int j = 0;
for(; j < wordCount; ++j) {
string word = s.substr(i + j * wordLen, wordLen);
if(wordFreq.find(word) == wordFreq.end()) {
break;
}
seen[word]++;
if(seen[word] > wordFreq[word]) {
break;
}
}
if(j == wordCount) {
result.push_back(i);
}
}
return result;
}
};
int main() {
string s;
vector<string> words;
string tmp;
cin >> s;
while(cin >> tmp) {
words.push_back(tmp);
if(cin.get() == '\n')
break;
}
Solution solution;
vector<int> result = solution.findSubstring(s, words);
for(auto num : result) {
cout << num << " ";
}
return 0;
}解法二:在每个可能的位置只遍历一次,使用滑动窗口来优化时间复杂度。
#include<iostream>
#include<vector>
#include<string>
#include<unordered_map>
using namespace std;
class Solution {
public:
vector<int> findSubstring(string s, vector<string>& words) {
vector<int> result;
if(s.empty() || words.empty()) {
return result;
}
int wordLen = words[0].length();
int wordCount = words.size();
int totalLen = wordLen * wordCount;
unordered_map<string, int> wordFreq;
for(const string& word : words) {
wordFreq[word]++;
}
for(int i = 0; i < wordLen; i++) {
int left = i, count = 0;
unordered_map<string, int> seen;
for(int j = i; j <= (int)s.length() - totalLen; j += wordLen) {
string word = s.substr(j, wordLen);
if(wordFreq.find(word) != wordFreq.end()) {
seen[word]++;
count++;
while(seen[word] > wordFreq[word]) {
string temp = s.substr(left, wordLen);
seen[temp]--;
count--;
left += wordLen;
}
if(count == wordCount) {
result.push_back(left);
string temp = s.substr(left, wordLen);
seen[temp]--;
count--;
left += wordLen;
}
} else {
seen.clear();
count = 0;
left = j + wordLen;
}
}
}
return result;
}
};
int main() {
string s;
vector<string> words;
string tmp;
cin >> s;
while(cin >> tmp) {
words.push_back(tmp);
if(cin.get() == '\n')
break;
}
Solution solution;
vector<int> result = solution.findSubstring(s, words);
for(auto num : result) {
cout << num << " ";
}
return 0;
}