Java优先级队列（堆）

🐵本篇文章将对优先级队列（堆）的相关知识进行讲解

一、优先级队列

队列是一种“先入先出”的数据结构，但有时操作的数据带有优先级，需要优先处理，这时普通的队列就不能满足需求。比如：在排队取票时，往往会有老人优先、退伍军人优先等。这就可以理解为优先级队列的一种体现

优先级队列的底层是由一种叫堆的数据结构实现的

二、堆

2.1 什么是堆

将元素按照完全二叉树的顺序存储方式存储到一维数组中，堆分为大根堆和小根堆

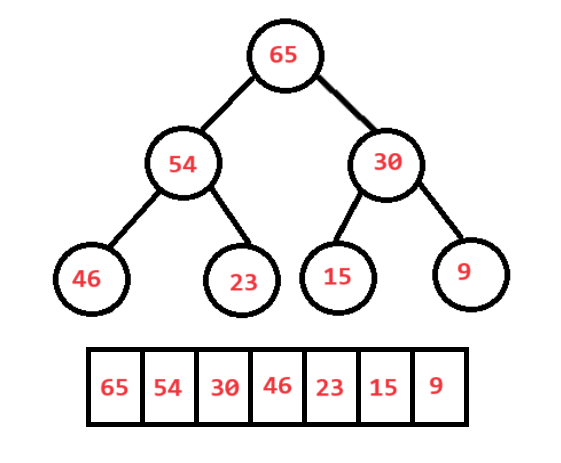
如上图，如果满足每一个父亲节点的值都大于其左右孩子结点的值，则称为大根堆

如上图，如果满足每一个父亲节点的值都小于其左右孩子结点的值，则称为小根堆

2.2 堆的创建

堆的向下调整

public class Heap {
    public int[] elem;
    public int usedSize; //记录数组中元素的个数

    public Heap() {
        this.elem = new int[10]; //构造方法：数组的初始容量为10
    }

    public void initElem(int[] array) { //初始化elem数组
        for (int i = 0; i < elem.length; i++) {
            elem[i] = array[i];
            usedSize++;
        }
    }
}

以大根堆为例，如果一棵完全二叉树是大根堆，则其每一课子树也都是大根堆，定义一个parent表示最后一棵子树的根结点的下标，再定义一个child表示parent结点的左孩子的下标

每调整完一个子树后就让parent--，去调整下一棵子树

那么该如何用代码表示parent和child的下标：首先是parent，usedSize表示数组中元素的个数，usedSize - 1就是最后一个结点的下标，根据二叉树的性质，让其减1再除2就是要得到的最后一个子树的根结点下标，表示为（usedSize -1 - 1）/ 2，child同样也是根据二叉树的性质，child = 2 * parent + 1

得到parent和child后，开始对第一个子树进行调整：首先要让child表示为左右孩子较大值结点的下标

    public void creatBigHeap() { //创建大根堆
        for (int parent = (usedSize - 1 - 1) / 2; parent >= 0 ; parent--) {
            siftDown(parent, usedSize);
        }
    }
    public void siftDown(int parent, int end) { //向下调整
        int child = 2 * parent + 1;
        if (child + 1 < usedSize && elem[child] < elem[child + 1]) {
            child++;
        }
        ...
    }

接下来就是比较elem[parent]和elem[child]，如果孩子结点大于其父亲结点则进行交换，交换完后，让parent = child,child = 2 * parent + 1，继续向下调整

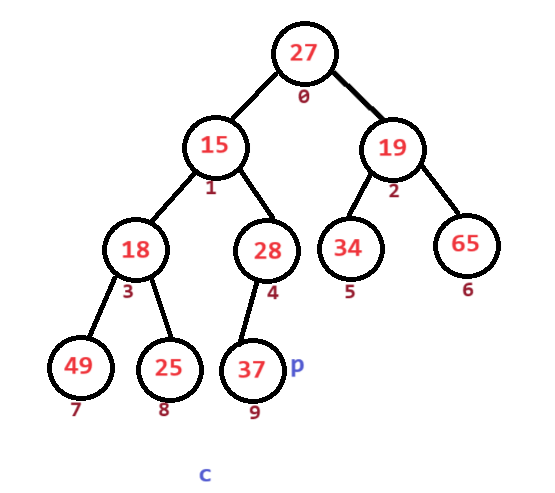
如上图，此时说明这个子树已经调整完毕，当在调整{19， 34}这个树时当parent = 5、child = 11时，这个树就调整完了，所以可以用代码写一个循环，循环条件为child < usedSize

    public void siftDown(int parent, int end) {
        int child = 2 * parent + 1;
        while(child < end) {
            if (child + 1 < usedSize && elem[child] < elem[child + 1]) {
                child++;
            }
            if (elem[child] > elem[parent]) {
                swap(child, parent);
                parent = child;
                child = 2 * parent + 1;
            } else {
                break;
            }

        }
    }
    private void swap(int child, int parent) {
        int tmp = elem[child];
        elem[child] = elem[parent];
        elem[parent] = tmp;
    }

那么整个大根堆就创建完了，完整代码如下：

public class Heap {
    public int[] elem;
    public int usedSize; //记录数组中元素的个数

    public Heap() {
        this.elem = new int[10]; //构造方法：数组的初始容量为10
    }
    public void initElem(int[] array) { //初始化elem数组
        for (int i = 0; i < elem.length; i++) {
            elem[i] = array[i];
            usedSize++;
        }
    }

    public void creatBigHeap() { //时间复杂度为O(n)
        for (int parent = (usedSize - 1 - 1) / 2; parent >= 0 ; parent--) {
            siftDown(parent, usedSize);
        }
    }
    public void siftDown(int parent, int end) {
        int child = 2 * parent + 1;
        while(child < end) {
            if (child + 1 < usedSize && elem[child] < elem[child + 1]) {
                child++;
            }
            if (elem[child] > elem[parent]) {
                swap(child, parent);
                parent = child;
                child = 2 * parent + 1;
            } else {
                break;
            }

        }
    }
    private void swap(int child, int parent) {
        int tmp = elem[child];
        elem[child] = elem[parent];
        elem[parent] = tmp;
    }
}

堆的向上调整和向下调整类似

    public void siftUp(int child) {
        int parent = (child - 1) / 2;
        while(parent > 0) {
            if (child + 1 < usedSize && elem[child] < elem[child + 1]) {
                child++;
            }
            if (elem[child] > elem[parent]) {
                swap(child, parent);
                child = parent;
                parent = (child - 1) / 2;
            } else {
                break;
            }
        }
    }

2.3 堆的插入与删除

堆的插入可以将元素放到数组的末尾，然后可以通过向上调整将其调整为大根堆或小根堆

    public void offer(int key) {
        if (isFull()) { //判断是否需要扩容
            elem = Arrays.copyOf(elem, 2 * usedSize);
        }
        
        elem[usedSize] = key;
        usedSize++;
        
        siftUp(usedSize - 1);
    }

堆的删除，由于堆顶的元素，所以可以将堆顶的元素和最后一个元素交换，再让usedSize--后将其向下调整为大根堆或小根堆即可

    public int poll() {
        int tmp = elem[0];
        swap(0, usedSize - 1);
        usedSize--;
        siftDown(0, usedSize);
        return tmp;
    }

2.4 堆排序

堆排序就是按照堆的思想排序，假如要将一组数据按照从小到大排序，则先将这组数据创建为大根堆，不建小根堆是因为其不能保证左右孩子也是有序的。以下图为例：

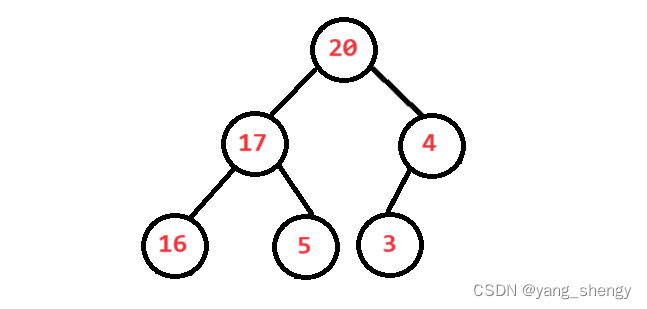
由于是大根堆，所以堆顶元素是最大的，将堆顶元素和最后一个元素交换，交换后再调整为大根堆，此时再将堆顶元素与倒数第二个元素交换，交换后再调整为大根堆，如此循环

    public void heapSort() {
        int end = usedSize - 1; //此时为最后一个元素的下标
        while(end > 0) {
            swap(0, end);
            siftDown(0, end - 1);//由于交换了0下标，所以只需要从0下标开始向下调整
                                //交换完后end位置不再属于大根堆，所以在end -1处结束调整
            end--;
        }
    }

三、PriorityQueue接口介绍

该接口的底层是一个小根堆，先来介绍在该接口中的几个字段

3.1 构造方法

首先是上述三个基础的构造方法，可以观察到这三个构造方法都调用了另外一个构造方法：

调用不带参数的构造方法，此时数组的大小为初始容量：11

调用第一个带参数的构造方法，此时数组的大小为initalCapacity

第三个构造方法，其参数是一个比较器，接下来简单复习一下比较器（Comparator接口）

class Person {
    public int age;
    public String name;
    public Person(int age, String name) {
        this.age = age;
        this.name = name;
    }
}

class Imp implements Comparator<Person> {
    public int compare(Person p1, Person p2) {
        return p1.name.compareTo(p2.name); //调用了String类的compareTo方法
            //前者>后者，返回一个大于0的数
            //前者<后者，返回一个小于0的数
            //前者=后者，返回0
    }
}

class Main {
    public static void main(String[] args) {
        Person p1 = new Person("Sans", 20);
        Person p2 = new Person("Frisk", 8);
        Imp imp = new Imp();
        System.out.println(imp.compare(p1, p2));
    }
}

调用第二个带参数的构造方法