20.1 Particles of a gas
Brownian motion
Fast modules
速率的数值大概了解下:
average speed of the molecules:400m/s
speed of sound:approximately 330m/s at STP(standard temperature and pressure)
Standard Temperature and Pressure (STP) 是物理学与化学中的一个理想状态,也被简称为“标况”或“STP”。它表示的是温度为0℃(或273.15K)和压强为101.325千帕(或1个大气压,约为760毫米汞柱)时的状况。这样的定义接近海平面上水的冰点。STP是为了研究方便而制定的,用于描述物质在特定条件下的特征。由于地表各处的温度、压强皆不同,即使是同一地点的温度压强也随测量时间不同而相异,因此STP为研究者提供了一个统一的参考条件。
20.2 Explaining pressure
Two factors affect the force, and hence the pressure, that the gas exerts on the box:
●the number of molecules that hit each side of the box in one second
●the force with which a molecule collides with the wall.
Questions
这两个question并不容易解释,不要轻视。
1
1
1.State and explain, in terms of the kinetic model (the movement of molecules), what happens to the
pressure inside a tyre when more molecules at the same temperature are pumped into the tyre.
答:
Pressure increases because more molecules are hitting a unit area of the tyre per second. The molecules have the same speed and each collision causes the same impulse or change in momentum. Since more molecules hit per second, the rate of change of momentum, i.e. the force, increases.
2.
2.
2.Explain, using the kinetic model, why a can containing air may explode if the temperature rises.
答:
At higher temperatures, the molecules have higher internal energy and move faster. The number of collisions per second increases and the change in momentum of each collision also increases. For both these reasons, the rate of change of momentum of the molecules increases. The force on the wall is equal and opposite to the rate of change of momentum of the molecules. As the force increases, the pressure inside the can may cause it to explode.
20.3 Measuring gases
Avogadro constant(阿伏伽德罗常数)
N
A
=
6.02
∗
1
0
23
/
m
o
l
N_A=6.02*10^{23}/mol
NA=6.02∗1023/mol
1
u
=
1.66
×
1
0
−
27
1u=1.66\times10^{-27}
1u=1.66×10−27
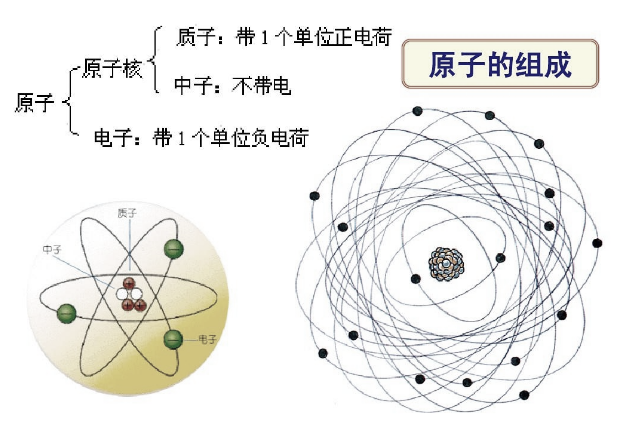
一个原子只有一个原子核,不同元素 的原子核内有不同的质子数,例如:
氧原子核有16个质子,
碳原子核有12个质子。
20.4-20.6知识点汇总
| 公式名称 | 对象 | 公式 | 使用条件 |
|---|---|---|---|
| Boyle’s Law | 理想气体 | P 1 V 1 = P 2 V 2 P_1V_1=P_2V_2 P1V1=P2V2 | 恒温 |
| Charle’s Law | 理想气体 | V 1 T 1 = V 2 T 2 \frac{V_1}{T_1}=\frac{V_2}{T_2} T1V1=T2V2 | 恒压 |
| Gay-Lussac’s Law | 理想气体 | P 1 T 1 = P 2 T 2 \frac{P_1}{T_1}=\frac{P_2}{T_2} T1P1=T2P2 | 恒体积 |
| Ideal Gas Law | 理想气体 |
P
V
=
n
R
T
PV = nRT
PV=nRT P V = N k T PV=NkT PV=NkT |
其中:
R
=
k
⋅
N
A
R=k\cdot N_A
R=k⋅NA
n
=
N
/
N
A
n=N/N_A
n=N/NA
n
R
=
N
k
nR=Nk
nR=Nk
| 符号 | 名称 | 备注 |
|---|---|---|
| R | universal molar gas constant | 8.31 J / ( m o l ⋅ K ) 8.31J/(mol·K) 8.31J/(mol⋅K) |
| k | Boltzmann constant | 1.3806 × 1 0 − 23 J / K 1.3806×10^{-23}J/K 1.3806×10−23J/K |
| n | amount(number of moles) of an ideal gas | 摩尔量 |
| N | number of molecules | 分子数量 |

20.7 Modelling gases:the kinetic model
more frequent or harder collisions give rise to greater pressure. Its temperature indicates the average kinetic energy of its particles;
the faster they move, the greater their average kinetic energy and the higher the temperature.(这句话其实是在说等温慢速压缩)
下面是模型假设

题目中会出现的公式
| physical quantity | formula |
|---|---|
| change in momentum | 2 m c 2mc 2mc |
| force | 2 m c 2 l c = m c 2 l \frac{2mc}{\frac{2l}{c}}=\frac{mc^2}{l} c2l2mc=lmc2 |
| pressure for one molecule | m c 2 l l 2 = m c 2 l 3 \frac{\frac{mc^2}{l}}{l^2}=\frac{mc^2}{l^3} l2lmc2=l3mc2 |
| pressure for all molecules |
1
3
N
⋅
m
<
c
2
>
l
3
\frac{1}{3}\frac{N\cdot m<c^2>}{l^3}
31l3N⋅m<c2> = 1 3 N ⋅ m < c 2 > V \frac{1}{3}\frac{N\cdot m<c^2>}{V} 31VN⋅m<c2> = 1 3 ρ < c 2 > \frac{1}{3}\rho<c^2> 31ρ<c2> |
20.8 Temperature and molecular kinetic energy
1
3
N
m
<
c
2
>
=
n
R
T
=
N
k
T
\frac{1}{3}Nm<c^2>=nRT=NkT
31Nm<c2>=nRT=NkT
k
i
n
e
t
i
c
e
n
e
r
g
y
=
1
2
m
<
c
2
>
=
3
R
T
2
N
A
=
3
2
k
T
kinetic\ energy=\frac{1}{2}m<c^2>=\frac{3RT}{2N_A}=\frac{3}{2}kT
kinetic energy=21m<c2>=2NA3RT=23kT
The root-mean-square speed
c r . m . s = < c 2 > = 1 n ∑ i = 1 n v i 2 c_{r.m.s}=\sqrt{<c^2>}=\sqrt{\frac{1}{n}\sum_{i=1}^nv_i^2} cr.m.s=<c2>=n1∑i=1nvi2
Mass kinetic energy and temperature
In a sample of air, the mean k.e. of
the nitrogen molecules is the same as that of the oxygen molecules and that of the carbon dioxide
molecules. This comes about because they are all repeatedly colliding with one another, sharing their
energy. Carbon dioxide molecules have greater mass than oxygen molecules; since their mean
translational k.e. is the same, it follows that the carbon dioxide molecules move more slowly than the
oxygen molecules.
Exam-style Questions
理想气体与非理想气体
| ideal gas | non-ideal gas | |
|---|---|---|
| 内能 | E t o t a l = E k E_{total}=E_k Etotal=Ek | E t o t a l = E k + E p E_{total}=E_k+E_p Etotal=Ek+Ep |
| 适用公式 | p V = N k T = n R T pV=NkT=nRT pV=NkT=nRT | |
| The First Law of Thermodynamics | E t o t a l = Δ u = q + W E_{total}=\Delta u=q+W Etotal=Δu=q+W | E t o t a l = Δ u = q + W E_{total}=\Delta u=q+W Etotal=Δu=q+W |
两种压缩分析(题目中会用到)
①Chapter19中提到isothermal change
②快速压缩的相关内容在20.7中、本章课后习题 的5b中都有有涉及
| isothermal change (等温慢速压缩) | adiabatic (绝热快速压缩) | |
|---|---|---|
| p p p | ↑ | ↑ |
| V V V | ↓ | ↓ |
| T T T | 不变 | ↑ |
| E k E_k Ek | 不变(散热实现) | ↑(来不及散热) |
| E p ( i d e a l g a s ) E_p(ideal\ gas) Ep(ideal gas) | 0 0 0 | 0 0 0 |
| E p ( n o n − i d e a l g a s ) E_p(non-ideal\ gas) Ep(non−ideal gas) | 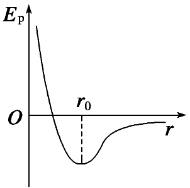 |  |
| E t o t a l E_{total} Etotal | 不变 | ↑ |
| q q q | < 0 \lt 0 <0(散热实现) | = 0 = 0 =0(来不及散热) |
| W W W | > 0 \gt 0 >0 | > 0 >0 >0 |
| Δ u = q + W ( i d e a l g a s ) \Delta u=q+W(ideal\ gas) Δu=q+W(ideal gas) | 0 0 0 | > 0 \gt 0 >0 |
| Δ u = q + W ( n o n − i d e a l g a s ) \Delta u=q+W(non-ideal\ gas) Δu=q+W(non−ideal gas) | 不一定是 0 不一定是0 不一定是0 | > 0 \gt 0 >0 |