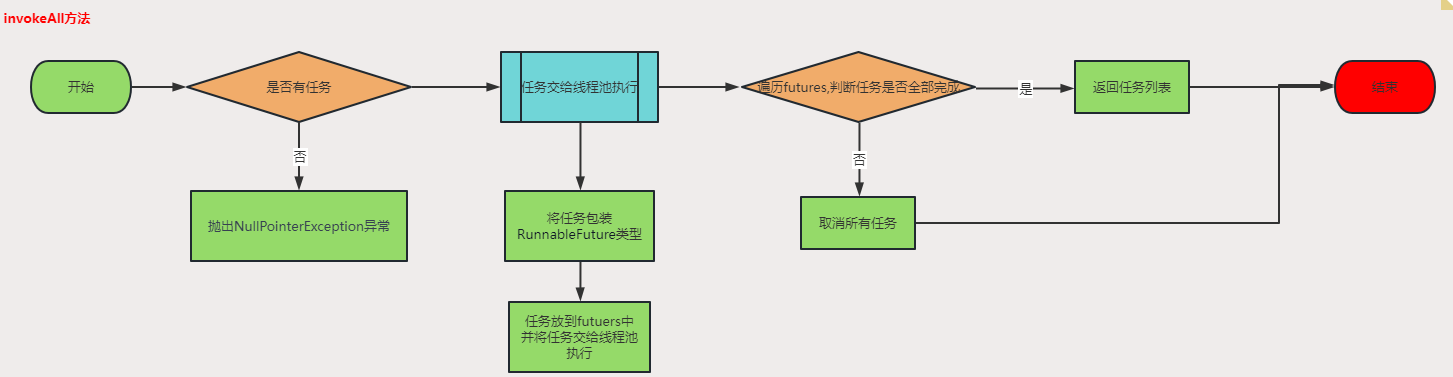
1.全排列
给定一个不含重复数字的数组 nums ,返回其 所有可能的全排列 。你可以 按任意顺序 返回答案。
示例 1:
输入:nums = [1,2,3]
输出:[[1,2,3],[1,3,2],[2,1,3],[2,3,1],[3,1,2],[3,2,1]]
示例 2:
输入:nums = [0,1]
输出:[[0,1],[1,0]]
示例 3:
输入:nums = [1]
输出:[[1]]
我的解法(回溯)
class Solution {
public List<List<Integer>> permute(int[] nums) {
Arrays.sort(nums);
List<List<Integer>> res = new ArrayList<>();
// 记录已经被选过的元素
boolean[] used = new boolean[nums.length];
backtracing(nums, used, res, new ArrayDeque<Integer>());
return res;
}
public void backtracing(int[] nums, boolean[] used, List<List<Integer>> res, Deque<Integer> path){
if(path.size() == nums.length){
res.add(new ArrayList<>(path));
return;
}
for(int i = 0; i < nums.length; ++i){
if(used[i] == true) continue;
used[i] = true;
path.add(nums[i]);
backtracing(nums, used, res, path);
path.removeLast();
used[i] = false;
}
}
}
2.子集
给你一个整数数组 nums ,数组中的元素 互不相同 。返回该数组所有可能的子集(幂集)。
解集 不能 包含重复的子集。你可以按 任意顺序 返回解集。
示例 1:
输入:nums = [1,2,3]
输出:[[],[1],[2],[1,2],[3],[1,3],[2,3],[1,2,3]]
示例 2:
输入:nums = [0]
输出:[[],[0]]
我的解法(回溯)
class Solution {
public List<List<Integer>> subsets(int[] nums) {
List<List<Integer>> res = new ArrayList<>();
backtracking(nums, 0, res, new ArrayDeque<Integer>());
return res;
}
public void backtracking(int[] nums, int startIndex, List<List<Integer>> res, Deque<Integer> path){
res.add(new ArrayList<>(path));
for(int i = startIndex; i < nums.length; ++i){
path.add(nums[i]);
backtracking(nums, i + 1, res, path);
path.removeLast();
}
}
}
3.根据身高重建队列
假设有打乱顺序的一群人站成一个队列,数组 people 表示队列中一些人的属性(不一定按顺序)。每个 people[i] = [hi, ki] 表示第 i 个人的身高为 hi ,前面 正好 有 ki 个身高大于或等于 hi 的人。
请你重新构造并返回输入数组 people 所表示的队列。返回的队列应该格式化为数组 queue ,其中 queue[j] = [hj, kj] 是队列中第 j 个人的属性(queue[0] 是排在队列前面的人)。
示例 1:
输入:people = [[7,0],[4,4],[7,1],[5,0],[6,1],[5,2]]
输出:[[5,0],[7,0],[5,2],[6,1],[4,4],[7,1]]
解释:
编号为 0 的人身高为 5 ,没有身高更高或者相同的人排在他前面。
编号为 1 的人身高为 7 ,没有身高更高或者相同的人排在他前面。
编号为 2 的人身高为 5 ,有 2 个身高更高或者相同的人排在他前面,即编号为 0 和 1 的人。
编号为 3 的人身高为 6 ,有 1 个身高更高或者相同的人排在他前面,即编号为 1 的人。
编号为 4 的人身高为 4 ,有 4 个身高更高或者相同的人排在他前面,即编号为 0、1、2、3 的人。
编号为 5 的人身高为 7 ,有 1 个身高更高或者相同的人排在他前面,即编号为 1 的人。
因此 [[5,0],[7,0],[5,2],[6,1],[4,4],[7,1]] 是重新构造后的队列。
示例 2:
输入:people = [[6,0],[5,0],[4,0],[3,2],[2,2],[1,4]]
输出:[[4,0],[5,0],[2,2],[3,2],[1,4],[6,0]]
我的解法(贪心)
class Solution {
public int[][] reconstructQueue(int[][] people) {
Arrays.sort(people, (a, b) -> {
if(a[0] == b[0]) return a[1] - b[1];
return b[0] - a[0];
});
LinkedList<int[]> res = new LinkedList<>();
for(int[] p : people){
res.add(p[1], p);
}
return res.toArray(new int[people.length][]);
}
}
4.盛最多水的容器
给定一个长度为 n 的整数数组 height 。有 n 条垂线,第 i 条线的两个端点是 (i, 0) 和 (i, height[i]) 。
找出其中的两条线,使得它们与 x 轴共同构成的容器可以容纳最多的水。
返回容器可以储存的最大水量。
说明:你不能倾斜容器。
示例 1:

输入:[1,8,6,2,5,4,8,3,7]
输出:49
解释:图中垂直线代表输入数组 [1,8,6,2,5,4,8,3,7]。在此情况下,容器能够容纳水(表示为蓝色部分)的最大值为 49。
示例 2:
输入:height = [1,1]
输出:1
我的解法(暴力破解)
class Solution {
public int maxArea(int[] height) {
int max = 0;
for(int i = 0; i < height.length; ++i){
for(int j = height.length - 1; j >= 0; --j){
max = Math.max(max, (j - i) * Math.min(height[i], height[j]));
}
}
return max;
}
}
一做算法题脑子就跟浆糊一样,两眼一瞪,啥也不会,暴力破解还超时,没有天理啊。
官方解法(双指针)
class Solution {
public int maxArea(int[] height) {
int res = 0, i = 0, j = height.length - 1;
while(i < j){
res = height[i] < height[j] ?
Math.max(res, (j - i) * height[i++]):
Math.max(res, (j - i) * height[j--]);
}
return res;
}
}
没有思路时,一定要仔细分析,如果实在想不出来就动笔画画图。