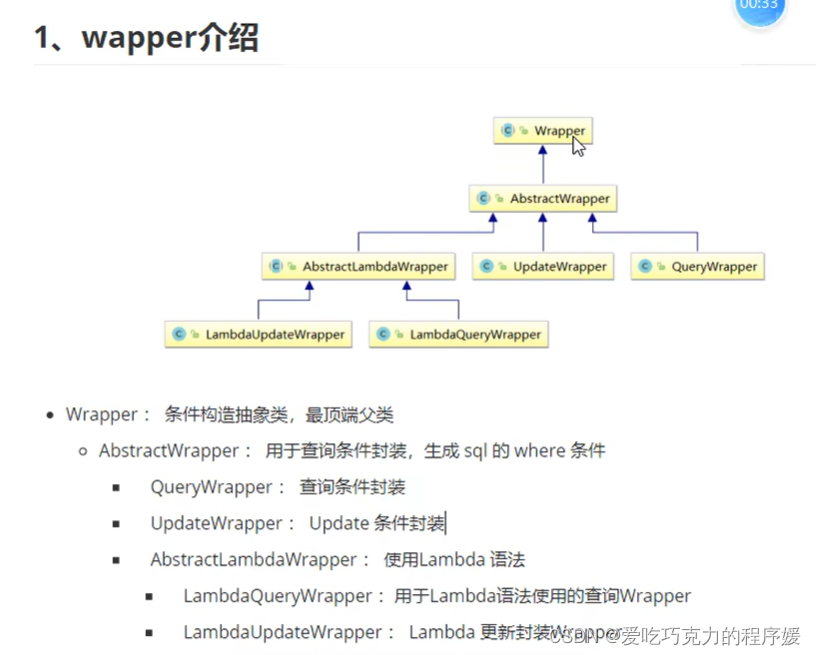
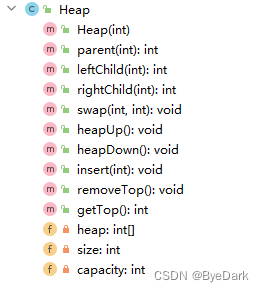
堆的函数主要有，插入，删除，查看堆顶元素。
建堆主要依靠插入函数。
我们需要定义一个数组，int类型长度和int类型容量。
在操作过程中我们需要用到查看父亲节点函数，查看左孩子节点函数，查看右孩子节点函数和交换元素位置函数。
除了上面之外，插入和删除两个操作需要涉及到堆的元素上浮函数和元素下沉函数。
所有信息如下所示：
代码：

package org.example.heap;

import java.util.*;

public class Heap {
    private int[] heap;
    private int size;
    private int capacity;

    public Heap(int capacity){
        this.capacity = capacity;
        this.size = 0;
        this.heap = new int[capacity];
    }

    //0->1->2
    public int parent(int index){
        return (index - 1) / 2;
    }

    public int leftChild(int index){
        return index * 2 + 1;
    }

    public int rightChild(int index){
        return index * 2 + 2;
    }

    public void swap(int i,int j){
        int temp = heap[i];
        heap[i] = heap[j];
        heap[j] = temp;
    }

    /**
     * 上移
     */
    public void heapUp(){
        int index = size -1 ;
        //一直可以移动到堆顶
        while(index>0&&heap[index]>heap[parent(index)]){
            swap(index,parent(index));
            index = parent(index);
        }
    }

    /**
     * 下沉
     */
    public void heapDown(){
        int index = 0;
        //有一个孩子说明就不是根节点，没必要左右节点都进行判断，因为左节点没有右节点一定没有，左节点的优先级要高于右节点
        //这样子代码冗余度过高，我们考虑通过预先确定较大值的下标来简化代码
//        while(leftChild(index)<size){
//            int left = leftChild(index);
//            int right = rightChild(index);
//            if(right<size){
//                int maxNum = Math.max(heap[left],heap[right]);
//                if(heap[index]>maxNum)break;
//                //把大的拿个换上来
//                if(heap[left]<heap[right]){
//                    swap(index,right);
//                    index = rightChild(index);
//                }else {
//                    swap(index,left);
//                    index = leftChild(index);
//                }
//            }else{
//                if(heap[index]>heap[left])break;
//                swap(index,left);
//                index = leftChild(index);
//            }
//        }
        while(leftChild(index)<size){
            int left = leftChild(index);
            int right = rightChild(index);
            int min = left;
            if(right<size&&heap[right]>heap[left])
                min = right;
            if(heap[min]<heap[index])break;
            else{
                swap(index,min);
                index = min;
            }
        }
    }

    /**
     * 插入元素
     */
    public void insert(int value) {
        //给堆设置一个阈值，如果堆内的元素达到了总容量的0.6自动触发扩容
        size++;
        if(size>=capacity*0.6){
            //进行堆扩容
            int[] temp = new int[capacity*2];
            if (capacity >= 0) System.arraycopy(heap, 0, temp, 0, capacity);
            heap = temp;
            capacity*=2;
        }
        heap[size-1] = value;
        heapUp();
    }

    /**
     * 移除堆顶元素
     */
    public void removeTop(){
        if(size==0)
            throw new IllegalStateException("堆是空的");
        int min = heap[0];
        heap[0] = heap[size-1];
        size--;
        heapDown();
    }

    /**
     * 获取堆顶元素
     */
    public int getTop(){
        if(size>0)
            return heap[0];
        else
            throw new IllegalStateException("堆中无元素");
    }
}

我们测试一下：

package org.example.heap;

public class HeapTest {
    public static void main(String[] args) {
        Heap heap = new Heap(10);
        heap.insert(2);
        heap.insert(1);
        heap.insert(3);
        heap.insert(2);
        heap.insert(5);
        //5
        System.out.println(heap.getTop());
        heap.removeTop();
        //3
        System.out.println(heap.getTop());
        heap.removeTop();
        //2
        System.out.println(heap.getTop());
        heap.removeTop();
        //2
        System.out.println(heap.getTop());
        heap.removeTop();
        //1
        System.out.println(heap.getTop());
    }
}

结果符合预期