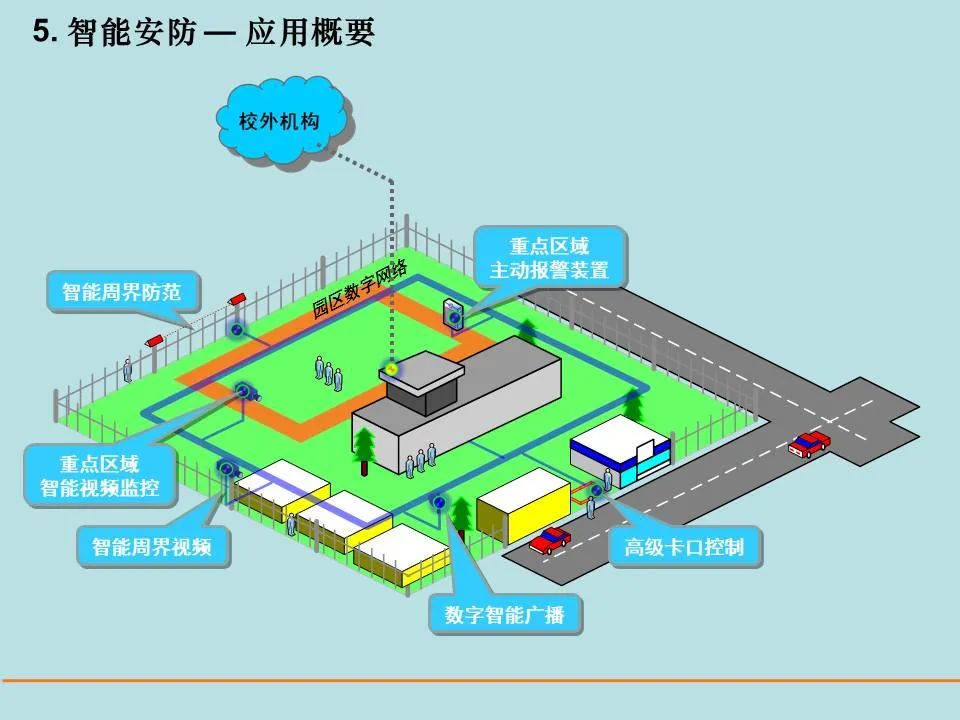
飞行路线

这一题除了堆优化和dijstra算法和链式前向星除外还多考了一个考点就是，分层图，啥叫分层图呢？简而言之就是一个三维的图，按照其题意来说有几个可以免费的点就有几层，而且这个分层的权值为0（这样就相当于免费了）， 怎么来理解这个意思呢？就是相当于这个dijstra算法它遍历的不再是一个一维图而是一个三维图，本质还是一样的，由于我们储存的边信息用的是链式前向星，所有所有的边都是按照顺序结构存放在一个一个顺序表中，所以我们不用担心空间复杂度的问题，只需要担心时间复杂度，但是由于我们用到了对堆优化。这一题就是相当于将前面的堆优化就上dijstra算法加上链式前向星重新复习一遍。

代码如下

#include<iostream>
#include<queue>
#include<string.h>
using namespace std;
const int M = 2e5;
const int N = 5e6;
int ans[N], cnt = 0, head[N], s, t, n, m, k;
bool vis[N];
//优先队列的结构体
struct node {
	int id;
	int dis;
	bool operator< (const node& x) const {
		return x.dis < dis;
	}
};
//优先队列
priority_queue<node> q;
//边的结构体
struct EDGE
{
	int next;
	int w;
	int to;
}e[N];
//键边函数
void add(int u, int v, int w)
{
	e[++cnt].w = w;
	e[cnt].to = v;
	e[cnt].next = head[u];
	head[u] = cnt;
}
//dijstra函数
void dijstra()
{
	ans[s] = 0;
	q.push(node{ s,0 });
	while (!q.empty())
	{
		node tmp = q.top();
		q.pop();
		int k = tmp.id;
		if (vis[k])
			continue;
		vis[k] = true;
		for (int i = head[k]; i != 0; i = e[i].next)
		{
			int to = e[i].to;
			if (!vis[to] && ans[to] > ans[k] + e[i].w)
			{
				ans[to] = ans[k] + e[i].w;
				q.push(node{ to,ans[to] });
			}
		}
	}
}

int main()
{
	cin >> n >> m >> k;
	cin >> s >> t;
	s++;
	t++;
	memset(ans, 0x3f, sizeof(ans));
	for (int i = 1; i <= m; i++)
	{
		int u, v, w;
		cin >> u >> v >> w;
		++u;
		++v;
		add(u, v, w);
		add(v, u, w);
		for (int j = 1; j <= k; j++) {
			add(u + (j - 1) * n, v + j * n, 0);
			add(v + (j - 1) * n, u + j * n, 0);
			add(v + j * n, u + j * n, w);
			add(u + j * n, v + j * n, w);
		}
	}
	dijstra();
	int anss = 0x7fffffff;
	for (int i = 0; i <= k; i++)
	{
		if (anss > ans[t + i * n])
		{
			anss = ans[t + i * n];
		}
	}
	cout << anss << endl;
	return 0;
}

选数

为什么要重新写一下这一题，因为我在这题错过两遍了，为了防止错三遍 ，再写一遍，结果终于是在没有外力靠住下写出来了
主要思路还是深搜：在dfs函数中需要定义三个变量，第一是就是一记录有多少个答案，第二就是就是for循环的下标，第三就是sum用于记录这个和。

代码如下（我竟然真的靠自己完全写出来的）

#include<iostream>
#include<queue>
#include<algorithm>
#include<cmath>
using namespace std;
int n,m;
int a[100000];
bool ispear(int x)
{
	if(x==1)
	return false;
	if(x==2)
	return true;
	if(x>3)
	{
		for(int i=2;i<=sqrt(x);i++)
		{
			if(x%i==0)
			return false;
		}
	}
	return true;
}
int ans=0;
void dfs(int sum,int step,int cnt)
{
	if(cnt>=m)
	{
		if(ispear(sum))
		{
			ans++;
		}
		return ;
	}
	for(int i=step+1;i<=n;i++)
	{
		dfs(sum+a[i],i,cnt+1);
	}
}
int main()
{
	cin>>n>>m;
	for(int i=1;i<=n;i++)
	cin>>a[i];
	dfs(0,0,0);
	cout<<ans<<endl;
	return 0;
}