盛水最多的容器（Python+Go）

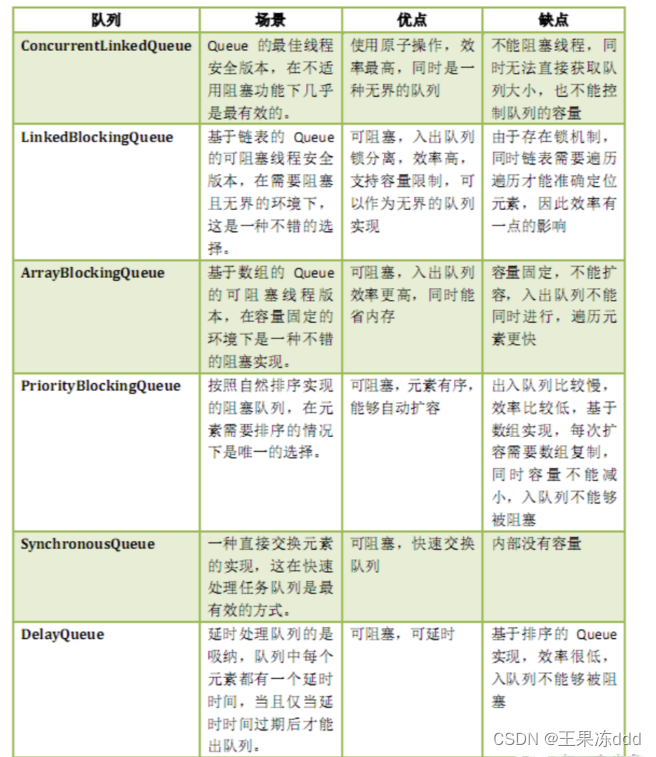
给定一个长度为 n 的整数数组 height 。有 n 条垂线，第 i 条线的两个端点是 (i, 0) 和 (i, height[i]) 。

找出其中的两条线，使得它们与 x 轴共同构成的容器可以容纳最多的水。返回容器可以储存的最大水量。(不能倾斜容器)
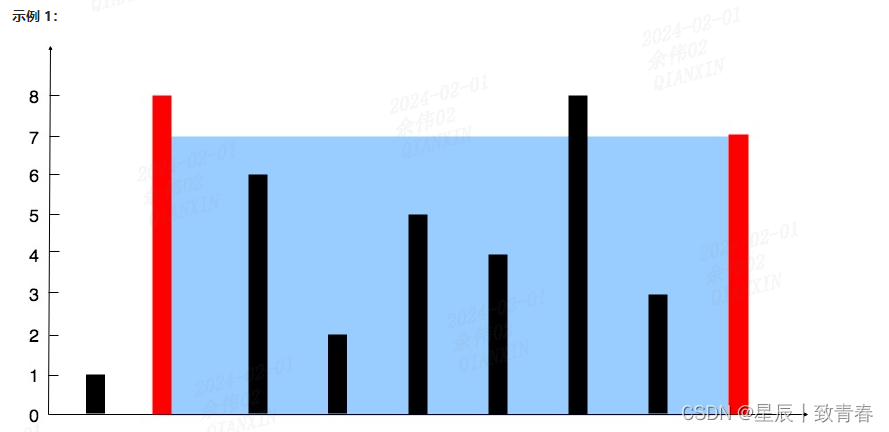
在这里插入图片描述

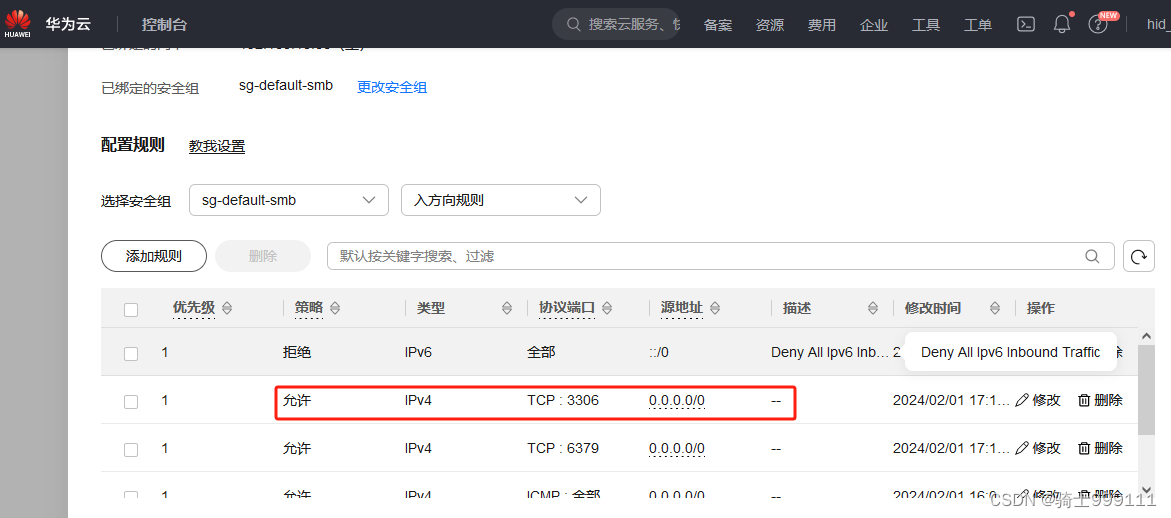
输入：[1,8,6,2,5,4,8,3,7]
输出：49
解释：图中垂直线代表输入数组 [1,8,6,2,5,4,8,3,7]。在此情况下，容器能够容纳水（表示为蓝色部分）的最大值为 49。

示例 2：

输入：height = [1,1]
输出：1

思路一：暴力求解（数据多，会超时）
对高（height）进行大到小排序，然后从大到小依次计算所有面积值。（想用两块最大height组成面积）

def maxArea_by_sort(height):
	area = 0
	 domain = [(height[i], i) for i in range(len(height))]
	 line = sorted(domain, key=lambda x: x[0], reverse=True)  
	 for i in range(len(line)):
	     for j in range(i + 1, len(line)):
	         area = max(area, line[j][0] * abs(line[j][1] - line[i][1]))
	 return area

思路二：双指针
双指针分别指向数组左右边界，需要确定指针如何移动：“短板效应”中，盛最多的水，取决于最小的那块板。so：

容积 = min( height [指针右]，height [指针左]） * （指针左 - 指针右）.

 area = min(height[r], height[l]) * (l - r)

每移动一步，左右距离必定减小：如果移动较长的那块板，最小长度不变，（l - r）必定会减小，所以area减小；移动较小的那块板，最小长度才可能增加，area才可能会增大。
Python：

def maxArea_double_pointer(self):
    height = self.height
    area = 0
    r, l = 0, len(height)-1
    while r < l:
        area = max(area,min(height[r], height[l]) * (l - r))
        if height[l] > height[r]:
            r += 1
        else:
            l -= 1
    return area

Go：

func maxArea(height []int)  int{
	maxarea := 0
	r,l := 0,len(height)-1

	for r < l{
		area := MinInt(height[r],height[l]) * (l - r)
		maxarea = MaxInt(area,maxarea)
		if height[r] < height[l]{
			r +=1
		}else {
			l -=1
		}
	}

	return maxarea
}

func MaxInt(a,b int) int {
	if a > b{
		return a
	}
	return b
}
func MinInt(a,b int) int {
	if a < b {
		return a
	}
	return b
}