【LeetCode】98. 验证二叉搜索树（中等）——代码随想录算法训练营Day20

题目链接：98. 验证二叉搜索树

题目描述

给你一个二叉树的根节点 root ，判断其是否是一个有效的二叉搜索树。

有效二叉搜索树定义如下：

节点的左子树只包含小于当前节点的数。
节点的右子树只包含大于当前节点的数。
所有左子树和右子树自身必须也是二叉搜索树。

示例 1：

输入：root = [2,1,3]
输出：true

示例 2：

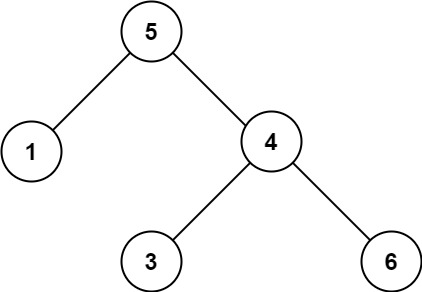
输入：root = [5,1,4,null,null,3,6]
输出：false
解释：根节点的值是 5 ，但是右子节点的值是 4 。

提示：

树中节点数目范围在[1, 104] 内
-231 <= Node.val <= 231 - 1

文章讲解：代码随想录

视频讲解：你对二叉搜索树了解的还不够！ | LeetCode：98.验证二叉搜索树_哔哩哔哩_bilibili

题解1：递归法

思路：二叉搜索树的中序遍历序列一定是一个递增序列，利用这个方法来判断是否是二叉搜索树。

/**
 * Definition for a binary tree node.
 * function TreeNode(val, left, right) {
 *     this.val = (val===undefined ? 0 : val)
 *     this.left = (left===undefined ? null : left)
 *     this.right = (right===undefined ? null : right)
 * }
 */
/**
 * @param {TreeNode} root
 * @return {boolean}
 */
var isValidBST = function(root) {
    let num = -Math.MAX_VALUE;
    const inorder = function (node) {
        if (!node) {
            return true;
        }
        const left = inorder(node.left); // 左
        // 中
        if (node.val <= num) {
            return false;
        }
        num = node.val;
        const right = inorder(node.right); // 右
        return left && right;
    }
    return inorder(root);
};

分析：时间复杂度为 O(n)，空间复杂度为 O(logn)。

题解2：递归法——双指针优化

思路：使用一个指针进行二叉树的中序遍历，另一个指针指向遍历的前一个元素，用双指针指向的元素进行比较。

/**
 * Definition for a binary tree node.
 * function TreeNode(val, left, right) {
 *     this.val = (val===undefined ? 0 : val)
 *     this.left = (left===undefined ? null : left)
 *     this.right = (right===undefined ? null : right)
 * }
 */
/**
 * @param {TreeNode} root
 * @return {boolean}
 */
var isValidBST = function(root) {
    let pre = null;
    const inorder = function (node) {
        if (!node) {
            return true;
        }
        const left = inorder(node.left); // 左
        // 中
        if (pre && node.val <= pre.val) {
            return false;
        }
        pre = node;
        const right = inorder(node.right); // 右
        return left && right;
    }
    return inorder(root);
};

分析：时间复杂度为 O(n)，空间复杂度为 O(logn)。

题解3：迭代法

思路：使用中序遍历的迭代法解此题。

/**
 * Definition for a binary tree node.
 * function TreeNode(val, left, right) {
 *     this.val = (val===undefined ? 0 : val)
 *     this.left = (left===undefined ? null : left)
 *     this.right = (right===undefined ? null : right)
 * }
 */
/**
 * @param {TreeNode} root
 * @return {boolean}
 */
var isValidBST = function(root) {
    let pre = null, cur = root;
    const stack = [];
    while (stack.length > 0 || cur) {
        if (cur) {
            stack.push(cur);
            cur = cur.left; // 左
        } else {
            cur = stack.pop();
            // 中
            if (pre && cur.val <= pre.val) {
                return false;
            } else {
                pre = cur;
            }
            cur = cur.right; // 右
        }
    }
    return true;
};

分析：时间复杂度为 O(n)，空间复杂度为 O(logn)。