约数里面的一个重要性质：一个数的约数都是成对存在的(以sqrt(x)为分界线)

---

一、求一个数的所有约数---试除法

int x; cin>>x;
int yue[10000]={0},idx=0;
for(int i=1;i<=x/i;i++)
{
    if(x%i==0)
    {
        yue[idx++]=i;
        cout<<i<<" ";
    }
}

for(int i=idx-1;i>=0;i--)
{
    if(x/yue[i]!=yue[i])cout<<x/yue[i]<<" ";
}

---

二、求一个数的约数的个数---(先对它分解质因数，然后套公式）

const int mod=1e9+7;
map<int,int>h;

int x; cin>>x;
for(int i=2;i<=x/i;i++)
{
    while(x%i==0)
    {
        x/=i;
        h[i]++;
    }
}
if(x>1)h[x]++;

long long ans=1;
for(auto i:h)
{
    ans=ans*(i.second+1)%mod;
}
cout<<ans;

---

三、求一个数的所有约数的和---(先对它分解质因数，然后套公式）

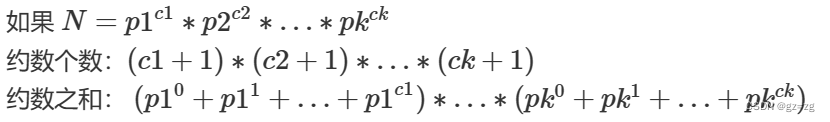
const int mod=1e9+7;
map<int,int>h;

int x; cin>>x;
for(int i=2;i<=x/i;i++)
{
    while(x%i==0)
    {
        x/=i;
        h[i]++;
    }
}
if(x>1)h[x]++;

long long ans=1;
for(auto i:h)
{
    int a=i.first;int b=i.second;
        
    long long temp=1;
    while(b--)temp=(temp*a+1)%mod;
       
    ans=ans*temp%mod;
}
cout<<ans;

---

四、求两个正整数的最大公约数---辗转相除法

int gcd(int a,int b)
{
    if(b==0)return a;
    else return gcd(b,a%b);
}

---