主要符号表
| LaTeX | 符号 | 说明 | How to read letter? |
|---|---|---|---|
| \mathit{x} | 标量 | ||
| \boldsymbol{x} | 向量 | ||
| \mathrm{x} | 变量集 | ||
| \mathbf{A} | 矩阵 | ||
| \mathbf{I} | 单位阵 | ||
| \mathcal{X} | 样本空间或状态空间 | calligraphic X | |
| \mathcal{D} | 概率分布 | Ɗ calligraphic D | |
| \mathit{H} | 数据样本(数据集) | ||
| \mathcal{H} | 假设空间 | calligraphic H | |
| \mathit{H} | 假设集 | ||
| \mathfrak{L} | 学习算法 | 𝕷 is Gothic L ; or frak L fraktur L | |
| \left ( \cdot ,\cdot ,\cdot \right ) | 行向量 | ||
| \left ( \cdot ;\cdot ;\cdot \right ) | 列向量 | ||
| \left ( \cdot \right )^{T} | 向量或矩阵转置 | ||
| \left \{ \cdot \cdot \cdot \right \} | 集合 | ||
| \left | \left \{ \cdot \cdot \cdot \right \} \right | | 集合 | ||
| \left \| \cdot \right \|_{p} | |||
| \mathit{P}\left ( \cdot \right ),\mathit{P}\left ( \cdot |\cdot \right ) | 概率质量函数,条件概率质量函数 | ||
| \mathit{p}\left ( \cdot \right ),\mathit{p}\left ( \cdot | \cdot \right ) | 概率密度函数,条件概率密度函数 | ||
| \mathbb{E}_{\cdot \sim \mathcal{D}}\left [ f\! \left ( \cdot \right ) \right ] | 函数 | ||
| sup\left ( \cdot \right ) | 上确界 | Supremum | |
| \mathbb{I}\! \left ( \cdot \right ) | 指示函数,在 | ||
| sign\left ( \cdot \right ) | 符号函数,在 |
How to read?
𝔸 is blackboard A; 𝔄 is Gothic A.
If there is only one 𝐴 being used, though, we read it as A regardless to the font.
This is a soft question. We can read the letters 𝐀, 𝐁, etc. as bold A, bold B, etc. We can read the letters AA, BB, etc. as italic A, italic B, etc. We can read the letters ,
, etc. as calligraphic A, calligraphic B, etc. But how do we read letters such as 𝔸, 𝔹, etc., or 𝔄, 𝔅, etc.?
I think it is just acceptable to say "A" and "B"...
It is quite rare that you have to read out aloud any maths that has the same letter appearing in two different fonts like this.
-
12"Small pee is an element of pee which is a subset of the powerset of A"?– Willie WongJan 22, 2013 at 13:54
-
2If I were teaching a class, I'd read that as "let p be a subset of A chosen in the family big p" (or something similar according to context)– Andrea MoriJan 22, 2013 at 14:35
-
Yes but how often does such a sentence come up in everyday maths? Also usually there is a bit of context which allows you to get round having to read it exactly as it is written...for example "our subset 𝑝 of 𝐴"– frettyJan 24, 2013 at 9:59
参考:
✍ Calligraphy 𝓉̲ℯ̲𝓍̲𝓉̲ font generator - 𝙁𝙎𝙮𝙢𝙗𝙤𝙡𝙨 (fsymbols.com)
上确界_百度百科 (baidu.com)
Text, Fonts & Gothic symbols 𝖈𝖔𝖕𝖞 𝖆𝖓𝖉 𝖕𝖆𝖘𝖙𝖊 🕱 (letraspro.com)soft question - How to read letters such as $\mathbb A$, $\mathbb B$, etc., or $\mathfrak A$, $\mathfrak B$, etc.? - Mathematics Stack Exchange

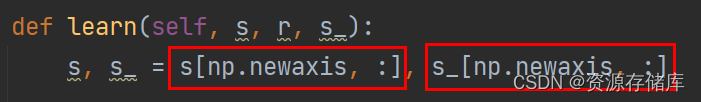







\mathbb,\mathcalor\mathfrak.Jan 23, 2013 at 0:37