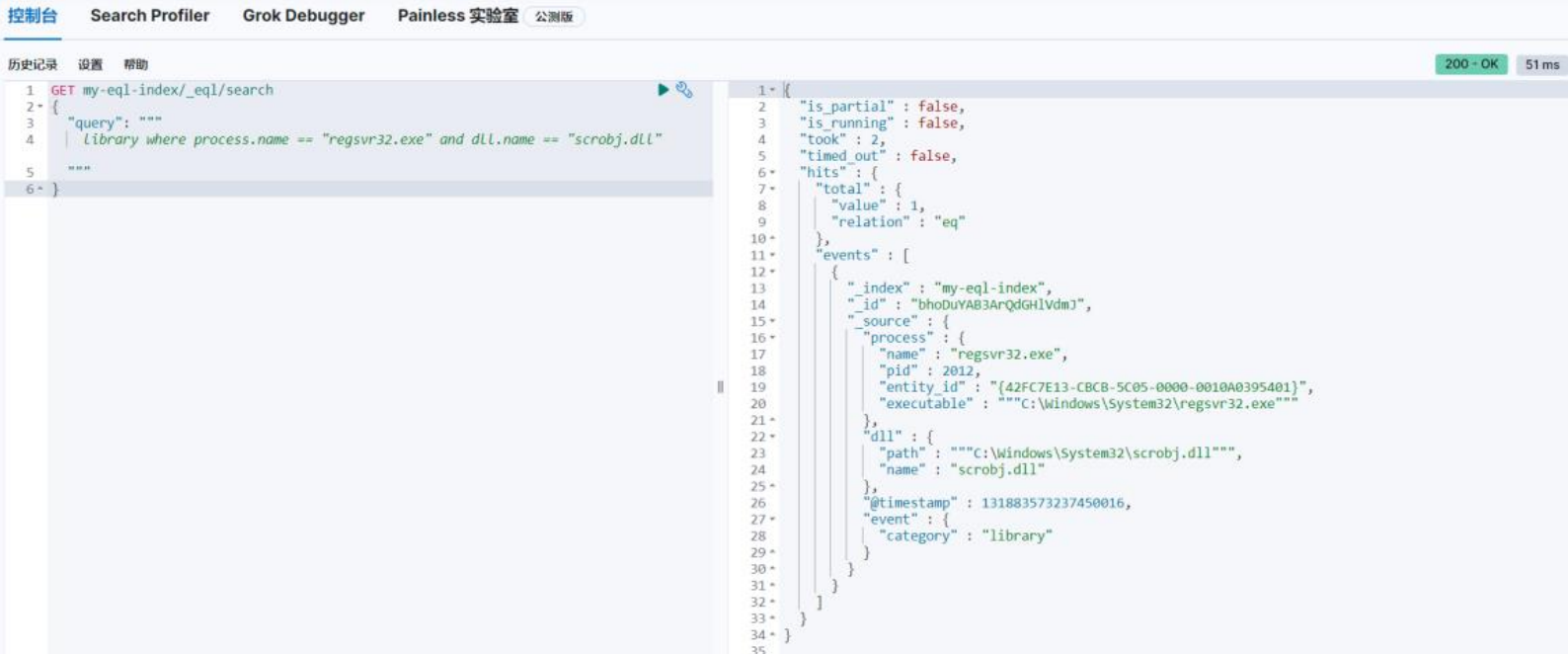
解法一:
求出
f
(
x
)
,
进而对
f
(
x
)
进行积分。
求出f(x),进而对f(x)进行积分。
求出f(x),进而对f(x)进行积分。
令
ln
x
=
t
,
原式
f
(
t
)
=
ln
(
1
+
e
t
)
e
t
令\ln x=t,原式f(t)=\frac{\ln (1+e^t)}{e^t}
令lnx=t,原式f(t)=etln(1+et)
则
∫
f
(
x
)
d
x
=
∫
ln
(
1
+
e
x
)
e
x
d
x
=
∫
ln
(
1
+
e
x
)
e
−
x
d
x
则\int f(x)\,{\rm d}x=\int\frac{\ln(1+e^x)}{e^x}\,{\rm d}x\\=\int \ln (1+e^x)e^{-x}\,{\rm d}x
则∫f(x)dx=∫exln(1+ex)dx=∫ln(1+ex)e−xdx
=
−
∫
ln
(
1
+
e
x
)
d
e
−
x
=-\int\ln(1+e^x)\,{\rm d}{e^{-x}}
=−∫ln(1+ex)de−x
=
−
ln
(
1
+
e
x
)
e
−
x
+
∫
e
−
x
d
ln
(
1
+
e
x
)
=-\ln(1+e^x)e^{-x}+\int e^{-x}\,{\rm d}{\ln (1+e^x)}
=−ln(1+ex)e−x+∫e−xdln(1+ex)
=
−
ln
(
1
+
e
x
)
e
−
x
+
∫
e
−
x
1
1
+
e
x
×
e
x
d
x
=-\ln(1+e^x)e^{-x}+\int e^{-x}\frac{1}{1+e^x}\times e^x\,{\rm d}x
=−ln(1+ex)e−x+∫e−x1+ex1×exdx
=
−
ln
(
1
+
e
x
)
e
−
x
+
∫
1
1
+
e
x
d
x
=-\ln(1+e^x)e^{-x}+\int\frac{1}{1+e^x}\,{\rm d}x
=−ln(1+ex)e−x+∫1+ex1dx
=
−
ln
(
1
+
e
x
)
e
−
x
+
x
−
ln
(
e
x
+
1
)
+
C
=-\ln(1+e^x)e^{-x}+x-\ln(e^x+1)+C
=−ln(1+ex)e−x+x−ln(ex+1)+C
计算
∫
1
1
+
e
x
d
x
:
计算\int\frac{1}{1+e^x}\,{\rm d}x:
计算∫1+ex1dx:
原式
=
∫
(
1
+
e
x
)
−
e
x
1
+
e
x
d
x
原式=\int \frac{(1+e^x)-e^x}{1+e^x}\,{\rm d}x
原式=∫1+ex(1+ex)−exdx
=
∫
(
1
−
e
x
1
+
e
x
)
d
x
=\int (1-\frac{e^x}{1+e^x})\,{\rm d}x
=∫(1−1+exex)dx
=
x
−
∫
d
(
e
x
+
1
)
1
+
e
x
=x-\int\frac{{\rm d}{(e^x+1)}}{1+e^x}
=x−∫1+exd(ex+1)
=
x
−
ln
(
e
x
+
1
)
+
C
=x-\ln(e^x+1)+C
=x−ln(ex+1)+C
解法二:
令
t
=
ln
x
(
x
=
e
t
)
∫
f
(
ln
x
)
d
ln
x
令t=\ln x(x=e^t)\int f(\ln x)\,{\rm d}{\ln x}
令t=lnx(x=et)∫f(lnx)dlnx
=
∫
ln
(
1
+
x
)
x
×
1
x
d
x
=\int \frac{\ln (1+x)}{x}\times \frac{1}{x}\,{\rm d}x
=∫xln(1+x)×x1dx
=
∫
ln
(
1
+
x
)
x
2
d
x
=\int \frac{\ln (1+x)}{x^2}\,{\rm d}x
=∫x2ln(1+x)dx
=
−
∫
ln
(
1
+
x
)
d
1
x
=-\int \ln(1+x)\,{\rm d}{\frac{1}{x}}
=−∫ln(1+x)dx1
=
−
ln
(
1
+
x
)
x
+
∫
1
x
×
1
x
+
1
d
x
=-\frac{\ln (1+x)}{x}+\int\frac{1}{x}\times\frac{1}{x+1}\,{\rm d}x
=−xln(1+x)+∫x1×x+11dx
=
−
ln
(
1
+
x
)
x
+
ln
∣
x
x
+
1
∣
+
C
=-\frac{\ln (1+x)}{x}+\ln \lvert\frac{x}{x+1} \rvert+C
=−xln(1+x)+ln∣x+1x∣+C
=
−
ln
(
1
+
e
t
)
e
t
+
ln
∣
e
t
e
t
+
1
∣
+
C
=-\frac{\ln(1+e^t)}{e^t}+\ln \lvert \frac{e^t}{e^t+1}\rvert+C
=−etln(1+et)+ln∣et+1et∣+C
由于积分变量为x,则所求为
−
ln
(
1
+
e
x
)
e
x
+
ln
∣
e
x
e
x
+
1
∣
+
C
-\frac{\ln(1+e^x)}{e^x}+\ln \lvert \frac{e^x}{e^x+1}\rvert+C
−exln(1+ex)+ln∣ex+1ex∣+C