1007、1009：与进制问题、输出问题

1007

题目：本题要求计算A/B，其中A是不超过1000位的正整数，B是1位正整数。你需要输出商数Q和余数R，使得A = B * Q + R成立

思路：对于数字元素拆分，除法的计算方法用代码实现（唯一一点就是在输出的时候首元素按照输出的格式是不能有0的情况存在）

以下是我初次通过本地测试成功的代码，但是在上传到牛客的时候出现，实际结果没有输出q数组，我就很奇怪，我使用了相同测试用例到本地没有问题，但是在网上就是通过不了。

#include<iostream>
#include<cstdio>
#include<string>
using namespace std;

int main() {
	string str;
	int n,len;
	freopen("i.txt","r",stdin);
	char q[1001];
	cin>>str>>n;
	int mode=0;
	for(int i=0; i<str.length(); i++) {
		mode=mode*10+((int)str[i]-'0');
		q[i]=(char)mode/n+'0';
		mode%=n;
		len++;
	}
	for(int i=0; i<len; i++) {
		if(i==0&&q[i]=='0') {
				continue;
			}
		printf("%c",q[i]);
	}
	printf(" %d",mode);

	return 0;
}

参考代码：

#include<iostream>
#include<string>
using namespace std;
int main(){
    string a;
    int b;
    cin>>a>>b;
    int yu = a[0] - '0';
    for(int i = 1;i<a.size();i++){
        int tem = yu*10+ (a[i] - '0');
        cout<<tem/b;//输出结果
        yu = tem %b;
    }
    cout<<" "<<yu;//输出结果
    return 0;
}

然后就学会了：以后在获取到结果的时候，能通过使用for循环输出结果。这样就又省了一次for循环，减少代码运行的时间

改进代码：（以下代码就能顺利通过测试用例）

#include<iostream>
#include<cstdio>
#include<string>
using namespace std;

int main() {
	string str;
	int n,len;
	// freopen("i.txt","r",stdin);
	char q[1001];
	cin>>str>>n;
	int mode=0;
	for(int i=0; i<str.length(); i++) {
		mode=mode*10+((int)str[i]-'0');
		q[i]=(char)mode/n+'0';
		mode%=n;
		
		if(i==0&&q[i]=='0') {
			continue;
		}
		printf("%c",q[i]);
	}
	printf(" %d",mode);

	return 0;
}

总结

1.使用for循环顺手输出结果

2.字符转数字是使用了字符数字与字符数字‘0’的差值获取了整型数字

3.使用取余数的方式可以获取一串数字里面的元素。

1009

简单，但是麻烦

题目：

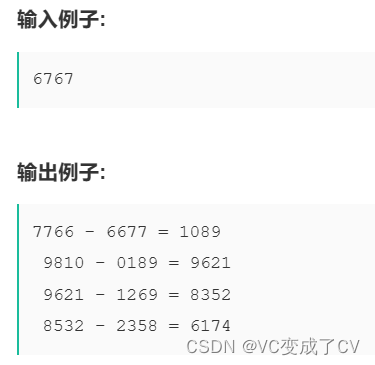
给定任一个各位数字不完全相同的4位正整数，如果我们先把4个数字按非递增排序，再按非递减排序，然后用第1个数字减第2个数字，将得到
 一个新的数字。一直重复这样做，我们很快会停在有“数字黑洞”之称的6174，这个神奇的数字也叫Kaprekar常数。
 
 例如，我们从6767开始，将得到
 
 7766 - 6677 = 1089
 9810 - 0189 = 9621
 9621 - 1269 = 8352
 8532 - 2358 = 6174
 7641 - 1467 = 6174
 ... ...
 
 现给定任意4位正整数，请编写程序演示到达黑洞的过程。

#include<iostream>
#include<cstdio>
#include<algorithm>

using namespace std;
bool cmp(int a,int b) {
	return a>b;
}

int main() {
	// freopen("in.txt","r",stdin);
	int num;
	cin>>num;
	do{
		int n[4];
		for(int i=3; i>=0; i--) {
			n[i]=num%10;
			num=num/10;
		}
		sort(n,n+4,cmp);
		printf("%d%d%d%d - %d%d%d%d = ",n[0],n[1],n[2],n[3],n[3],n[2],n[1],n[0]);
		num=(n[0]*1000+n[1]*100+n[2]*10+n[3])-(n[3]*1000+n[2]*100+n[1]*10+n[0]);
		printf("%d\n",num);	
	}while(num!=6174);
	return 0;
}