JAVA代码编写
104. 二叉树的最大深度
给定一个二叉树 root ,返回其最大深度。
二叉树的 最大深度 是指从根节点到最远叶子节点的最长路径上的节点数。
示例 1:

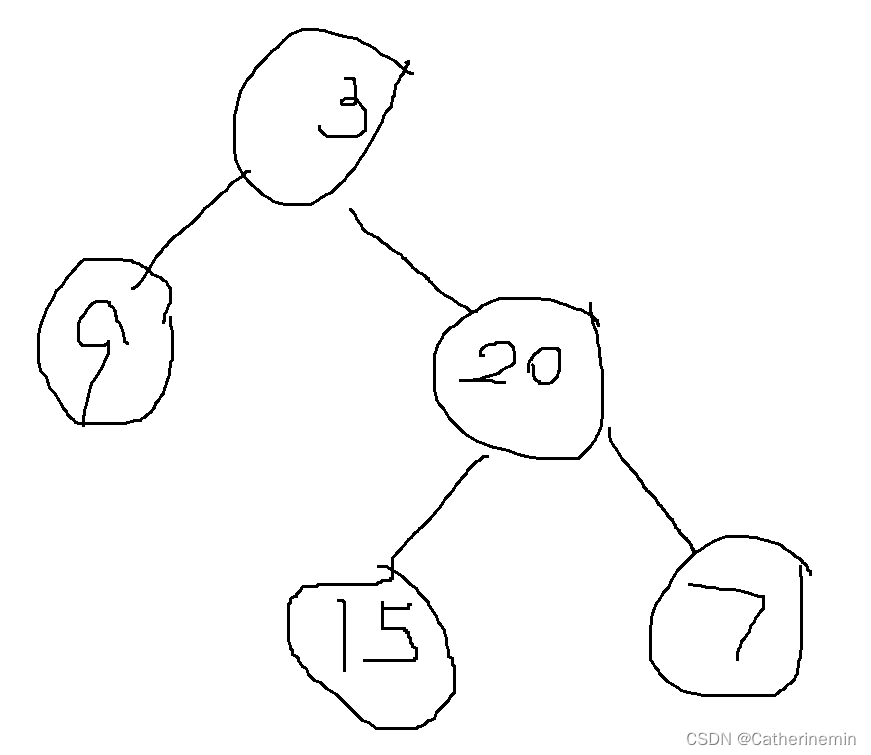
输入:root = [3,9,20,null,null,15,7]
输出:3
示例 2:
输入:root = [1,null,2]
输出:2
提示:
- 树中节点的数量在
[0, 104]区间内。 -100 <= Node.val <= 100
教程:https://programmercarl.com/0104.%E4%BA%8C%E5%8F%89%E6%A0%91%E7%9A%84%E6%9C%80%E5%A4%A7%E6%B7%B1%E5%BA%A6.html#%E5%85%B6%E4%BB%96%E8%AF%AD%E8%A8%80%E7%89%88%E6%9C%AC
视频:https://www.bilibili.com/video/BV1Gd4y1V75u/
方法一:递归
思路:
复杂度分析:
-
时间复杂度: O ( n ) O(n) O(n)
-
空间复杂度: O ( n ) O(n) O(n)
class TreeNode {
int val;
TreeNode left;
TreeNode right;
TreeNode() {}
TreeNode(int val) { this.val = val; }
TreeNode(int val, TreeNode left, TreeNode right) {
this.val = val;
this.left = left;
this.right = right;
}
}
public class Solution {
public int maxDepth(TreeNode root) {
if(root==null) return 0;
int leftDepth = maxDepth(root.left);
int rightDepth = maxDepth(root.right);
return Math.max(leftDepth, rightDepth) + 1;
}
public static void main(String[] args) {
// 创建一棵二叉树
TreeNode root = new TreeNode(3);
root.left = new TreeNode(9);
root.right = new TreeNode(20, new TreeNode(15), new TreeNode(7));
// 调用 maxDepth 方法计算二叉树的最大深度
Solution solution = new Solution();
int depth = solution.maxDepth(root);
System.out.println("The maximum depth of the binary tree is: " + depth);
}
}
111. 二叉树的最小深度
给定一个二叉树,找出其最小深度。
最小深度是从根节点到最近叶子节点的最短路径上的节点数量。
**说明:**叶子节点是指没有子节点的节点。
示例 1:
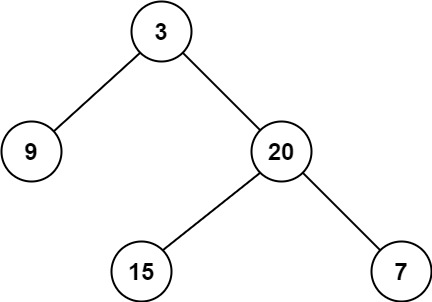
输入:root = [3,9,20,null,null,15,7]
输出:2
示例 2:
输入:root = [2,null,3,null,4,null,5,null,6]
输出:5
提示:
- 树中节点数的范围在
[0, 105]内 -1000 <= Node.val <= 1000
教程:https://programmercarl.com/0111.%E4%BA%8C%E5%8F%89%E6%A0%91%E7%9A%84%E6%9C%80%E5%B0%8F%E6%B7%B1%E5%BA%A6.html
视频:https://www.bilibili.com/video/BV1QD4y1B7e2/
方法一:递归
思路:
复杂度分析:
-
时间复杂度: O(n),其中 n 是树中节点的个数。
-
空间复杂度: O(h),其中 h 是树的高度。
class Solution {
public int minDepth(TreeNode root) {
if(root ==null) return 0;
int leftDepth = minDepth(root.left);
int rightDepth = minDepth(root.right);
if(root.left == null){
return rightDepth+1;
}
if(root.right == null){
return leftDepth+1;
}
//左右结点都不为null
return Math.min(leftDepth,rightDepth)+1;
}
}
222. 完全二叉树的节点个数
给你一棵 完全二叉树 的根节点 root ,求出该树的节点个数。
完全二叉树 的定义如下:在完全二叉树中,除了最底层节点可能没填满外,其余每层节点数都达到最大值,并且最下面一层的节点都集中在该层最左边的若干位置。若最底层为第 h 层,则该层包含 1~ 2h 个节点。
示例 1:
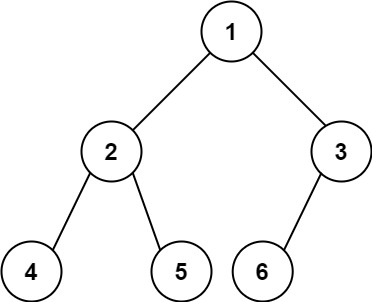
输入:root = [1,2,3,4,5,6]
输出:6
示例 2:
输入:root = []
输出:0
示例 3:
输入:root = [1]
输出:1
提示:
- 树中节点的数目范围是
[0, 5 * 104] 0 <= Node.val <= 5 * 104- 题目数据保证输入的树是 完全二叉树
**进阶:**遍历树来统计节点是一种时间复杂度为 O(n) 的简单解决方案。你可以设计一个更快的算法吗?
教程:https://programmercarl.com/0222.%E5%AE%8C%E5%85%A8%E4%BA%8C%E5%8F%89%E6%A0%91%E7%9A%84%E8%8A%82%E7%82%B9%E4%B8%AA%E6%95%B0.html
视频:https://www.bilibili.com/video/BV1eW4y1B7pD/
方法一:递归
思路:
复杂度分析:
- 时间复杂度: O ( n ) O(n) O(n)
- 空间复杂度: O ( n ) O(n) O(n)
class TreeNode {
int val;
TreeNode left;
TreeNode right;
TreeNode() {}
TreeNode(int val) { this.val = val; }
TreeNode(int val, TreeNode left, TreeNode right) {
this.val = val;
this.left = left;
this.right = right;
}
}
class Solution {
public int countNodes(TreeNode root) {
if(root==null) return 0;
int leftNodes= countNodes(root.left);
int rightNodes = countNodes(root.right);
return leftNodes+rightNodes+1;
}
}