目录
LeetCode之路——111. 二叉树的最小深度
分析
解法一:广度优先查询
解法二:深度优先查询

LeetCode之路——111. 二叉树的最小深度
给定一个二叉树,找出其最小深度。
最小深度是从根节点到最近叶子节点的最短路径上的节点数量。
说明:叶子节点是指没有子节点的节点。
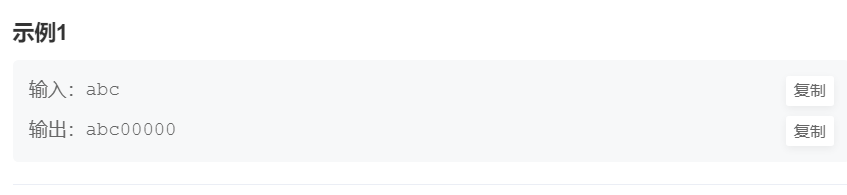
示例 1:
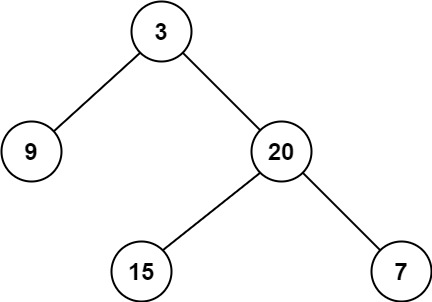
输入:root = [3,9,20,null,null,15,7] 输出:2
示例 2:
输入:root = [2,null,3,null,4,null,5,null,6] 输出:5
提示:
-
树中节点数的范围在
[0, 105]内 -
-1000 <= Node.val <= 1000
分析
还是可以使用层序遍历的思路来处理,最小深度的节点的条件:左右孩子都为空。
解法一:广度优先查询
class Solution {
public int minDepth(TreeNode root){
if (root == null) {
return 0;
}
Queue<TreeNode> queue = new LinkedList<>();
queue.offer(root);
int deep = 0;
while (!queue.isEmpty()){
int size = queue.size();
deep++;
TreeNode cur = null;
for (int i = 0; i < size; i++) {
cur = queue.poll();
//如果当前节点的左右孩子都为空,直接返回最小深度
if (cur.left == null && cur.right == null){
return deep;
}
if (cur.left != null) queue.offer(cur.left);
if (cur.right != null) queue.offer(cur.right);
}
}
return deep;
}
}
-
时间复杂度:O(n)
-
空间复杂度:O(n)
解法二:深度优先查询
官网题解:
class Solution {
public int minDepth(TreeNode root) {
if (root == null) {
return 0;
}
if (root.left == null && root.right == null) {
return 1;
}
int min_depth = Integer.MAX_VALUE;
if (root.left != null) {
min_depth = Math.min(minDepth(root.left), min_depth);
}
if (root.right != null) {
min_depth = Math.min(minDepth(root.right), min_depth);
}
return min_depth + 1;
}
}
作者:力扣官方题解
链接:https://leetcode.cn/problems/minimum-depth-of-binary-tree/solutions/382646/er-cha-shu-de-zui-xiao-shen-du-by-leetcode-solutio/
来源:力扣(LeetCode)
-
时间复杂度:O(N),其中 N是树的节点数。对每个节点访问一次。
-
空间复杂度:O(H),其中 H 是树的高度。空间复杂度主要取决于递归时栈空间的开销,最坏情况下,树呈现链状,空间复杂度为 O(N)。平均情况下树的高度与节点数的对数正相关,空间复杂度为O(logN)。

![[Python进阶] 消息框、弹窗:ctypes](https://img-blog.csdnimg.cn/4c1a986e92c041ca82c64c2c8f403996.png)