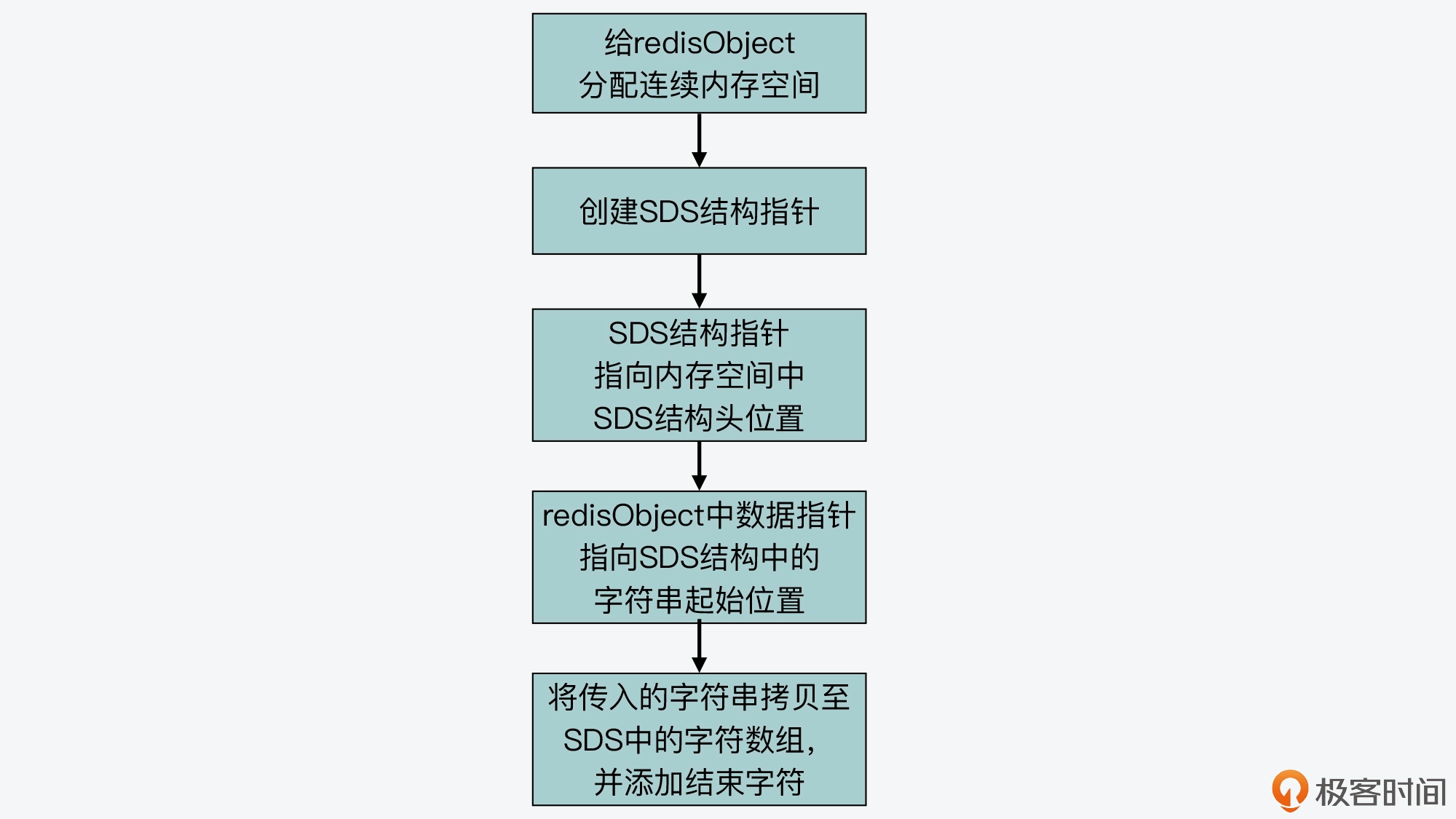
求三维坐标系的旋转矩阵通常需要求分别沿3个坐标轴的二维坐标系下的旋转矩阵,二维坐标系下的旋转矩阵的推导过程通常以某一点逆时针旋转 θ \theta θ角度进行推理。以下将通过此例来详细讲解二维坐标系下的旋转矩阵推导过程,并进一步给出其他方式的旋转矩阵。
一、二维坐标中点的旋转变换
点的旋转矩阵(逆时针旋转)
已知点
P
(
x
,
y
)
P(x, y)
P(x,y),将该点以逆时针方向旋转
θ
\theta
θ角度后得到点
P
′
(
x
′
,
y
′
)
P^{\prime}(x^{\prime}, y^{\prime})
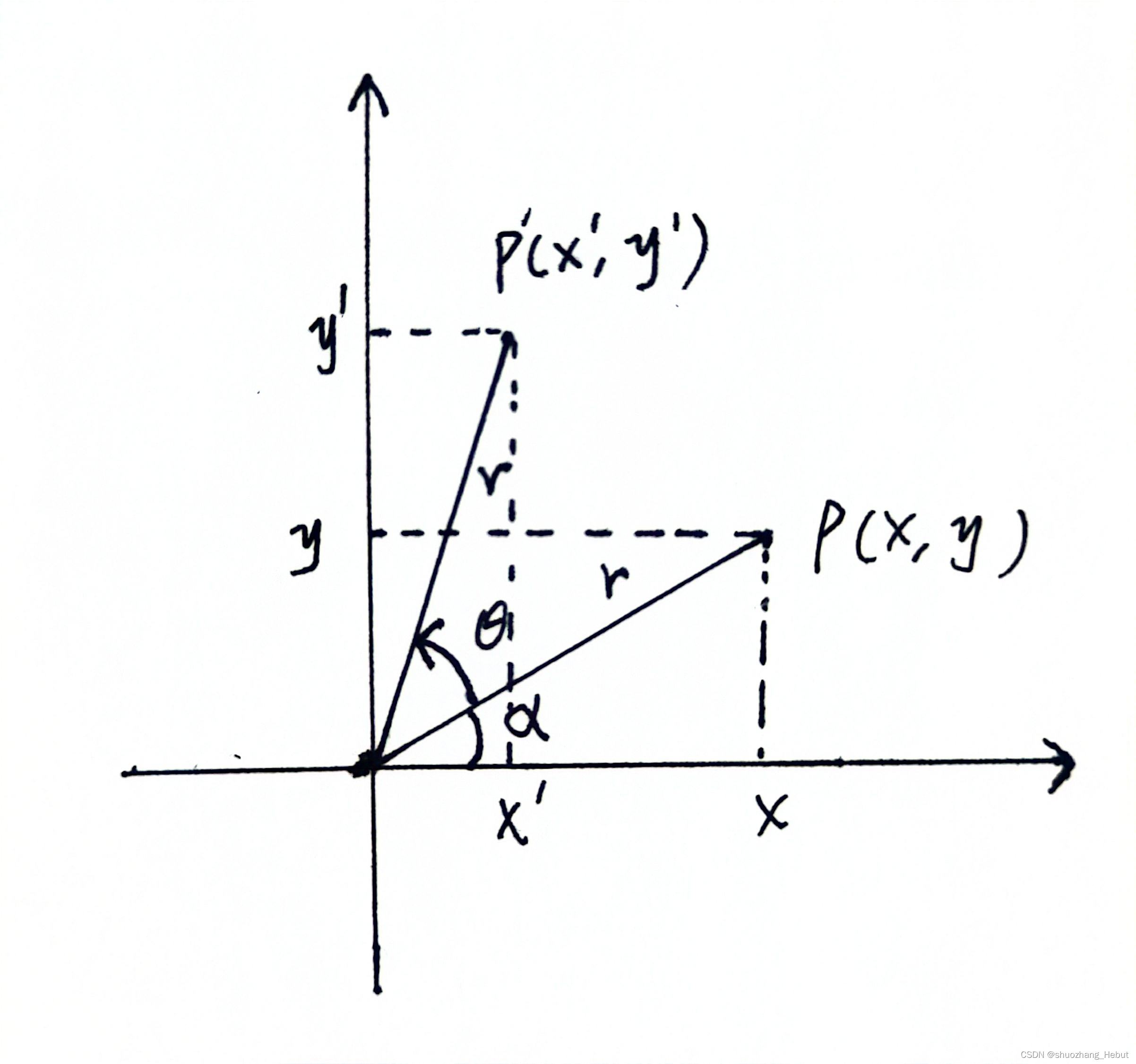
P′(x′,y′),如下图所示。求由点
P
P
P到点
P
′
P^{\prime}
P′的旋转矩阵。
-
设半径为 r r r,由图可以分别得到以下三角公式:
- 对于点
P
(
x
,
y
)
P(x, y)
P(x,y)
x = r cos α y = r sin α (1) \begin{aligned} & x=r \cos \alpha \\ & y=r \sin \alpha \end{aligned} \tag{1} x=rcosαy=rsinα(1) - 对于点
P
′
(
x
′
,
y
′
)
P^{\prime}(x^{\prime}, y^{\prime})
P′(x′,y′)
x ′ = r cos ( α + θ ) y ′ = r sin ( α + θ ) (2) \begin{aligned} & x^{\prime}=r \cos (\alpha+\theta) \\ & y^{\prime}=r \sin (\alpha+\theta) \end{aligned} \tag{2} x′=rcos(α+θ)y′=rsin(α+θ)(2)
- 对于点
P
(
x
,
y
)
P(x, y)
P(x,y)
-
根据两角和的正弦与余弦公式:
sin ( α + θ ) = sin α cos θ + cos α sin θ cos ( α + θ ) = cos α cos θ − sin α sin θ (3) \begin{aligned} & \sin (\alpha+\theta)=\sin \alpha \cos \theta+\cos \alpha \sin \theta \\ & \cos (\alpha+\theta)=\cos \alpha \cos \theta-\sin \alpha \sin \theta \end{aligned} \tag{3} sin(α+θ)=sinαcosθ+cosαsinθcos(α+θ)=cosαcosθ−sinαsinθ(3) -
将公式(3)代入公式(2),可得到:
x ′ = r cos α cos θ − r sin α sin θ y ′ = r sin α cos θ + r cos α sin θ (4) \begin{aligned} & x^{\prime} =r \cos \alpha \cos \theta-r \sin \alpha \sin \theta \\ & y^{\prime} =r \sin \alpha \cos \theta+r \cos \alpha \sin \theta \\ \end{aligned} \tag{4} x′=rcosαcosθ−rsinαsinθy′=rsinαcosθ+rcosαsinθ(4) -
将公式(1)代入公式(4),化简掉 α \alpha α,可得到 ( x ′ , y ′ ) (x^{\prime}, y^{\prime}) (x′,y′)与 ( x , y ) (x, y) (x,y)的关系,即:
x ′ = x cos θ − y sin θ y ′ = y cos θ + x sin θ (5) \begin{aligned} & x^{\prime} =x \cos \theta-y \sin \theta \\ & y^{\prime} =y \cos \theta+x \sin \theta \\ \end{aligned} \tag{5} x′=xcosθ−ysinθy′=ycosθ+xsinθ(5) -
整理公式(5)可得到旋转矩阵公式:
x ′ = cos θ ⋅ x − sin θ ⋅ y y ′ = sin θ ⋅ x + cos θ ⋅ y ⇒ [ x ′ y ′ ] = [ cos θ − sin θ sin θ cos θ ] [ x y ] (6) \begin{aligned} x^{\prime}&=\cos \theta \cdot x-\sin \theta \cdot y \\ y^{\prime}&=\sin \theta \cdot x+\cos \theta \cdot y \\ \Rightarrow \left[\begin{array}{l} x^{\prime} \\ y^{\prime}\end{array}\right] &=\left[\begin{array}{cc}\cos \theta & -\sin \theta \\ \sin \theta & \cos \theta \end{array}\right] \left[\begin{array}{l} x \\ y \end{array}\right] \end{aligned} \tag{6} x′y′⇒[x′y′]=cosθ⋅x−sinθ⋅y=sinθ⋅x+cosθ⋅y=[cosθsinθ−sinθcosθ][xy](6) -
因此二维坐标系下逆时针旋转矩阵 R R R为:
R = [ cos θ − sin θ sin θ cos θ ] R = \left[\begin{array}{cc}\cos \theta & -\sin \theta \\ \sin \theta & \cos \theta \end{array}\right] R=[cosθsinθ−sinθcosθ]
点的旋转矩阵(顺时针旋转)
已知点 P ′ ( x ′ , y ′ ) P^{\prime}(x^{\prime}, y^{\prime}) P′(x′,y′),将该点以顺时针方向旋转 θ \theta θ角度后得到点 P ( x , y ) P(x, y) P(x,y),求 P P P。
1、利用求逆矩阵的方式
- 二阶矩阵的逆的求法为:主对角元素换位置,次对角元素变符号,再乘上系数1/(ad-bc),由于旋转矩阵
R
R
R的ad-bc=1,因此
R
R
R的逆矩阵
R
′
R^{\prime}
R′为:
R ′ = [ cos θ sin θ − sin θ cos θ ] R^{\prime} = \left[\begin{array}{cc}\cos \theta & \sin \theta \\ -\sin \theta & \cos \theta \end{array}\right] R′=[cosθ−sinθsinθcosθ] - 因此旋转公式为:
[ x y ] = [ cos θ sin θ − sin θ cos θ ] [ x ′ y ′ ] \left[\begin{array}{l} x \\ y \end{array}\right] = \left[\begin{array}{cc}\cos \theta & \sin \theta \\ -\sin \theta & \cos \theta \end{array}\right] \left[\begin{array}{l} x^{\prime} \\ y^{\prime}\end{array}\right] [xy]=[cosθ−sinθsinθcosθ][x′y′]
2、利用几何知识推导
将 P ′ P^{\prime} P′顺时针旋转 θ \theta θ角度到 P P P,相当于逆时针旋转 ( 2 π − θ ) (2 \pi-\theta) (2π−θ),由于三角函数的周期为 2 π 2 \pi 2π,因此相当于旋转 − θ -\theta −θ角度。
- 将
−
θ
-\theta
−θ代入到公式(6),得到:
x = cos ( − θ ) ⋅ x ′ − sin ( − θ ) ⋅ y ′ = cos θ ⋅ x ′ + sin θ ⋅ y ′ y = sin ( − θ ) ⋅ x ′ + cos ( − θ ) ⋅ y ′ = − sin θ ⋅ x ′ + cos θ ⋅ y ′ \begin{aligned} x & =\cos (-\theta) \cdot x^{\prime}-\sin (-\theta) \cdot y^{\prime} \\ & =\cos \theta \cdot x^{\prime}+\sin \theta \cdot y^{\prime} \\ y & =\sin (-\theta) \cdot x^{\prime}+\cos (-\theta) \cdot y^{\prime} \\ & =-\sin \theta \cdot x^{\prime}+\cos \theta \cdot y^{\prime} \end{aligned} xy=cos(−θ)⋅x′−sin(−θ)⋅y′=cosθ⋅x′+sinθ⋅y′=sin(−θ)⋅x′+cos(−θ)⋅y′=−sinθ⋅x′+cosθ⋅y′ - 整理上述公式可得到顺时针时的旋转矩阵公式:
x = cos θ ⋅ x ′ + sin θ ⋅ y ′ y = − sin θ ⋅ x ′ + cos θ ⋅ y ′ ⇒ [ x y ] = [ cos θ sin θ − sin θ cos θ ] [ x ′ y ′ ] \begin{aligned} x&=\cos \theta \cdot x^{\prime}+\sin \theta \cdot y^{\prime} \\ y&=-\sin \theta \cdot x^{\prime}+\cos \theta \cdot y^{\prime} \\ \Rightarrow \left[\begin{array}{l} x \\ y \end{array}\right] &= \left[\begin{array}{cc}\cos \theta & \sin \theta \\ -\sin \theta & \cos \theta \end{array}\right] \left[\begin{array}{l} x^{\prime} \\ y^{\prime}\end{array}\right] \end{aligned} xy⇒[xy]=cosθ⋅x′+sinθ⋅y′=−sinθ⋅x′+cosθ⋅y′=[cosθ−sinθsinθcosθ][x′y′]
二、二维坐标系的旋转变换
二维坐标系 XOY \text{XOY} XOY中有一点 P ( x , y ) P(x, y) P(x,y),将坐标系 XOY \text{XOY} XOY逆时针旋转 θ \theta θ角度后得到新坐标系 X ′ O ′ Y ′ \text{X}^{\prime}\text{O}^{\prime}\text{Y}^{\prime} X′O′Y′,求点 P P P在新坐标系下的坐标 P ′ ( x ′ , y ′ ) P^{\prime}(x^{\prime}, y^{\prime}) P′(x′,y′),这个过程称为坐标系变换。
新坐标的求法可以转换为逆向旋转坐标点的方式,并利用点的旋转变换求得。因此,坐标系顺时针旋转 θ \theta θ角度,相当于原坐标系中某一点逆时针旋转 θ \theta θ角度,反之亦然。
总结
- 某点逆时针旋转
θ
\theta
θ角度(坐标系顺时针旋转
θ
\theta
θ角度)的旋转矩阵为:
R = [ cos θ − sin θ sin θ cos θ ] R = \left[\begin{array}{cc}\cos \theta & -\sin \theta \\ \sin \theta & \cos \theta \end{array}\right] R=[cosθsinθ−sinθcosθ] - 某点顺时针旋转
θ
\theta
θ角度(坐标系逆时针旋转
θ
\theta
θ角度)的旋转矩阵为:
R ′ = [ cos θ sin θ − sin θ cos θ ] R^{\prime} = \left[\begin{array}{cc}\cos \theta & \sin \theta \\ -\sin \theta & \cos \theta \end{array}\right] R′=[cosθ−sinθsinθcosθ]
三维坐标变换可进一步参考Link3。
参考:
Link1
Link2
Link3